Digitale Werkzeuge in der Schule/Unterwegs in 3-D – Punkte, Vektoren, Geraden und Ebenen im Raum/Lagebeziehungen und Winkel (Gerade und Ebene, 2 Ebenen): Unterschied zwischen den Versionen
Aus ZUM Projektwiki
< Digitale Werkzeuge in der Schule | Unterwegs in 3-D – Punkte, Vektoren, Geraden und Ebenen im Raum
Keine Bearbeitungszusammenfassung |
Keine Bearbeitungszusammenfassung Markierung: 2017-Quelltext-Bearbeitung |
||
| Zeile 480: | Zeile 480: | ||
'''2. Schritt:''' Einsetzen der Normalenvektoren in die Formel. | '''2. Schritt:''' Einsetzen der Normalenvektoren in die Formel. | ||
<math>cos(\alpha) = \frac{ \begin{pmatrix} 2 \\ -2 \\ -1 \end{pmatrix} \ast \begin{pmatrix} 7 \\ 1 \\ -3 \end{pmatrix}}{\begin{pmatrix} 2 \\ -2 \\ -1 \end{pmatrix} \cdot \begin{pmatrix} 7 \\ 1 \\ -3 \end{pmatrix}} \Leftrightarrow cos(\alpha) = \frac{16}{\sqrt{9} \cdot \sqrt{59}} \Leftrightarrow cos(\alpha) = \frac{16}{3 \cdot \sqrt{59}}</math> | <math>cos(\alpha) = \frac{ \left| \begin{pmatrix} 2 \\ -2 \\ -1 \end{pmatrix} \ast \begin{pmatrix} 7 \\ 1 \\ -3 \end{pmatrix} \right|}{\left| \begin{pmatrix} 2 \\ -2 \\ -1 \end{pmatrix} \right| \cdot \ left| \begin{pmatrix} 7 \\ 1 \\ -3 \end{pmatrix} \right| } \Leftrightarrow cos(\alpha) = \frac{16}{\sqrt{9} \cdot \sqrt{59}} \Leftrightarrow cos(\alpha) = \frac{16}{3 \cdot \sqrt{59}}</math> | ||
'''3. Schritt:''' Auflösen der Gleichung. | '''3. Schritt:''' Auflösen der Gleichung. | ||
| Zeile 487: | Zeile 487: | ||
{{Box | Aufgabe <Nummer>: | {{Box | Aufgabe <Nummer>: Schnittwinkel zwischen Ebenen | | ||
Sei <math>E</math> eine Ebene mit <math>E: \vec{x} = \begin{pmatrix} 0 \\ 0 \\ 0 \end{pmatrix} + r \cdot \begin{pmatrix} 1 \\ 0 \\ 0 \end{pmatrix}+ s \cdot \begin{pmatrix} 0 \\ 1 \\ 0\end{pmatrix}, r,s \in \mathbb{R}</math> , | Sei <math>E</math> eine Ebene mit <math>E: \vec{x} = \begin{pmatrix} 0 \\ 0 \\ 0 \end{pmatrix} + r \cdot \begin{pmatrix} 1 \\ 0 \\ 0 \end{pmatrix}+ s \cdot \begin{pmatrix} 0 \\ 1 \\ 0\end{pmatrix}, r,s \in \mathbb{R}</math> , | ||
| Zeile 500: | Zeile 500: | ||
{{Lösung versteckt|1= Bei der Ebene <math>E</math> handelt es sich um die <math>x_1-x_2-</math> Ebene. Der Normalenvektor ist also <math>\vec{n} = | {{Lösung versteckt|1= Bei der Ebene <math>E</math> handelt es sich um die <math>x_1-x_2-</math> Ebene. Der Normalenvektor ist also <math>\vec{n} = \begin{pmatrix} 0 \\ 0 \\ 1 \end{pmatrix} </math>. Der Normalenvektor der Ebene <math>F</math> kann abgelesen werden: <math>\vec{m} = \begin{pmatrix} 2 \\ 6 \\ 4 \end{pmatrix} </math>. | ||
Einsetzen in die Formel liefert: | Einsetzen in die Formel liefert: | ||
<math>cos(\alpha) = \frac{ \begin{pmatrix} 0 \\ 0 \\ 1 \end{pmatrix} \ast \begin{pmatrix} 2 \\ 6 \\ 4 \end{pmatrix}}{\begin{pmatrix} 0 \\ 0 \\ 1 \end{pmatrix} \cdot \begin{pmatrix} 2 \\ 6 \\ 4 \end{pmatrix}} \Leftrightarrow cos(\alpha) = \frac{4}{\sqrt{1} \cdot \sqrt{4+36+16}} \Leftrightarrow cos(\alpha) = \frac{4}{\sqrt{56}}</math> | <math>cos(\alpha) = \frac{ \left| \begin{pmatrix} 0 \\ 0 \\ 1 \end{pmatrix} \ast \begin{pmatrix} 2 \\ 6 \\ 4 \end{pmatrix} \right|}{\left| \begin{pmatrix} 0 \\ 0 \\ 1 \end{pmatrix} \right| \cdot \left| \begin{pmatrix} 2 \\ 6 \\ 4 \end{pmatrix} \right|} \Leftrightarrow cos(\alpha) = \frac{|4|}{\sqrt{1} \cdot \sqrt{4+36+16}} \Leftrightarrow cos(\alpha) = \frac{4}{\sqrt{56}}</math> | ||
Nun muss die Formel mit Hilfe des Taschenrechners aufgelöst werden: | Nun muss die Formel mit Hilfe des Taschenrechners aufgelöst werden: | ||
| Zeile 518: | Zeile 518: | ||
Einsetzen in die Formel liefert: | Einsetzen in die Formel liefert: | ||
<math>cos(\alpha) = \frac{ \begin{pmatrix} 2 \\ 6 \\ 4 \end{pmatrix} \ast \begin{pmatrix} 2 \\ 4 \\ -7 \end{pmatrix}}{\begin{pmatrix} 2 \\ 6 \\ 4 \end{pmatrix} \cdot \begin{pmatrix} 2 \\ 4 \\ -7 \end{pmatrix}} \Leftrightarrow cos(\alpha) = \frac{0}{\sqrt{4+36+16} \cdot \sqrt{4+16+49}} \Leftrightarrow cos(\alpha) = \frac{0}{\sqrt{3864}} \Leftrightarrow cos(\alpha) = 0</math>. | <math>cos(\alpha) = \frac{ \left| \begin{pmatrix} 2 \\ 6 \\ 4 \end{pmatrix} \ast \begin{pmatrix} 2 \\ 4 \\ -7 \end{pmatrix} \right|}{\left| \begin{pmatrix} 2 \\ 6 \\ 4 \end{pmatrix} \right| \cdot \left| \begin{pmatrix} 2 \\ 4 \\ -7 \end{pmatrix} \right|} \Leftrightarrow cos(\alpha) = \frac{0}{\sqrt{4+36+16} \cdot \sqrt{4+16+49}} \Leftrightarrow cos(\alpha) = \frac{0}{\sqrt{3864}} \Leftrightarrow cos(\alpha) = 0</math>. | ||
Nun muss die Formel mit Hilfe des Taschenrechners aufgelöst werden: | Nun muss die Formel mit Hilfe des Taschenrechners aufgelöst werden: | ||
| Zeile 532: | Zeile 532: | ||
Einsetzen in die Formel liefert: | Einsetzen in die Formel liefert: | ||
<math>cos(\alpha) = \frac{ \begin{pmatrix} 0 \\ 0 \\ 1 \end{pmatrix} \ast \begin{pmatrix} 2 \\ 4 \\ -7 \end{pmatrix}}{\begin{pmatrix} 0 \\ 0 \\ 1 \end{pmatrix} \cdot \begin{pmatrix} 2 \\ 4 \\ -7 \end{pmatrix}} \Leftrightarrow cos(\alpha) = \frac{-7}{\sqrt{1} \cdot \sqrt{4+16+49}} \Leftrightarrow cos(\alpha) = \frac{7}{\sqrt{69}}</math> | <math>cos(\alpha) = \frac{\left| \begin{pmatrix} 0 \\ 0 \\ 1 \end{pmatrix} \ast \begin{pmatrix} 2 \\ 4 \\ -7 \end{pmatrix} \right| }{\left| \begin{pmatrix} 0 \\ 0 \\ 1 \end{pmatrix} \right| \cdot \left| \begin{pmatrix} 2 \\ 4 \\ -7 \end{pmatrix} \right|} \Leftrightarrow cos(\alpha) = \frac{|-7|}{\sqrt{1} \cdot \sqrt{4+16+49}} \Leftrightarrow cos(\alpha) = \frac{7}{\sqrt{69}}</math> | ||
Nun muss die Formel mit Hilfe des Taschenrechners aufgelöst werden: | Nun muss die Formel mit Hilfe des Taschenrechners aufgelöst werden: | ||
| Zeile 579: | Zeile 579: | ||
Einsetzen in die Formel liefert: | Einsetzen in die Formel liefert: | ||
<math>cos(\gamma) = \frac{ \begin{pmatrix} 0 \\ 0 \\ 0{,}8 \end{pmatrix} \ast \begin{pmatrix} 0 \\ -1 \\ 0{,}4 \end{pmatrix}}{\begin{pmatrix} 0 \\ 0 \\ 0{,}8 \end{pmatrix} \cdot \begin{pmatrix} 0 \\ -1 \\ 0{,}4 \end{pmatrix}} \Leftrightarrow cos(\gamma) = \frac{\frac{8}{25}}{\frac{4}{5} \cdot \sqrt{\frac{29}{25}}} </math> | <math>cos(\gamma) = \frac{ \left| \begin{pmatrix} 0 \\ 0 \\ 0{,}8 \end{pmatrix} \ast \begin{pmatrix} 0 \\ -1 \\ 0{,}4 \end{pmatrix \right|}}{\left| \begin{pmatrix} 0 \\ 0 \\ 0{,}8 \end{pmatrix} \right| \cdot \left| \begin{pmatrix} 0 \\ -1 \\ 0{,}4 \end{pmatrix} \right|} \Leftrightarrow cos(\gamma) = \frac{\frac{8}{25}}{\frac{4}{5} \cdot \sqrt{\frac{29}{25}}} </math> | ||
Umstellen der Formel ergibt: <math> \gamma=cos^{-1} \left( \frac{\frac{8}{25}}{\frac{4}{5} \cdot \sqrt{\frac{29}{25}}} \right) \Leftrightarrow \gamma \approx 68{,}2 ^\circ </math> | Umstellen der Formel ergibt: <math> \gamma=cos^{-1} \left( \frac{\frac{8}{25}}{\frac{4}{5} \cdot \sqrt{\frac{29}{25}}} \right) \Leftrightarrow \gamma \approx 68{,}2 ^\circ </math> | ||
| Zeile 601: | Zeile 601: | ||
Einsetzen in die Formel liefert: | Einsetzen in die Formel liefert: | ||
<math> cos(\beta)=\frac{ \begin{pmatrix} 0\\ -1\\ 0{,}4 \end{pmatrix} \ast \begin{pmatrix} 0\\ -1\\ -0{,}4 \end{pmatrix}}{\begin{pmatrix} 0\\ -1\\ 0{,}4 \end{pmatrix} \cdot \begin{pmatrix} 0\\ -1\\ -0{,}4 \end{pmatrix}} </math> | <math> cos(\beta)=\frac{\left| \begin{pmatrix} 0\\ -1\\ 0{,}4 \end{pmatrix} \ast \begin{pmatrix} 0\\ -1\\ -0{,}4 \end{pmatrix} \right|}{\left| \begin{pmatrix} 0\\ -1\\ 0{,}4 \end{pmatrix} \right| \cdot \left| \begin{pmatrix} 0\\ -1\\ -0{,}4 \end{pmatrix} \right|} </math> | ||
<math> \Leftrightarrow cos(\beta)=\frac{\frac{21}{25}}{\sqrt{\frac{29}{25}} \cdot \sqrt{\frac{29}{25}}} \Leftrightarrow cos(\beta)=\frac{\frac{21}{25}}{\frac{29}{25}} \Leftrightarrow cos(\beta)=\frac{21}{29}</math> | <math> \Leftrightarrow cos(\beta)=\frac{\frac{21}{25}}{\sqrt{\frac{29}{25}} \cdot \sqrt{\frac{29}{25}}} \Leftrightarrow cos(\beta)=\frac{\frac{21}{25}}{\frac{29}{25}} \Leftrightarrow cos(\beta)=\frac{21}{29}</math> | ||
Version vom 8. Mai 2021, 17:44 Uhr
Hier entsteht das Lernpfadkapitel "Lagebeziehungen und Winkel (Gerade und Ebene, 2 Ebenen)".
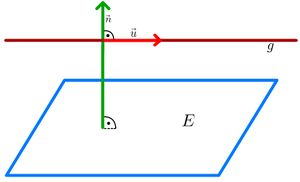
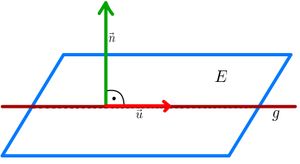
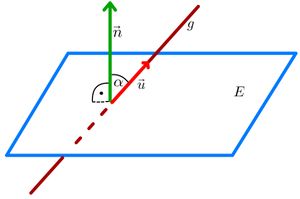
Lagebeziehung Gerade-Ebene
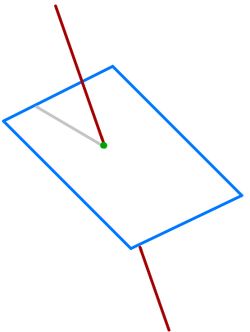
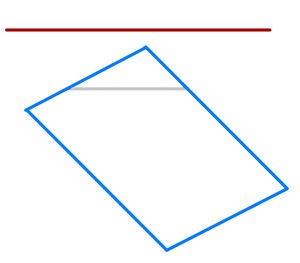
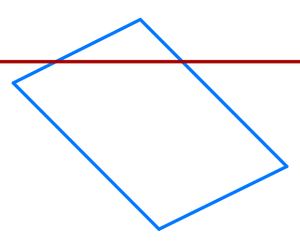
Mögliche Lagebeziehungen zwischen Gerade und Ebene
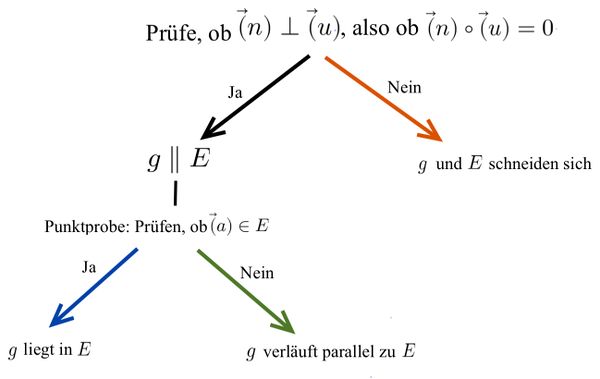
Untersuchung der Lagebeziehung zwischen Gerade und Ebene
⭐Berechnung des Winkels zwischen Gerade und Ebene
Lagebeziehung Ebene-Ebene
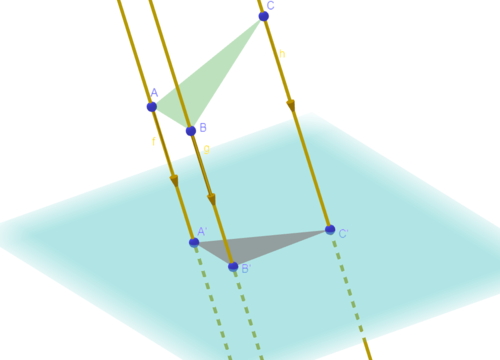
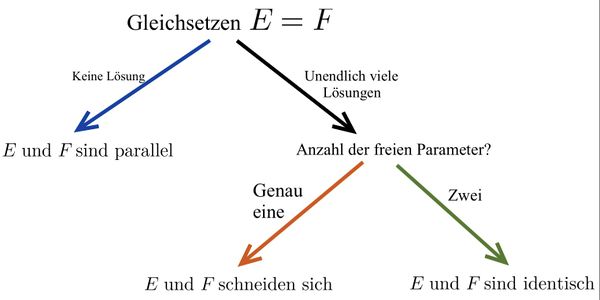
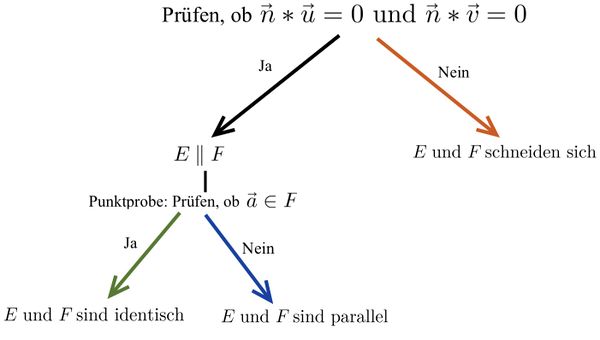
Mögliche Lagebeziehungen zwischen zwei Ebenen
Untersuchung der Lagebeziehung von zwei Ebenen
Zwei Ebenengleichungen in Parameterform
Eine Ebenengleichungen in Parameterform – eine Ebenengleichung in Koordinatenform
Zwei Ebenengleichungen in Koordinatenform
⭐Berechnung des Winkels zwischen Ebene und Ebene