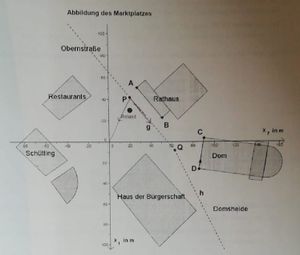
|
|
| Zeile 163: |
Zeile 163: |
| {{LearningApp|app=20418376|width=100%|height=500px}} | | {{LearningApp|app=20418376|width=100%|height=500px}} |
| | Arbeitsmethode | Farbe={{Farbe|orange}} }} | | | Arbeitsmethode | Farbe={{Farbe|orange}} }} |
|
| |
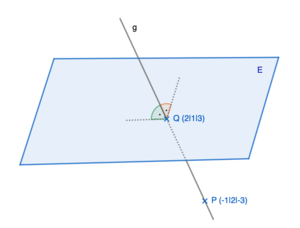
| ==Spurpunkte==
| |
|
| |
| {{Box | Merksatz: Spurpunkte | {{Lösung versteckt|1= Unter einem Spurpunkt versteht man den Schnittpunkt einer Geraden mit der Koordinatenebene.
| |
| Gegeben ist eine Geradengleichung in Parameterform <math>g:\vec{x} = \vec{p} + s \cdot \vec{u}</math>.
| |
| Gesucht sind die Spurpunkte der Geraden. Da es im dreidimensionalen Raum drei Koordinatenebenen gibt
| |
| (die <math>x_1x_2</math>, <math>x_1x_3</math> und <math>x_2x_3</math>-Ebene)
| |
| lassen sich drei Spurpunkte berechnen:
| |
|
| |
| <math>S_1</math> ist der Schnittpunkt von Gerade und <math>x_2x_3</math>-Ebene
| |
|
| |
| <math>S_2</math> ist der Schnittpunkt von Gerade und <math>x_1x_3</math>-Ebene
| |
|
| |
| <math>S_3</math> ist der Schnittpunkt von Gerade und <math>x_1x_2</math>-Ebene | 2=Infobox | 3=Einklappen}} | Merksatz}}
| |
|
| |
| {{Box | Merksatz: Berechnung der Spurpunkte | {{Lösung versteckt|1= Den Spurpunkt <math>S_1</math> berechnet man folgendermaßen:
| |
|
| |
| '''1.''' Setze die erste Koordinate der Geradegleichung gleich Null und berechne den den dazugehörigen Parameter λ.
| |
|
| |
| '''2.''' Setze λ in die Geradegleichung ein, um die Koordinaten des Spurpunktes zu erhalten.
| |
|
| |
| Für <math>S_2</math> und <math>S_3</math> geht man auf gleicher Weise vor.| 2=Infobox | 3=Einklappen}} | Merksatz}}
| |
|
| |
| {{Box | 1= Beispiel: Spurpunkte berechnen | 2= Gegeben ist die Geradengleichung <math>g\colon\vec{x}=\begin{pmatrix} 1 \\ {-}4 \\ 4 \end{pmatrix}+\lambda \cdot \begin{pmatrix} 1 \\ 2 \\ {-}1 \end{pmatrix} </math>
| |
|
| |
| Zum Berechnen von Spurpunkt <math>S_1</math> setzen wir die <math> x_1</math>-Koordinate von <math>S_1</math> gleich Null: <math>S_1(0|?|?)</math>.
| |
|
| |
| '''1.''' <math>x_1 = 0</math> in die erste Zeile der Geradengleichung einsetzen, um <math>\lambda</math> zu berechnen
| |
|
| |
| <div align="center"><math>1 + \lambda = 0</math> → <math>\lambda = {-}1</math></div>
| |
|
| |
| '''2.''' <math>\lambda</math> in die Geradengleichung einsetzt, um den Spurpunkt zu berechnen
| |
|
| |
| <div align="center"><math>g \colon \vec{s_1}=\begin{pmatrix} 1 \\ {-}4 \\ 4 \end{pmatrix} + {-}1 \cdot \begin{pmatrix} 1 \\ 2 \\ {-}1 \end{pmatrix} = \begin{pmatrix} 0 \\ {-}6 \\ 5 \end{pmatrix} </math></div>
| |
|
| |
| Antwort: Der Spurpunkt <math>S_1</math> hat die Koordinaten <math>(0|{-}6|5)</math>.| 3=Hervorhebung1}}
| |
|
| |
| {{Box | Aufgabe 6: Spurpunkte |
| |
|
| |
| Berechne nun die übrigen beiden Spurpunkte <math>S_2</math> und <math>S_3</math> aus dem vorherigen Beispiel und stelle die Ebenengleichung dazu auf.
| |
|
| |
| {{Lösung versteckt|1= '''1.'''<math>{-}4 + s \cdot 2 = 0</math> → <math>s = 2</math>
| |
|
| |
| '''2.''' <math>g \colon \vec{s_2}=\begin{pmatrix} 1 \\ {-}4 \\ 4 \end{pmatrix} + 2 \cdot \begin{pmatrix} 1 \\ 2 \\ {-}1 \end{pmatrix} = \begin{pmatrix} 3 \\ 0 \\ 2 \end{pmatrix} </math>
| |
|
| |
| <math>S_2</math> hat die Koordinaten <math>(3|0|2)</math>.|2=Lösung anzeigen|3=Lösung verbergen}}
| |
|
| |
| {{Lösung versteckt|1= '''1.''' <math>4 - s = 0 </math>→ <math>s = 4</math>
| |
|
| |
| '''2.''' <math>g \colon \vec{s_3}=\begin{pmatrix} 1 \\ {-}4 \\ 4 \end{pmatrix} + 4 \cdot \begin{pmatrix} 1 \\ 2 \\ {-}1 \end{pmatrix} = \begin{pmatrix} 5 \\ 4 \\ 0 \end{pmatrix} </math>
| |
|
| |
| <math>S_3</math> hat die Koordinaten <math>(5|4|0)</math>.|2=Lösung anzeigen|3=Lösung verbergen}}
| |
|
| |
| {{Lösung versteckt|1= <math>E \colon \vec{x}=\begin{pmatrix} 0 \\ {-}6 \\ 5 \end{pmatrix}+s \cdot \begin{pmatrix} 3 \\ 6 \\ {-}3 \end{pmatrix} +t \cdot \begin{pmatrix} 5 \\ 10 \\ {-}5 \end{pmatrix} </math>|2=Lösung anzeigen|3=Lösung verbergen}} | Arbeitsmethode | Farbe={{Farbe|orange}} }}
| |
|
| |
|
| |
| {{Box | Aufgabe 7: Spurpunkte berechnen |
| |
|
| |
| Gib die Schnittpunkte der Geraden g mit den Koordinatenebenen an (Spurpunkte der Geraden)
| |
|
| |
| '''a)''' <math>g \colon \vec{x}=\begin{pmatrix} 1 \\ 1 \\ 1 \end{pmatrix} + r \cdot \begin{pmatrix} {-}1 \\ 2 \\ 1 \end{pmatrix} </math>
| |
|
| |
| '''b)''' <math>g\colon \vec{x}=\begin{pmatrix} {-}2 \\ 3 \\ 5 \end{pmatrix} + s \cdot \begin{pmatrix} 1 \\ 1 \\ 0 \end{pmatrix} </math>
| |
|
| |
| {{Lösung versteckt|1= '''a)''' <math>S_1 = (0 | 3 | 2), S_2 = (1{,}5 | 0 | 0{,}5), S_3 = (2 | {-}1 | 0)</math>|2=Lösung anzeigen|3=Lösung verbergen}}
| |
|
| |
| {{Lösung versteckt|1= '''b)''' <math>S_1 = (0 | 5 | 5), S_2 = ({-}5 | 0 | 5),
| |
|
| |
| S_3</math> kann in diesem Fall nicht berechnet werden. Was heißt dies für unsere Gerade?|2=Lösung anzeigen|3=Lösung verbergen}}
| |
|
| |
| {{Lösung versteckt|1= Unsere Gerade aus Aufgabe '''b)''' schneidet die <math>x_1x_2</math>-Ebene nicht.|2=Lösung anzeigen|3=Lösung verbergen}} | Arbeitsmethode | Farbe={{Farbe|orange}} }}
| |
|
| |
|
| {{Box | Aufgabe 8: Ein U-Boot taucht auf | | | {{Box | Aufgabe 8: Ein U-Boot taucht auf | |