Digitale Werkzeuge in der Schule/Fit für VERA-8/Terme: Unterschied zwischen den Versionen
Aus ZUM Projektwiki
Keine Bearbeitungszusammenfassung Markierung: 2017-Quelltext-Bearbeitung |
Markierung: 2017-Quelltext-Bearbeitung |
||
| (31 dazwischenliegende Versionen von 2 Benutzern werden nicht angezeigt) | |||
| Zeile 260: | Zeile 260: | ||
=====Aufgabe===== | =====Aufgabe===== | ||
{{Box | 1= Aufgabe 2: Wähle die richtige Antwort | 2= a) Was lässt sich sinnvollerweise ausklammern? | {{Box | 1= Aufgabe 2: Wähle die richtige Antwort | 2= '''a)''' Was lässt sich sinnvollerweise ausklammern? | ||
{{Lösung versteckt|1=Schaue dir (nochmal) die [[#Terme faktorisieren|Beispiele]] aus dem Video von Lehrer Schmidt an. | {{Lösung versteckt|1=Schaue dir (nochmal) die [[#Terme faktorisieren|Beispiele]] aus dem Video von Lehrer Schmidt an. | ||
|2=Tipp|3=Verbergen}} | |2=Tipp|3=Verbergen}} | ||
<br \> | <br \> | ||
<div class="multiplechoice-quiz"> | <div class="multiplechoice-quiz"> | ||
(i) <math> 9x - 15 </math> (!<math> 5 </math>) (<math> 3 </math>) (!<math> 9 </math>) (!<math> x</math>) <math> </math> | '''(i)''' <math> 9x - 15 </math> (!<math> 5 </math>) (<math> 3 </math>) (!<math> 9 </math>) (!<math> x</math>) <math> </math> | ||
(ii) <math> -36 + 12x </math> (!<math> 9 </math>) (<math> 12 </math>) (!<math> 24 </math>) (!<math> x</math>) | '''(ii)''' <math> -36 + 12x </math> (!<math> 9 </math>) (<math> 12 </math>) (!<math> 24 </math>) (!<math> x</math>) | ||
(iii) <math> 5xy + 4xz + 3x </math> (!<math> 5 </math>) (<math> x </math>) (!<math> y </math>) (!<math> 2z </math>) | '''(iii)''' <math> 5xy + 4xz + 3x </math> (!<math> 5 </math>) (<math> x </math>) (!<math> y </math>) (!<math> 2z </math>) | ||
</div> | </div> | ||
b) Wie sieht der erste Zwischenschritt beim Ausklammern aus? <br \> | '''b)''' Wie sieht der erste Zwischenschritt beim Ausklammern aus? <br \> | ||
{{Lösung versteckt|1=Schaue bei Teilaufgabe a) nach, was du ausklammerst. | {{Lösung versteckt|1=Schaue bei Teilaufgabe a) nach, was du ausklammerst. | ||
|2=Tipp|3=Verbergen}} | |2=Tipp|3=Verbergen}} | ||
<div class="multiplechoice-quiz"> | <div class="multiplechoice-quiz"> | ||
(i) <math> 9x - 15 </math> (<math> 3 \cdot 3x - 3 \cdot 5 </math>) (!<math> 3 \cdot 9x - 3 \cdot 5 </math>) (!<math> 3 \cdot 3x + 3 \cdot 5 </math>) (!<math> 3 \cdot x -15 </math>) | '''(i)''' <math> 9x - 15 </math> (<math> 3 \cdot 3x - 3 \cdot 5 </math>) (!<math> 3 \cdot 9x - 3 \cdot 5 </math>) (!<math> 3 \cdot 3x + 3 \cdot 5 </math>) (!<math> 3 \cdot x -15 </math>) | ||
(ii) <math> -36 + 12x </math> (<math> 12 \cdot [-3] + 12 \cdot x </math>) (!<math> 12 \cdot 3 + 12 \cdot x </math>) (!<math> 12 \cdot [-3] - 12 \cdot x </math>) (!<math> 12 \cdot [-3] + x </math>) | '''(ii)''' <math> -36 + 12x </math> (<math> 12 \cdot [-3] + 12 \cdot x </math>) (!<math> 12 \cdot 3 + 12 \cdot x </math>) (!<math> 12 \cdot [-3] - 12 \cdot x </math>) (!<math> 12 \cdot [-3] + x </math>) | ||
</div> | </div> | ||
c) Klammere komplett aus: | '''c)''' Klammere komplett aus: | ||
{{Lösung versteckt|1=Schaue bei Teilaufgabe a) nach, was du ausklammerst und bei b) wie dein erster Zwischenschritt aussieht. Mache zur Überprüfung die Probe wie es im Kapitel zum [[#Terme faktorisieren|''Faktorisieren'']] erklärt ist. <br /> | {{Lösung versteckt|1=Schaue bei Teilaufgabe a) nach, was du ausklammerst und bei b) wie dein erster Zwischenschritt aussieht. Mache zur Überprüfung die Probe wie es im Kapitel zum [[#Terme faktorisieren|''Faktorisieren'']] erklärt ist. <br /> | ||
|2=Tipp|3=Verbergen}} | |2=Tipp|3=Verbergen}} | ||
<div class="multiplechoice-quiz"> | <div class="multiplechoice-quiz"> | ||
(i) <math> 9x - 15 </math> (<math> 3 \cdot [3x - 5] </math>) (!<math> 3 \cdot [9x - 15] </math>) (!<math> 3 \cdot [x - 5] </math>) (!<math> 3 \cdot [3x + 5] </math>) | '''(i)''' <math> 9x - 15 </math> (<math> 3 \cdot [3x - 5] </math>) (!<math> 3 \cdot [9x - 15] </math>) (!<math> 3 \cdot [x - 5] </math>) (!<math> 3 \cdot [3x + 5] </math>) | ||
(ii) <math> -36 + 12x </math> (<math> 12 \cdot [-3 + x] </math>) (!<math> 12 \cdot [3 + x ] </math>) (!<math> 12 \cdot [6 + 3x] </math>) (!<math> 3 \cdot [3 + x] </math>) | '''(ii)''' <math> -36 + 12x </math> (<math> 12 \cdot [-3 + x] </math>) (!<math> 12 \cdot [3 + x ] </math>) (!<math> 12 \cdot [6 + 3x] </math>) (!<math> 3 \cdot [3 + x] </math>) | ||
(iii) <math> 5xy + 4xz + 3x </math> (!<math> x \cdot [54yz + 3]</math>) (<math> x \cdot [5y + 4z + 3] </math>) (!<math> 2x \cdot [5y + 4z + 3]</math>) (!<math> 5 \cdot [xy + 4xz + 3x]</math>) | '''(iii)''' <math> 5xy + 4xz + 3x </math> (!<math> x \cdot [54yz + 3]</math>) (<math> x \cdot [5y + 4z + 3] </math>) (!<math> 2x \cdot [5y + 4z + 3]</math>) (!<math> 5 \cdot [xy + 4xz + 3x]</math>) | ||
</div> | </div> | ||
| 3= Arbeitsmethode | Farbe={{Farbe|orange}} }} | | 3= Arbeitsmethode | Farbe={{Farbe|orange}} }} | ||
| Zeile 320: | Zeile 320: | ||
| 3=Arbeitsmethode}} | | 3=Arbeitsmethode}} | ||
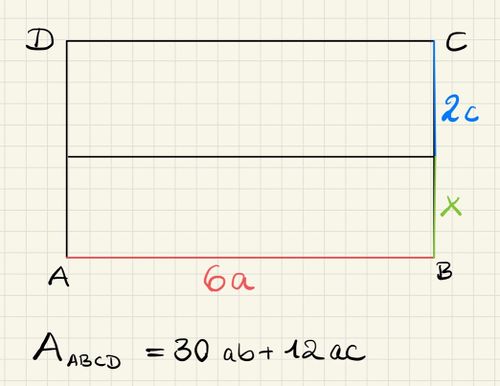
{{Box | 1= Aufgabe 4: Distributivgesetz veranschaulicht | 2= | {{Box | 1= Aufgabe 4: Distributivgesetz veranschaulicht | 2= '''a)''' Wie lang ist die Strecke <math> x </math>?<br /> | ||
[[Datei:Knobel .jpg|500px|links]] <br /> <br /> <br /> <br /> <br /> <br /> <br /> <br /> <br /> <br /> <br /> <br /> <br /> <br /> <br /> <br /> <br /> <br /> | [[Datei:Knobel .jpg|500px|links]] <br /> <br /> <br /> <br /> <br /> <br /> <br /> <br /> <br /> <br /> <br /> <br /> <br /> <br /> <br /> <br /> <br /> <br /> | ||
{{Lösung versteckt|1=Was kannst du aus dem Term <math> 30ab+12ac </math>, der den Flächeninhalt des Rechtecks beschreibt, ausklammern? | {{Lösung versteckt|1=Was kannst du aus dem Term <math> 30ab+12ac </math>, der den Flächeninhalt des Rechtecks beschreibt, ausklammern? | ||
| Zeile 335: | Zeile 335: | ||
<math> x = </math> '''5b()''' | <math> x = </math> '''5b()''' | ||
</div> | </div> | ||
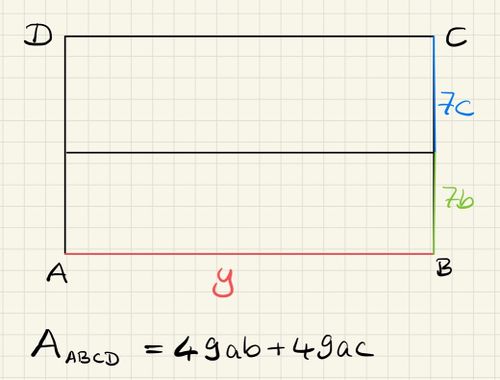
'''b)''' Wie lang ist die Strecke <math> y </math>?<br /> | |||
[[Datei:Knobelaufgabe.jpg|500px|links]] <br /> <br /> <br /> <br /> <br /> <br /> <br /> <br /> <br /> <br /> <br /> <br /> <br /> <br /> <br /> <br /> <br /> <br /> | [[Datei:Knobelaufgabe.jpg|500px|links]] <br /> <br /> <br /> <br /> <br /> <br /> <br /> <br /> <br /> <br /> <br /> <br /> <br /> <br /> <br /> <br /> <br /> <br /> | ||
{{Lösung versteckt|1=Was kannst du aus dem Term <math> 49ab+49ac </math>, der den Flächeninhalt des Rechtecks beschreibt, ausklammern? | {{Lösung versteckt|1=Was kannst du aus dem Term <math> 49ab+49ac </math>, der den Flächeninhalt des Rechtecks beschreibt, ausklammern? | ||
| Zeile 375: | Zeile 375: | ||
{{Box|1=Beachte|2= | {{Box|1=Beachte|2= | ||
* Bisher hast du lediglich die Herleitung der | * Bisher hast du lediglich die Herleitung der '''1. binomischen Formel''' kennengelernt. Die Herleitungen der '''2. und 3. binomischen Formel''' erfolgen sehr ähnlich und werden hier nicht thematisiert. Falls du dich trotzdem dafür interessierst, schau doch gerne mal bei Serlo vorbei: https://de.serlo.org/mathe/terme-gleichungen/terme-variablen/binomische-formeln | ||
* Die binomischen Formeln werden dir im Laufe deiner Schulzeit immer wieder begegnen, weshalb du sie unbedingt auswendig können solltest. Falls dir dies schwer fällt, schaue dir folgendes Video dazu an. <br \> <br \> <div align="center">{{#ev:youtube|EYbvhWEG6kE}}</div>|3=Merksatz}} | * Die binomischen Formeln werden dir im Laufe deiner Schulzeit immer wieder begegnen, weshalb du sie unbedingt auswendig können solltest. Falls dir dies schwer fällt, schaue dir folgendes Video dazu an. <br \> <br \> <div align="center">{{#ev:youtube|EYbvhWEG6kE}}</div>|3=Merksatz}} | ||
| Zeile 383: | Zeile 383: | ||
{{Lösung versteckt|1= | {{Lösung versteckt|1= | ||
Zur Erinnerung: <div align="center">'''1. binomische Formel:''' <math>({\color{green}a}+{\color{blue}b})^2 = {\color{green}a}^2+2{\color{green}a}{\color{blue}b}+{\color{blue}b}^2 </math> </div> <br \> <br \> | Zur Erinnerung: <div align="center">'''1. binomische Formel:''' <math>({\color{green}a}+{\color{blue}b})^2 = {\color{green}a}^2+2{\color{green}a}{\color{blue}b}+{\color{blue}b}^2 </math> </div> <br \> <br \> | ||
Für a und b können verschiedene Zahlen eingesetzt werden: <br \> | Für <math>a</math> und <math>b</math> können verschiedene Zahlen eingesetzt werden: <br \> | ||
a)<math>({\color{green}5}+{\color{blue}3})^2 = {\color{green}5}^2+2 \cdot {\color{green}5} \cdot {\color{blue}3}+{\color{blue}3}^2 = 25+30+9 = 64 (=8^2)</math> <br \> <br \> | a)<math>({\color{green}5}+{\color{blue}3})^2 = {\color{green}5}^2+2 \cdot {\color{green}5} \cdot {\color{blue}3}+{\color{blue}3}^2 = 25+30+9 = 64 (=8^2)</math> <br \> <br \> | ||
Für a und b können auch andere Variablen eingesetzt werden: <br \> | Für <math>a</math> und <math>b</math> können auch andere Variablen eingesetzt werden: <br \> | ||
b)<math>({\color{green}(uv)}+{\color{blue}w})^2 = {\color{green}(uv)}^2+2{\color{green}(uv)}{\color{blue}w}+{\color{blue}w}^2 = u^2v^2+2 \cdot uvw+w^2 </math> <br \> <br \> | b)<math>({\color{green}(uv)}+{\color{blue}w})^2 = {\color{green}(uv)}^2+2{\color{green}(uv)}{\color{blue}w}+{\color{blue}w}^2 = u^2v^2+2 \cdot uvw+w^2 </math> <br \> <br \> | ||
Selbst längere Terme kann man für a und b einsetzen: <br \> | Selbst längere Terme kann man für <math>a</math> und <math>b</math> einsetzen: <br \> | ||
c)<math>({\color{green}(2s+t)}+{\color{blue}u})^2 = {\color{green}(2s+t)}^2+2{\color{green}(2s+t)}{\color{blue}u}+{\color{blue}u}^2 </math> <br \> | c)<math>({\color{green}(2s+t)}+{\color{blue}u})^2 = {\color{green}(2s+t)}^2+2{\color{green}(2s+t)}{\color{blue}u}+{\color{blue}u}^2 </math> <br \> | ||
|2=Beispiele zur 1. binomischen Formel|3=Beispiele ausblenden}} | |2=Beispiele zur 1. binomischen Formel|3=Beispiele ausblenden}} | ||
| Zeile 410: | Zeile 410: | ||
<div class="zuordnungs-quiz"> | <div class="zuordnungs-quiz"> | ||
{{{!}} | {{{!}} | ||
{{!}} 1. binomische Formel {{!}}{{!}} <math forcemathmode="png">(x+19)^2</math> {{!}}{{!}} <math forcemathmode="png">({3\over 4}+p)^2</math> {{!}}{{!}} <math forcemathmode="png">(1,34+\sqrt{5})^2</math> | {{!}} 1. binomische Formel {{!}}{{!}} <math forcemathmode="png">(x+19)^2</math> {{!}}{{!}} <math forcemathmode="png">({3\over 4}+p)^2</math> {{!}}{{!}} <math forcemathmode="png">(1{,}34+\sqrt{5})^2</math> | ||
{{!}}- | {{!}}- | ||
{{!}} 2. binomische Formel {{!}}{{!}} <math forcemathmode="png">(19-x)^2</math> {{!}}{{!}} <math forcemathmode="png">(3-5)^2</math> {{!}}{{!}} <math forcemathmode="png">(25-y)^2</math> | {{!}} 2. binomische Formel {{!}}{{!}} <math forcemathmode="png">(19-x)^2</math> {{!}}{{!}} <math forcemathmode="png">(3-5)^2</math> {{!}}{{!}} <math forcemathmode="png">(25-y)^2</math> | ||
{{!}}- | {{!}}- | ||
{{!}} 3. binomische Formel {{!}}{{!}} <math forcemathmode="png">(5+t)(5-t)</math> {{!}}{{!}} <math forcemathmode="png">({3\over 8}-7)(7+{3\over 8})</math> {{!}}{{!}} <math forcemathmode="png"> (1,37-2)(1,37+2) </math> | {{!}} 3. binomische Formel {{!}}{{!}} <math forcemathmode="png">(5+t)(5-t)</math> {{!}}{{!}} <math forcemathmode="png">({3\over 8}-7)(7+{3\over 8})</math> {{!}}{{!}} <math forcemathmode="png">(1{,}37-2)(1{,}37+2)</math> | ||
{{!}}- | {{!}}- | ||
{{!}} Das ist keine binomische Formel {{!}}{{!}} <math forcemathmode="png">(4+7)(5-7)</math> {{!}}{{!}} <math forcemathmode="png">(5+7)^{1\over 2}</math> {{!}}{{!}} <math forcemathmode="png">s+3^2</math> | {{!}} Das ist keine binomische Formel {{!}}{{!}} <math forcemathmode="png">(4+7)(5-7)</math> {{!}}{{!}} <math forcemathmode="png">(5+7)^{1\over 2}</math> {{!}}{{!}} <math forcemathmode="png">s+3^2</math> | ||
| Zeile 428: | Zeile 428: | ||
<div class="lueckentext-quiz"> | <div class="lueckentext-quiz"> | ||
a)<math> 225+30a+a^2 = (</math>'''15()'''<math>+</math>'''a()'''<math>)^2 </math> <br /> | a) <math> 225+30a+a^2 = (</math>'''15()'''<math>+</math>'''a()'''<math>)^2 </math> <br /> | ||
b)<math> 9a^2-16b^2 = (</math>'''3a()'''<math>+</math>'''4b()'''<math>)\cdot ( </math>'''3a()'''<math>-</math>'''4b()''') <br /> | b) <math> 9a^2-16b^2 = (</math>'''3a()'''<math>+</math>'''4b()'''<math>)\cdot ( </math>'''3a()'''<math>-</math>'''4b()''') <br /> | ||
c)<math> 81u^2-36u+4 = (</math>'''9u()'''<math>-</math>'''2()'''<math>)^2 </math> <br /> | c) <math> 81u^2-36u+4 = (</math>'''9u()'''<math>-</math>'''2()'''<math>)^2 </math> <br /> | ||
d)<math> 4m^2+28m+49 = (</math>'''2m()'''<math>+</math>'''7()'''<math>)^2 </math> <br /> | d) <math> 4m^2+28m+49 = (</math>'''2m()'''<math>+</math>'''7()'''<math>)^2 </math> <br /> | ||
e)<math> 64y^2-160yz+100z^2 = (</math>'''8y()'''<math>-</math>'''10z()'''<math>)^2 </math> <br /> | e) <math> 64y^2-160yz+100z^2 = (</math>'''8y()'''<math>-</math>'''10z()'''<math>)^2 </math> <br /> | ||
f)<math> 36u^2-121w^2 = (</math>'''6u()'''<math>+</math>'''11w()'''<math>)\cdot ( </math>'''6u()'''<math>-</math>'''11w()''') <br /> | f) <math> 36u^2-121w^2 = (</math>'''6u()'''<math>+</math>'''11w()'''<math>)\cdot ( </math>'''6u()'''<math>-</math>'''11w()''') <br /> | ||
</div> | </div> | ||
| Zeile 441: | Zeile 441: | ||
{{Lösung versteckt|1= | {{Lösung versteckt|1= | ||
Wir suchen die passende binomische Formel für den Term <math> 9x^2-6xy+y^2 </math>. | Wir suchen die passende binomische Formel für den Term <math> 9x^2-6xy+y^2 </math>. | ||
Die Anzahl der Summanden bzw. Minuenden geben uns Auskunft darüber, welche | Die Anzahl der Summanden bzw. Minuenden geben uns Auskunft darüber, welche binomische Formel wir anwenden können. In diesem Fall haben wir '''zwei Summanden''' und '''einen Minuenden'''. Dies stimmt mit der '''2. binomischen Formel''' überein: <br /> | ||
<math>( | <div align="center"> <math>(a-b)^2 = a^2-2ab+b^2 </math>. Unsere binomische Formel hat also die Form <math>(a-b)^2 </math>. </div> <br /> | ||
Nun müssen wir noch a und b herausfinden. Wir wissen <math> a^2 = 9x^2 </math> und <math> b^2 = y^2 </math>. | Nun müssen wir noch a und b herausfinden. Wir wissen, dass <math> a^2 = 9x^2 </math> und <math> b^2 = y^2 </math>. | ||
Nun ziehen wir aus diesen Ausdrücken die Wurzel, um a und b zu erhalten: <br /> | |||
<math> a = \sqrt{a^2} = \sqrt{9x^2} = 3x </math> und <math> b = \sqrt{b^2} = \sqrt{y^2} = y </math>. | <div align="center"> <math> a = \sqrt{a^2} = \sqrt{9x^2} = 3x </math> und <math> b = \sqrt{b^2} = \sqrt{y^2} = y </math>. </div> | ||
Also lautet die binomische Formel <math> (3x-y)^2 </math>. | Also lautet die binomische Formel <math> (3x-y)^2 </math>. | ||
Probe: <math> ({\color{green}3x}-{\color{blue}y})^2 = {\color{green}(3x)}^2-2 \cdot {\color{green}3x}{\color{blue}y}+{\color{blue}y}^2 = 9x^2-6xy+y^2 </math>. | <br /> <br /> | ||
Das Vorgehen für die | Probe:<div align="center"> <math> ({\color{green}3x}-{\color{blue}y})^2 = {\color{green}(3x)}^2-2 \cdot {\color{green}3x}{\color{blue}y}+{\color{blue}y}^2 = 9x^2-6xy+y^2 </math>. </div> <br /> | ||
Das Vorgehen für die 1. und 3. binomische Formel erfolgt sehr ähnlich. Falls du trotzdem Probleme beim Lösen der Aufgabe hast, siehe dir Tipp 2 an.|2=Beispiel|3=Beispiel verbergen}} | |||
{{Lösung versteckt|1= | {{Lösung versteckt|1= | ||
* Bei '''drei Summanden''' wendest du die '''1. binomische Formel''' an. | * Bei '''drei Summanden''' wendest du die '''1. binomische Formel''' an. | ||
* Bei '''zwei Summanden und einem Minuenden''' wendest du die '''2. binomische Formel''' an. | * Bei '''zwei Summanden und einem Minuenden''' wendest du die '''2. binomische Formel''' an. | ||
* Bei '''einem Summanden und einem Minuenden''' wendest du die '''3. binomische Formel''' an.|2=Tipp 2|3=Tipp ausblenden}} | * Bei '''einem Summanden und einem Minuenden''' wendest du die '''3. binomische Formel''' an.|2=Tipp 2|3=Tipp ausblenden}}| 3=Arbeitsmethode}} | ||
{{Box | 1=Aufgabe 3: Warum ist das so?| 2= | {{Box | 1=Aufgabe 3: Warum ist das so?| 2= | ||
Aktuelle Version vom 16. Dezember 2020, 12:05 Uhr
1) Terme zusammenfassen
Einführung
Aufgaben
2) Terme ausmultiplizieren und faktorisieren
Terme ausmultiplizieren
Aufgabe
Terme faktorisieren
Aufgabe
Weitere Aufgaben zum Ausmultiplizieren und Faktorisieren
3) Binomische Formeln
Was sind die binomischen Formeln?
Herleitung der binomischen Formeln
Beispiele
Aufgaben