Digitale Werkzeuge in der Schule/Fit für VERA-8/Stochastik: Unterschied zwischen den Versionen
Aus ZUM Projektwiki
Markierung: 2017-Quelltext-Bearbeitung |
Markierung: 2017-Quelltext-Bearbeitung |
||
| (7 dazwischenliegende Versionen von 3 Benutzern werden nicht angezeigt) | |||
| Zeile 60: | Zeile 60: | ||
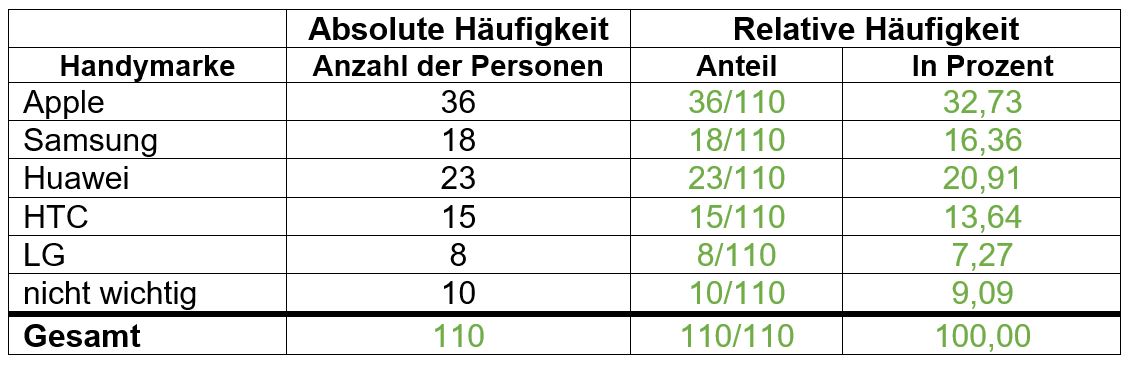
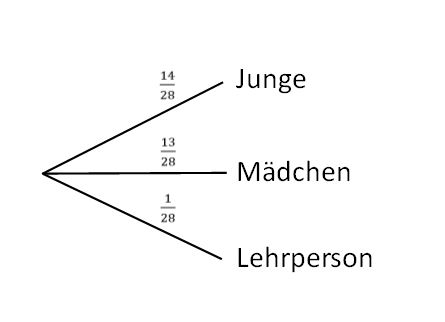
Auf dem Münsteraner Marktplatz wird eine Umfrage zum Thema Lieblingshandymarke durchgeführt. | Auf dem Münsteraner Marktplatz wird eine Umfrage zum Thema Lieblingshandymarke durchgeführt. | ||
<math>36</math> Personen beantworteten die Frage mit „Apple“, <math> | <math>10</math> Personen gaben bei der Umfrage an, dass ihnen die Handymarke nicht wichtig ist. <math>36</math> Personen beantworteten die Frage mit „Apple“, <math>8</math> Personen mit „LG“, <math>23</math> Personen mit „Huawei“, <math>15</math> Personen mit „HTC“ und <math>18</math> Personen mit „Samsung“. | ||
'''a)''' Fülle die Tabelle vollständig aus. Beachte, dass du den Bruch in folgender Form a/b eintippen solltest und ihn nicht kürzen darfst. | '''a)''' Fülle die Tabelle vollständig aus. Beachte, dass du den Bruch in folgender Form a/b eintippen solltest und ihn nicht kürzen darfst. | ||
| Zeile 72: | Zeile 72: | ||
! Anzahl der Personen | ! Anzahl der Personen | ||
! Anteil | ! Anteil | ||
! Prozent | ! in Prozent | ||
{{!-}} | {{!-}} | ||
{{!}} Apple | {{!}} Apple | ||
{{!}} <div class="lueckentext-quiz"> '''36()''' </div> | {{!}} <div class="lueckentext-quiz"> '''36()''' </div> | ||
{{!}} <div class="lueckentext-quiz"> '''36/110()''' </div> | {{!}} <div class="lueckentext-quiz"> '''36/110()''' </div> | ||
{{!}} <div class="lueckentext-quiz"> '''32,73 | {{!}} <div class="lueckentext-quiz"> '''32,73()''' </div> | ||
{{!-}} | {{!-}} | ||
{{!}} Samsung | {{!}} Samsung | ||
{{!}} <div class="lueckentext-quiz"> '''18()''' </div> | {{!}} <div class="lueckentext-quiz"> '''18()''' </div> | ||
{{!}} <div class="lueckentext-quiz"> '''18/110()''' </div> | {{!}} <div class="lueckentext-quiz"> '''18/110()''' </div> | ||
{{!}} <div class="lueckentext-quiz"> '''16,36 | {{!}} <div class="lueckentext-quiz"> '''16,36()''' </div> | ||
{{!-}} | {{!-}} | ||
{{!}} Huawei | {{!}} Huawei | ||
{{!}} <div class="lueckentext-quiz"> '''23()''' </div> | {{!}} <div class="lueckentext-quiz"> '''23()''' </div> | ||
{{!}} <div class="lueckentext-quiz"> '''23/110()''' </div> | {{!}} <div class="lueckentext-quiz"> '''23/110()''' </div> | ||
{{!}} <div class="lueckentext-quiz"> '''20,91 | {{!}} <div class="lueckentext-quiz"> '''20,91()''' </div> | ||
{{!-}} | {{!-}} | ||
{{!}} HTC | {{!}} HTC | ||
{{!}} <div class="lueckentext-quiz"> '''15()''' </div> | {{!}} <div class="lueckentext-quiz"> '''15()''' </div> | ||
{{!}} <div class="lueckentext-quiz"> '''15/110()''' </div> | {{!}} <div class="lueckentext-quiz"> '''15/110()''' </div> | ||
{{!}} <div class="lueckentext-quiz"> '''13,64 | {{!}} <div class="lueckentext-quiz"> '''13,64()''' </div> | ||
{{!-}} | {{!-}} | ||
{{!}} LG | {{!}} LG | ||
{{!}} <div class="lueckentext-quiz"> '''8()''' </div> | {{!}} <div class="lueckentext-quiz"> '''8()''' </div> | ||
{{!}} <div class="lueckentext-quiz"> '''8/110()''' </div> | {{!}} <div class="lueckentext-quiz"> '''8/110()''' </div> | ||
{{!}} <div class="lueckentext-quiz"> '''7,27 | {{!}} <div class="lueckentext-quiz"> '''7,27()''' </div> | ||
{{!-}} | {{!-}} | ||
{{!}} nicht wichtig | {{!}} nicht wichtig | ||
{{!}} <div class="lueckentext-quiz"> '''10()''' </div> | {{!}} <div class="lueckentext-quiz"> '''10()''' </div> | ||
{{!}} <div class="lueckentext-quiz"> '''10/110()''' </div> | {{!}} <div class="lueckentext-quiz"> '''10/110()''' </div> | ||
{{!}} <div class="lueckentext-quiz"> '''9,09 | {{!}} <div class="lueckentext-quiz"> '''9,09()''' </div> | ||
{{!-}} | {{!-}} | ||
! Gesamt | ! Gesamt | ||
{{!}} <div class="lueckentext-quiz"> '''110()''' </div> | {{!}} <div class="lueckentext-quiz"> '''110()''' </div> | ||
{{!}} <div class="lueckentext-quiz"> '''110/110()''' </div> | {{!}} <div class="lueckentext-quiz"> '''110/110()''' </div> | ||
{{!}} <math>100{,}00</math> | {{!}} <math>100{,}00</math> | ||
{{!)}} | {{!)}} | ||
| Zeile 118: | Zeile 118: | ||
{{Lösung versteckt| 1= Runde die berechnete Prozentzahl auf zwei Nachkommastellen genau. |2= Prozentzahl runden |3= }} | {{Lösung versteckt| 1= Runde die berechnete Prozentzahl auf zwei Nachkommastellen genau. |2= Prozentzahl runden |3= }} | ||
{{Lösung versteckt| 1=[[Datei: | {{Lösung versteckt| 1= [[Datei:Lösung-1a.jpg|zentriert]] |2= Lösung |3= }} | ||
'''b)''' Die drei Bilder zeigen unterschiedliche Säulendiagramme. | '''b)''' Die drei Bilder zeigen unterschiedliche Säulendiagramme. | ||
| Zeile 147: | Zeile 147: | ||
{{Box | {{Box | ||
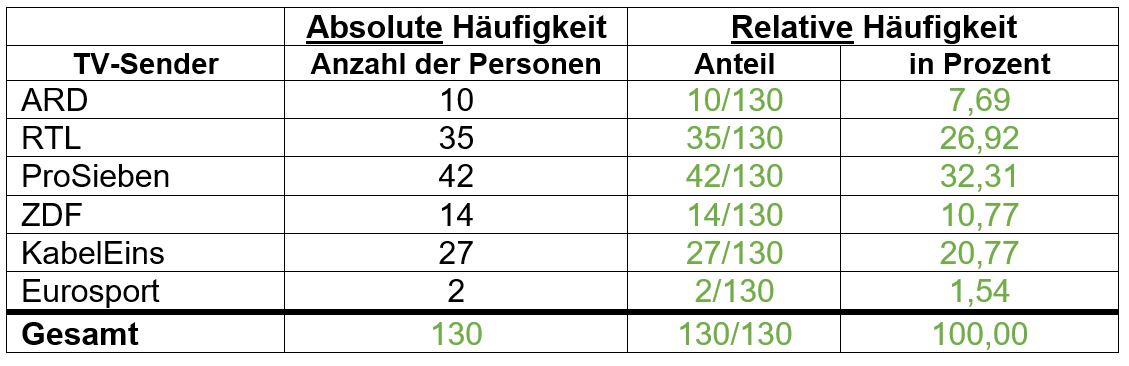
| 1= Aufgabe 2 | | 1= Aufgabe 2: TV Sender | ||
| 2= | | 2= | ||
| Zeile 210: | Zeile 168: | ||
! Anzahl der Personen | ! Anzahl der Personen | ||
! Anteil | ! Anteil | ||
! Prozent | ! in Prozent | ||
{{!-}} | {{!-}} | ||
{{!}} ARD | {{!}} ARD | ||
{{!}} <math>10</math> | {{!}} <math>10</math> | ||
{{!}} <div class="lueckentext-quiz"> '''10/130()''' </div> | {{!}} <div class="lueckentext-quiz"> '''10/130()''' </div> | ||
{{!}} <div class="lueckentext-quiz"> '''7,69 | {{!}} <div class="lueckentext-quiz"> '''7,69()''' </div> | ||
{{!-}} | {{!-}} | ||
{{!}} RTL | {{!}} RTL | ||
{{!}} <math>35</math> | {{!}} <math>35</math> | ||
{{!}} <div class="lueckentext-quiz"> '''35/130()''' </div> | {{!}} <div class="lueckentext-quiz"> '''35/130()''' </div> | ||
{{!}} <div class="lueckentext-quiz"> '''26,92 | {{!}} <div class="lueckentext-quiz"> '''26,92()''' </div> | ||
{{!-}} | {{!-}} | ||
{{!}} ProSieben | {{!}} ProSieben | ||
{{!}} <math>42</math> | {{!}} <math>42</math> | ||
{{!}} <div class="lueckentext-quiz"> '''42/130()''' </div> | {{!}} <div class="lueckentext-quiz"> '''42/130()''' </div> | ||
{{!}} <div class="lueckentext-quiz"> '''32,31 | {{!}} <div class="lueckentext-quiz"> '''32,31()''' </div> | ||
{{!-}} | {{!-}} | ||
{{!}} ZDF | {{!}} ZDF | ||
{{!}} <math>14</math> | {{!}} <math>14</math> | ||
{{!}} <div class="lueckentext-quiz"> '''14/130()''' </div> | {{!}} <div class="lueckentext-quiz"> '''14/130()''' </div> | ||
{{!}} <div class="lueckentext-quiz"> '''10,77 | {{!}} <div class="lueckentext-quiz"> '''10,77()''' </div> | ||
{{!-}} | {{!-}} | ||
{{!}} KabelEins | {{!}} KabelEins | ||
{{!}} <math>27</math> | {{!}} <math>27</math> | ||
{{!}} <div class="lueckentext-quiz"> '''27/130()''' </div> | {{!}} <div class="lueckentext-quiz"> '''27/130()''' </div> | ||
{{!}} <div class="lueckentext-quiz"> '''20,77 | {{!}} <div class="lueckentext-quiz"> '''20,77()''' </div> | ||
{{!-}} | {{!-}} | ||
{{!}} Eurosport | {{!}} Eurosport | ||
{{!}} <math>2</math> | {{!}} <math>2</math> | ||
{{!}} <div class="lueckentext-quiz"> '''2/130()''' </div> | {{!}} <div class="lueckentext-quiz"> '''2/130()''' </div> | ||
{{!}} <div class="lueckentext-quiz"> '''1,54 | {{!}} <div class="lueckentext-quiz"> '''1,54()''' </div> | ||
{{!-}} | {{!-}} | ||
! Gesamt | ! Gesamt | ||
{{!}} <div class="lueckentext-quiz"> '''130()''' </div> | {{!}} <div class="lueckentext-quiz"> '''130()''' </div> | ||
{{!}} <div class="lueckentext-quiz"> '''130/130()''' </div> | {{!}} <div class="lueckentext-quiz"> '''130/130()''' </div> | ||
{{!}} < | {{!}} <math>100{,}00</math> | ||
{{!)}} | {{!)}} | ||
| Zeile 256: | Zeile 214: | ||
{{Lösung versteckt| 1= Runde die berechnete Prozentzahl auf zwei Nachkommastellen genau. |2= Prozentzahl runden |3= }} | {{Lösung versteckt| 1= Runde die berechnete Prozentzahl auf zwei Nachkommastellen genau. |2= Prozentzahl runden |3= }} | ||
{{Lösung versteckt| 1= [[Datei: | {{Lösung versteckt| 1= [[Datei:Lösung-2.jpg|zentriert]]|2= Lösung |3= }} | ||
| 3= Arbeitsmethode | | 3= Arbeitsmethode | ||
| Zeile 263: | Zeile 221: | ||
{{Box | {{Box | ||
| 1= Aufgabe | | 1= Aufgabe 3: Hotelbewertung | ||
| 2= | | 2= | ||
| Zeile 285: | Zeile 243: | ||
! '''Anzahl der Personen''' | ! '''Anzahl der Personen''' | ||
! Anteil | ! Anteil | ||
! '''Prozent''' | ! '''in Prozent''' | ||
{{!-}} | {{!-}} | ||
{{!}} <math>1</math> = "sehr gut" | {{!}} <math>1</math> = "sehr gut" | ||
| Zeile 324: | Zeile 282: | ||
</div> | </div> | ||
{{Lösung versteckt| 1= [[Datei:Lösung | {{Lösung versteckt| 1=[[Datei:Lösung-3a.jpg|zentriert]] |2= Lösung |3= }} | ||
<br /> | <br /> | ||
| Zeile 340: | Zeile 298: | ||
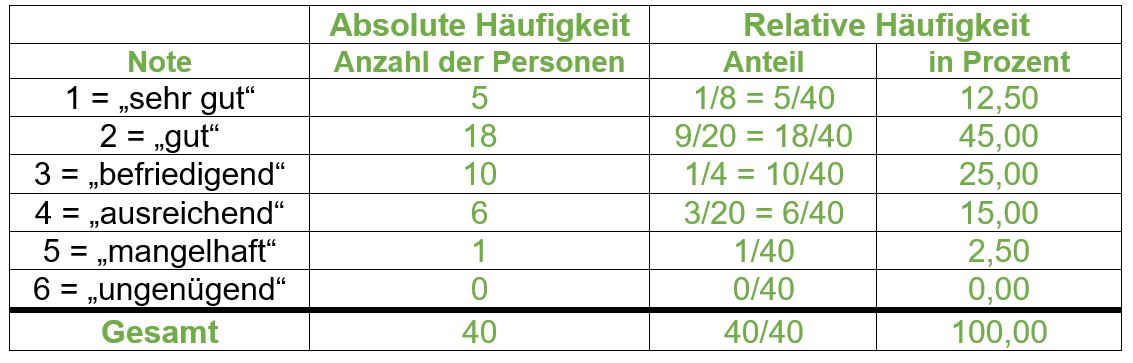
! Anzahl der Personen | ! Anzahl der Personen | ||
! Anteil | ! Anteil | ||
! Prozent | ! in Prozent | ||
{{!-}} | {{!-}} | ||
{{!}} <math>1</math> = "sehr gut" | {{!}} <math>1</math> = "sehr gut" | ||
{{!}} <div class="lueckentext-quiz"> '''5()''' </div> | {{!}} <div class="lueckentext-quiz"> '''5()''' </div> | ||
{{!}} <div class="lueckentext-quiz"> '''5/40()''' </div> | {{!}} <div class="lueckentext-quiz"> '''5/40()''' </div> | ||
{{!}} <div class="lueckentext-quiz"> '''12,50 | {{!}} <div class="lueckentext-quiz"> '''12,50()''' </div> | ||
{{!-}} | {{!-}} | ||
{{!}} <math>2</math> = "gut" | {{!}} <math>2</math> = "gut" | ||
{{!}} <div class="lueckentext-quiz"> '''18()''' </div> | {{!}} <div class="lueckentext-quiz"> '''18()''' </div> | ||
{{!}} <div class="lueckentext-quiz"> '''18/40()''' </div> | {{!}} <div class="lueckentext-quiz"> '''18/40()''' </div> | ||
{{!}} <div class="lueckentext-quiz"> '''45,00 | {{!}} <div class="lueckentext-quiz"> '''45,00()''' </div> | ||
{{!-}} | {{!-}} | ||
{{!}} <math>3</math> = "befriedigend" | {{!}} <math>3</math> = "befriedigend" | ||
{{!}} <div class="lueckentext-quiz"> '''10()''' </div> | {{!}} <div class="lueckentext-quiz"> '''10()''' </div> | ||
{{!}} <div class="lueckentext-quiz"> '''10/40()''' </div> | {{!}} <div class="lueckentext-quiz"> '''10/40()''' </div> | ||
{{!}} <div class="lueckentext-quiz"> '''25,00 | {{!}} <div class="lueckentext-quiz"> '''25,00()''' </div> | ||
{{!-}} | {{!-}} | ||
{{!}} <math>4</math> = "ausreichend" | {{!}} <math>4</math> = "ausreichend" | ||
{{!}} <div class="lueckentext-quiz"> '''6()''' </div> | {{!}} <div class="lueckentext-quiz"> '''6()''' </div> | ||
{{!}} <div class="lueckentext-quiz"> '''6/40()''' </div> | {{!}} <div class="lueckentext-quiz"> '''6/40()''' </div> | ||
{{!}} <div class="lueckentext-quiz"> '''15, | {{!}} <div class="lueckentext-quiz"> '''15,00()''' </div> | ||
{{!-}} | {{!-}} | ||
{{!}} <math>5</math> = "mangelhaft" | {{!}} <math>5</math> = "mangelhaft" | ||
{{!}} <div class="lueckentext-quiz"> '''1()''' </div> | {{!}} <div class="lueckentext-quiz"> '''1()''' </div> | ||
{{!}} <div class="lueckentext-quiz"> '''1/40()''' </div> | {{!}} <div class="lueckentext-quiz"> '''1/40()''' </div> | ||
{{!}} <div class="lueckentext-quiz"> '''2,50 | {{!}} <div class="lueckentext-quiz"> '''2,50()''' </div> | ||
{{!-}} | {{!-}} | ||
{{!}} <math>6</math> = "ungenügend" | {{!}} <math>6</math> = "ungenügend" | ||
{{!}} <div class="lueckentext-quiz"> '''0()''' </div> | {{!}} <div class="lueckentext-quiz"> '''0()''' </div> | ||
{{!}} <div class="lueckentext-quiz"> '''0/40()''' </div> | {{!}} <div class="lueckentext-quiz"> '''0/40()''' </div> | ||
{{!}} < | {{!}} <math>0{,}00</math> | ||
{{!-}} | {{!-}} | ||
! Gesamt | ! Gesamt | ||
{{!}} <div class="lueckentext-quiz"> '''40()''' </div> | {{!}} <div class="lueckentext-quiz"> '''40()''' </div> | ||
{{!}} <div class="lueckentext-quiz"> '''40/40()''' </div> | {{!}} <div class="lueckentext-quiz"> '''40/40()''' </div> | ||
{{!}} < | {{!}} <math>100{,}00</math> | ||
{{!)}} | {{!)}} | ||
| Zeile 383: | Zeile 341: | ||
{{Lösung versteckt| 1= [[Datei:Lösung- | {{Lösung versteckt| 1= [[Datei:Lösung-3b.jpg|zentriert]] |2= Lösung |3= }} | ||
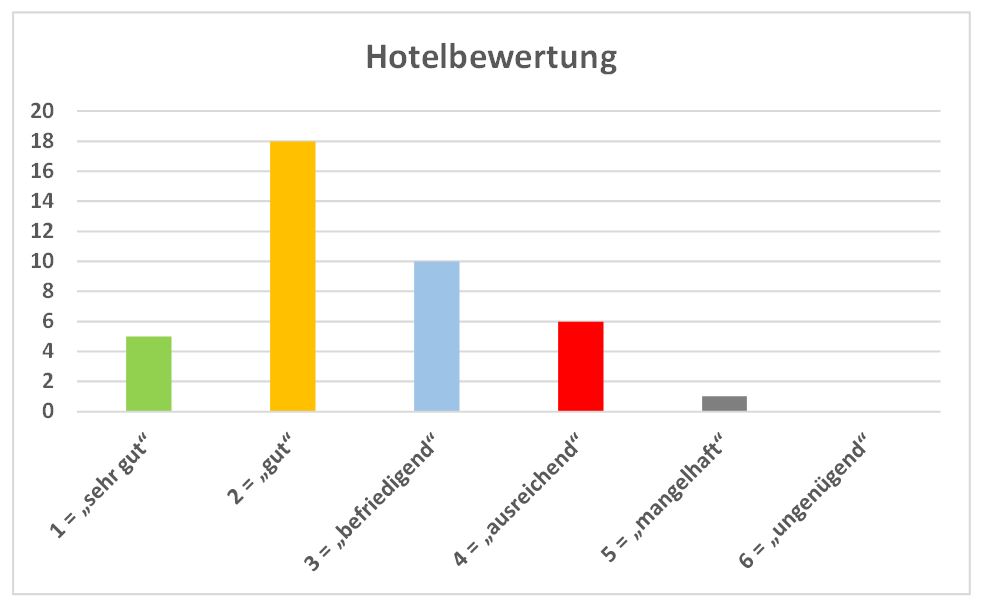
'''c)''' Zeichne ein Säulendiagramm, welches die absoluten Werte der Umfrage darstellt. | '''c)''' Zeichne ein Säulendiagramm, welches die absoluten Werte der Umfrage darstellt. | ||
| Zeile 389: | Zeile 347: | ||
{{Lösung versteckt| 1= [[Datei:Diagramm4-1.jpg|zentriert]] |2= Lösung |3= }} | {{Lösung versteckt| 1= [[Datei:Diagramm4-1.jpg|zentriert]] |2= Lösung |3= }} | ||
| 3= Arbeitsmethode | | 3= Arbeitsmethode | ||
| Farbe={{Farbe|grün|dunkel}} | }} | ||
{{Box | |||
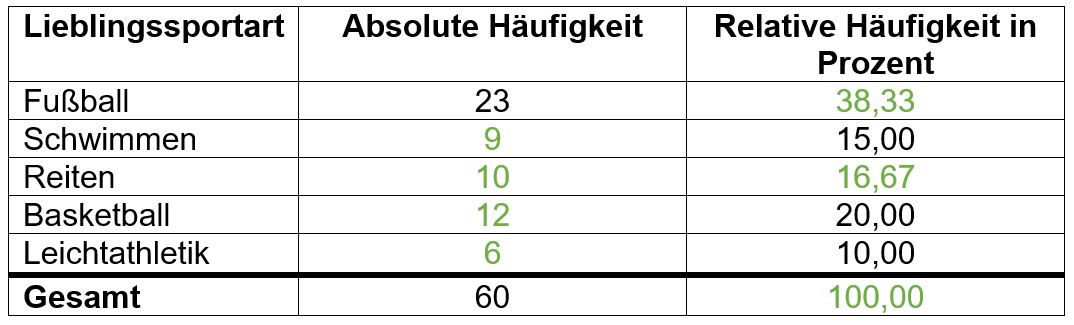
| 1= Aufgabe 4: Lieblingssportart | |||
| 2= Vervollständige die Tabelle: | |||
{{(!}} class="wikitable" | |||
! Lieblingssportart | |||
! Absolute Häufigkeit | |||
! Relative Häufigkeit in Prozent | |||
{{!-}} | |||
{{!}} Fußball | |||
{{!}} <math>23</math> | |||
{{!}} <div class="lueckentext-quiz"> '''38,33()''' </div> | |||
{{!-}} | |||
{{!}} Schwimmen | |||
{{!}} <div class="lueckentext-quiz"> '''9()''' </div> | |||
{{!}} <math>15{,}00</math> | |||
{{!-}} | |||
{{!}} Reiten | |||
{{!}} <div class="lueckentext-quiz"> '''10()''' </div> | |||
{{!}} <div class="lueckentext-quiz"> '''16,67()''' </div> | |||
{{!-}} | |||
{{!}} Basketball | |||
{{!}} <div class="lueckentext-quiz"> '''12()''' </div> | |||
{{!}} <math>20{,}00</math> | |||
{{!-}} | |||
{{!}} Leichtathletik | |||
{{!}} <div class="lueckentext-quiz"> '''6()''' </div> | |||
{{!}} <math>10{,}00</math> | |||
{{!-}} | |||
! Gesamt | |||
{{!}} <math>60</math> | |||
{{!}} <div class="lueckentext-quiz"> '''100,00()''' </div> | |||
{{!)}} | |||
{{Lösung versteckt| 1= Runde die berechnete Prozentzahl auf zwei Nachkommastellen genau. |2= Prozentzahl runden |3= }} | |||
{{Lösung versteckt| 1= [[Datei:Lösung-4.jpg|zentriert]] |2= Lösung |3= }} | |||
| 3= Arbeitsmethode | |||
| Farbe={{Farbe|grün|dunkel}} | |||
}} | }} | ||
| Zeile 614: | Zeile 613: | ||
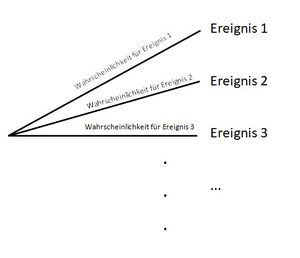
<math>P(\text{Ereignis A} | \text{Ereignis B})= \text{Wahrscheinlichkeit A} \cdot \text{Wahrscheinlichkeit B} </math> | <math>P(\text{Ereignis A} | \text{Ereignis B})= \text{Wahrscheinlichkeit A} \cdot \text{Wahrscheinlichkeit B} </math> | ||
<math>*</math> Diese Schreibweise bedeutet, dass erst | <math>*</math> Diese Schreibweise bedeutet, dass erst Ereignis A und danach Ereignis B eintritt. | ||
| Merksatz}} | | Merksatz}} | ||
| Zeile 701: | Zeile 700: | ||
Für das Ereignis eine Dame zu ziehen gibt es insgesamt <math>4</math> Karten. Also <math>4</math> mögliche Ergebnisse, dessen Wahrscheinlichkeiten nach der Summenregel addiert werden können. | Für das Ereignis eine Dame zu ziehen gibt es insgesamt <math>4</math> Karten. Also <math>4</math> mögliche Ergebnisse, dessen Wahrscheinlichkeiten nach der Summenregel addiert werden können. | ||
<math> P(\text{Dame wird gezogen}) = \tfrac{1}{32} + \tfrac{1}{32} + \tfrac{1}{32} + \tfrac{1}{32} = 4 \cdot \tfrac{1}{32} = \tfrac{4}{32} = \tfrac{1}{8} </math>|2=Lösung a)|3=Lösung}} | <math> P(\text{Dame wird gezogen}) = \tfrac{1}{32} + \tfrac{1}{32} + \tfrac{1}{32} + \tfrac{1}{32} = 4 \cdot \tfrac{1}{32} = \tfrac{4}{32} = \tfrac{1}{8} </math> | ||
Die Wahrscheinlichkeit eine Dame zu ziehen beträgt somit <math>\tfrac{1}{8}</math>. |2=Lösung a)|3=Lösung}} | |||
{{Lösung versteckt|1='''b)''' Es gibt insgesamt <math>8</math> Kreuz-Karten. | {{Lösung versteckt|1='''b)''' Es gibt insgesamt <math>8</math> Kreuz-Karten. | ||
Also gilt mit der Summenregel: | Also gilt mit der Summenregel: | ||
<math>P(\text{Kreuz-Karte wird gezogen})=\tfrac{1}{32}+\tfrac{1}{32}+\tfrac{1}{32}+\tfrac{1}{32}+\tfrac{1}{32}+\tfrac{1}{32}+\tfrac{1}{32}+\tfrac{1}{32}=8\cdot\tfrac{1}{32}=\tfrac{8}{32}=\tfrac{1}{4}</math> |2=Lösung b)|3=Lösung}} | <math>P(\text{Kreuz-Karte wird gezogen})=\tfrac{1}{32}+\tfrac{1}{32}+\tfrac{1}{32}+\tfrac{1}{32}+\tfrac{1}{32}+\tfrac{1}{32}+\tfrac{1}{32}+\tfrac{1}{32}=8\cdot\tfrac{1}{32}=\tfrac{8}{32}=\tfrac{1}{4}</math> | ||
Die Wahrscheinlichkeit eine Kreuz-Karte zu ziehen beträgt somit <math>\tfrac{1}{4}</math>.|2=Lösung b)|3=Lösung}} | |||
{{Lösung versteckt|1='''c)''' Es gibt <math>8</math> Pik und <math>8</math> Kreuz-Karten, also insgesamt <math>16</math> schwarze Karten. | {{Lösung versteckt|1='''c)''' Es gibt <math>8</math> Pik und <math>8</math> Kreuz-Karten, also insgesamt <math>16</math> schwarze Karten. | ||
Also gilt mit der Summenregel: | Also gilt mit der Summenregel: | ||
<math> P(\text{Schwarze Karte wird gezogen})=16\cdot\tfrac{1}{32}=\tfrac{16}{32}=\tfrac{1}{2} </math>|2=Lösung c)|3=Lösung}} | <math> P(\text{Schwarze Karte wird gezogen})=16\cdot\tfrac{1}{32}=\tfrac{16}{32}=\tfrac{1}{2} </math> | ||
Die Wahrscheinlichkeit eine schwarze Karte zu ziehen beträgt somit <math>\tfrac{1}{2}</math>.|2=Lösung c)|3=Lösung}} | |||
| Arbeitsmethode | Farbe={{Farbe|orange}} }} | | Arbeitsmethode | Farbe={{Farbe|orange}} }} | ||
| Zeile 741: | Zeile 746: | ||
Da unter den Steinen nur einmal der Buchstabe D vorhanden ist gilt: | Da unter den Steinen nur einmal der Buchstabe D vorhanden ist gilt: | ||
<math>P(\text{D wird gezogen})=\tfrac{1}{13}</math>. |2=Lösung a)|3=Lösung}} | <math>P(\text{D wird gezogen})=\tfrac{1}{13}</math>. | ||
Die Wahrscheinlichkeit den Buchstaben D zu ziehen beträgt somit <math>\tfrac{1}{13}</math>.|2=Lösung a)|3=Lösung}} | |||
{{Lösung versteckt|1='''b)''' Es gibt zwei Spielsteine mit dem Buchstaben N, die jeweils mit einer Wahrscheinlichkeit von <math>\tfrac{1}{13}</math> gezogen werden. | {{Lösung versteckt|1='''b)''' Es gibt zwei Spielsteine mit dem Buchstaben N, die jeweils mit einer Wahrscheinlichkeit von <math>\tfrac{1}{13}</math> gezogen werden. | ||
| Zeile 747: | Zeile 754: | ||
Wegen der Summenregel für Laplace-Experimente können die Wahrscheinlichkeiten der beiden möglichen Ergebnisse bzw. Spielsteine für das Ereignis addiert werden. | Wegen der Summenregel für Laplace-Experimente können die Wahrscheinlichkeiten der beiden möglichen Ergebnisse bzw. Spielsteine für das Ereignis addiert werden. | ||
Es gilt also: <math>P(\text{N wird gezogen})=\tfrac{1}{13}+\tfrac{1}{13}=\tfrac{2}{13}</math>|2=Lösung b)|3=Lösung}} | Es gilt also: <math>P(\text{N wird gezogen})=\tfrac{1}{13}+\tfrac{1}{13}=\tfrac{2}{13}</math> | ||
Die Wahrscheinlichkeit den Buchstaben N zu ziehen beträgt somit <math>\tfrac{2}{13}</math>.|2=Lösung b)|3=Lösung}} | |||
{{Lösung versteckt|1='''c)''' Es gibt insgesamt <math>3</math> Spielsteine mit dem Buchstaben O, die jeweils mit einer Wahrscheinlichkeit von <math>\tfrac{1}{13}</math> gezogen werden. Wegen der Summenregel für Laplace-Experimente können die Wahrscheinlichkeiten der drei möglichen Ergebnisse bzw. Spielsteine für das Ereignis addiert werden. | {{Lösung versteckt|1='''c)''' Es gibt insgesamt <math>3</math> Spielsteine mit dem Buchstaben O, die jeweils mit einer Wahrscheinlichkeit von <math>\tfrac{1}{13}</math> gezogen werden. Wegen der Summenregel für Laplace-Experimente können die Wahrscheinlichkeiten der drei möglichen Ergebnisse bzw. Spielsteine für das Ereignis addiert werden. | ||
Es gilt also: <math>P(\text{O wird gezogen})=\tfrac{1}{13}+\tfrac{1}{13}+\tfrac{1}{13}= \tfrac{3}{13}</math>|2=Lösung c) |3=Lösung}} | Es gilt also: <math>P(\text{O wird gezogen})=\tfrac{1}{13}+\tfrac{1}{13}+\tfrac{1}{13}= \tfrac{3}{13}</math> | ||
Die Wahrscheinlichkeit den Buchstaben O zu ziehen beträgt somit <math>\tfrac{3}{13}</math>.|2=Lösung c) |3=Lösung}} | |||
{{Lösung versteckt|1='''d)''' Insgesamt gibt es einen Spielstein mit A und drei mit einem O. Die restlichen Vokale sind nicht vorhanden. | {{Lösung versteckt|1='''d)''' Insgesamt gibt es einen Spielstein mit A und drei mit einem O. Die restlichen Vokale sind nicht vorhanden. | ||
Somit folgt mit der Summenregel: | Somit folgt mit der Summenregel: | ||
<math>P(\text{Vokal wird gezogen})=\tfrac{1}{13}+\tfrac{3}{13}=\tfrac{4}{13}</math>|2=Lösung d)|3=Lösung}} | <math>P(\text{Vokal wird gezogen})=\tfrac{1}{13}+\tfrac{3}{13}=\tfrac{4}{13}</math> | ||
Die Wahrscheinlichkeit einen Vokal zu ziehen beträgt somit <math>\tfrac{4}{13}</math>.|2=Lösung d)|3=Lösung}} | |||
| Arbeitsmethode | Farbe={{Farbe|orange}} }} | | Arbeitsmethode | Farbe={{Farbe|orange}} }} | ||
| Zeile 788: | Zeile 801: | ||
{{Lösung versteckt|1= Es gibt <math>3</math> unterschiedliche Kombinationen von Zahlen, deren Differenz <math>3</math> beträgt. Die 4 und 1, die 5 und 2 & die 6 und 3. Die einzelnen Kombinationen können jeweils in zwei unterschiedlichen Reihenfolgen geworfen werden. | {{Lösung versteckt|1= Es gibt <math>3</math> unterschiedliche Kombinationen von Zahlen, deren Differenz <math>3</math> beträgt. Die 4 und 1, die 5 und 2 & die 6 und 3. Die einzelnen Kombinationen können jeweils in zwei unterschiedlichen Reihenfolgen geworfen werden. | ||
Das Ereignis ist also: <math>E = | Das Ereignis ist also: <math>E = \lbrace \lbrace1,4\rbrace; \lbrace4,1\rbrace; \lbrace2,5\rbrace; \lbrace5,2\rbrace; \lbrace3,6\rbrace; \lbrace6,3\rbrace \rbrace</math> | ||
Es gibt somit insgesamt <math>6</math> verschiedene Ergebnisse für das Ereignis. Die einzelnen Ergebnisse haben alle eine Wahrscheinlichkeit von <math>\tfrac{1}{36}</math>, da es mit zwei Würfeln insgesamt <math>36</math> verschiedene Zahlenkombinationen gibt. | Es gibt somit insgesamt <math>6</math> verschiedene Ergebnisse für das Ereignis. Die einzelnen Ergebnisse haben alle eine Wahrscheinlichkeit von <math>\tfrac{1}{36}</math>, da es mit zwei Würfeln insgesamt <math>36</math> verschiedene Zahlenkombinationen gibt. | ||
| Zeile 797: | Zeile 810: | ||
{{Lösung versteckt|1=Die Primzahlen, die mit zwei Würfeln erreicht werden können, sind die <math>2</math>, <math>3</math>, <math>5</math>, <math>7</math>, <math>11</math>. Es gibt <math>8</math> unterschiedliche Kombinationen von Zahlen, deren Summe eine dieser Primzahlen ist. Die 1+1, die 1+2, die 1+4, die 1+6, die 2+3, die 2+5, die 3+4 und die 5+6. Die einzelnen Kombinationen können jeweils in zwei unterschiedlichen Reihenfolgen geworfen werden, außer das 1er-Pasch. | {{Lösung versteckt|1=Die Primzahlen, die mit zwei Würfeln erreicht werden können, sind die <math>2</math>, <math>3</math>, <math>5</math>, <math>7</math>, <math>11</math>. Es gibt <math>8</math> unterschiedliche Kombinationen von Zahlen, deren Summe eine dieser Primzahlen ist. Die 1+1, die 1+2, die 1+4, die 1+6, die 2+3, die 2+5, die 3+4 und die 5+6. Die einzelnen Kombinationen können jeweils in zwei unterschiedlichen Reihenfolgen geworfen werden, außer das 1er-Pasch. | ||
Das Ereignis ist also: <math>E = | Das Ereignis ist also: <math>E = \lbrace \lbrace1,1\rbrace; \lbrace1,2\rbrace; \lbrace2,1\rbrace; \lbrace1,4\rbrace; \lbrace4,1\rbrace; \lbrace1,6\rbrace; \lbrace6,1\rbrace; \lbrace2,3\rbrace; \lbrace3,2\rbrace; \lbrace2,5\rbrace; \lbrace5,2\rbrace; \lbrace3,4\rbrace; \lbrace4,3\rbrace; \lbrace5,6\rbrace; \lbrace6,5\rbrace \rbrace</math> | ||
Es gibt somit insgesamt <math>15</math> verschiedene Ergebnisse für das Ereignis. Die einzelnen Ergebnisse haben alle eine Wahrscheinlichkeit von <math>\tfrac{1}{36}</math>, da es mit zwei Würfeln insgesamt <math>36</math> verschiedene Zahlenkombinationen gibt. | Es gibt somit insgesamt <math>15</math> verschiedene Ergebnisse für das Ereignis. Die einzelnen Ergebnisse haben alle eine Wahrscheinlichkeit von <math>\tfrac{1}{36}</math>, da es mit zwei Würfeln insgesamt <math>36</math> verschiedene Zahlenkombinationen gibt. | ||
Aktuelle Version vom 14. Dezember 2020, 20:19 Uhr
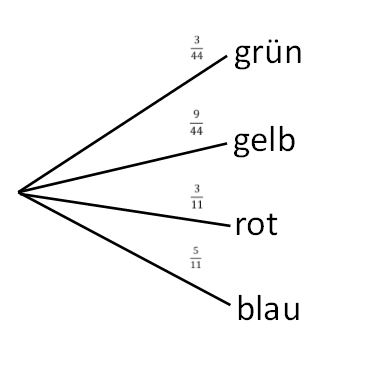
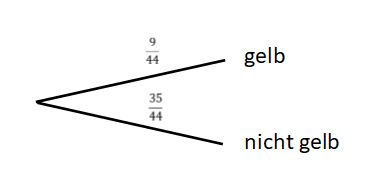
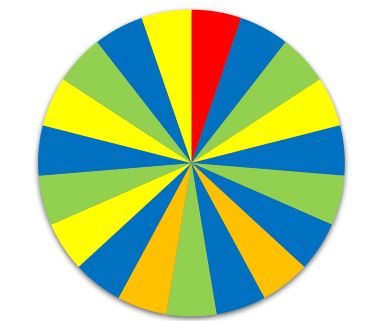
Absolute und relative Häufigkeit
Zufallsexperimente
Laplace-Experimente