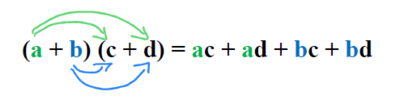Digitale Werkzeuge in der Schule/Fit für VERA-8/Terme: Unterschied zwischen den Versionen
Aus ZUM Projektwiki
Keine Bearbeitungszusammenfassung Markierung: 2017-Quelltext-Bearbeitung |
Markierung: 2017-Quelltext-Bearbeitung |
||
| (199 dazwischenliegende Versionen von 4 Benutzern werden nicht angezeigt) | |||
| Zeile 1: | Zeile 1: | ||
{{Box|1=Info |2=In diesem Lernpfadkapitel lernst du Grundlagen über Terme und binomische Formeln kennen. | {{Box|1=Info |2=In diesem Lernpfadkapitel lernst du Grundlagen über Terme und binomische Formeln kennen. Im ersten Teil geht es darum, Terme zusammenzufassen. Danach wiederholst du das Ausmultiplizieren und Faktorisieren und im letzten Teil die binomischen Formeln. Lege dir für die Aufgaben Zettel und Stifte bereit. Bei den Aufgaben unterscheiden wir folgende Typen: | ||
* In Aufgaben, die '''<span style="color: #F19E4F">orange</span>''' gefärbt sind, kannst du '''grundlegende Kompetenzen''' wiederholen und vertiefen. | * In Aufgaben, die '''<span style="color: #F19E4F">orange</span>''' gefärbt sind, kannst du '''grundlegende Kompetenzen''' wiederholen und vertiefen. | ||
* Aufgaben in '''<span style="color: #5E43A5">blauer</span>''' Farbe sind '''Aufgaben mittlerer Schwierigkeit'''. | * Aufgaben in '''<span style="color: #5E43A5">blauer</span>''' Farbe sind '''Aufgaben mittlerer Schwierigkeit'''. | ||
| Zeile 6: | Zeile 6: | ||
===1) Terme zusammenfassen=== | ===1) Terme zusammenfassen=== | ||
====Einführung==== | ====Einführung==== | ||
{{Box |1= Rechenregeln | 2=Terme enthalten unterschiedliche Rechenoperationen wie Addition, Subtraktion, Multiplikation und Division. Manche Teile von Termen kann man zusammenfassen, um so den Term zu vereinfachen. Beachte dabei: <br/> <br/> | |||
{{Box |1= Rechenregeln | 2=Terme | |||
'''Beim Zusammenfassen von Summen gilt:''' <br/> Nur gleiche Variablen in der gleichen Potenz dürfen zusammengefasst werden. <br/> | '''Beim Zusammenfassen von Summen gilt:''' <br/> Nur gleiche Variablen in der gleichen Potenz dürfen zusammengefasst werden. <br/> | ||
Beispiele: <br/> | Beispiele: <br/> | ||
<math> | 1) <math> {\color{blue}2a^2}{\color{red}+a+3a} </math> <br/> | ||
<math> = 2a^2+4a</math> <br/> <br/> | <math> = {\color{blue}2a^2}{\color{red}+4a}</math> <br/> <br/> | ||
<math> 2x+xy-3y^2-2xy+2xy^2 </math> <br/> | 2) <math> {\color{orange}2x}{\color{red}+xy}{\color{blue}-3y^2}{\color{red}-2xy}{\color{green}+2xy^2} </math> <br/> | ||
<math>= 2x-3y^2+2xy^2+ | <math>= {\color{orange}2x}{\color{blue}-3y^2}{\color{green}+2xy^2}{\color{red}+xy-2xy} </math> <br/> | ||
<math>= 2x-3y^2+2xy^2-xy </math> <br/> | <math>= {\color{orange}2x}{\color{blue}-3y^2}{\color{green}+2xy^2}{\color{red}-xy} </math> <br/> | ||
Hier konnten nur die beiden Teile mit xy zusammengefasst werden, da alle anderen Variablen unterschiedlich sind bzw. in einer anderen Potenz vorkommen. <br/> <br/> | Hier konnten nur die beiden Teile mit <math>{\color{red}xy}</math> zusammengefasst werden, da alle anderen Variablen unterschiedlich sind bzw. in einer anderen Potenz vorkommen. <br/> <br/> | ||
<math> {\color{blue}2x}{\color{red}+4y}{\color{green}-xy}{\color{red}+2y}{\color{blue}-3x}{\color{green}+5xy}</math> <br/> | 3) <math> {\color{blue}2x}{\color{red}+4y}{\color{green}-xy}{\color{red}+2y}{\color{blue}-3x}{\color{green}+5xy}</math> <br/> | ||
<math> = {\color{blue}-x}{\color{red}+6y}{\color{green}+4xy} </math> <br/> | <math> = {\color{blue}-x}{\color{red}+6y}{\color{green}+4xy} </math> <br/> | ||
Tipp: Es kann helfen die gleichen Potenzen und Variablen farblich zu markieren. | Tipp: Es kann helfen die gleichen Potenzen und Variablen farblich zu markieren. <br/> <br/> | ||
| Zeile 24: | Zeile 23: | ||
Es können auch Teile mit unterschiedlichen Potenzen oder Variablen zusammengefasst werden. <br/> | Es können auch Teile mit unterschiedlichen Potenzen oder Variablen zusammengefasst werden. <br/> | ||
Beispiel: <br/> | Beispiel: <br/> | ||
<math> | 4) <math> 2{\color{red}x} \cdot 4{\color{red}x}{\color{blue}y} </math> <br/> | ||
<math> = 2 \cdot x \cdot 4 \cdot x \cdot y </math> <br/> | <math> = 2 \cdot {\color{red}x} \cdot 4 \cdot {\color{red}x} \cdot {\color{blue}y} </math> <br/> | ||
<math> = 2 \cdot 4 \cdot x \cdot x \cdot y </math> <br/> | <math> = 2 \cdot 4 \cdot {\color{red}x} \cdot {\color{red}x} \cdot {\color{blue}y} </math> <br/> | ||
<math> = 8 \cdot x^2 \cdot y </math> <br/> | <math> = 8 \cdot {\color{red}x^2} \cdot {\color{blue}y} </math> <br/> | ||
<math> = | <math> = 8{\color{red}x^2}{\color{blue}y} </math> <br/> | ||
Beachte die Vorzeichen der Faktoren. <br/> | Beachte die Vorzeichen der Faktoren. <br/> | ||
{{Lösung versteckt| 1= <math> (-x) + (-x) = -2x </math> <br/> | {{Lösung versteckt| 1= <math> (-x) + (-x) = -2x </math> <br/> | ||
<math> (-x) \cdot (-y) = x \cdot y </math> <br/> <br/> | <math> (-x) \cdot (-y) = x \cdot y </math> <br/> <br/> | ||
'''Beachte außerdem: Punkt- vor Strichrechnung, die Klammer geht immer vor.''' |2=Erinnerung |3=Erinnerung ausblenden}} | '''Beachte außerdem: Punkt- vor Strichrechnung, die Klammer geht immer vor.''' |2=Erinnerung |3=Erinnerung ausblenden}} | ||
Beispiel: <br/> | Beispiel: <br/> | ||
<math> | 5) <math> 2{\color{red}x} \cdot (-7{\color{red}x^2}{\color{blue}y}) \cdot (-3{\color{blue}y^3}) </math> <br/> | ||
<math> = 2 \cdot (-7) \cdot (-3) \cdot x \cdot x^2 \cdot y \cdot y^3 </math> <br/> | <math> = 2 \cdot (-7) \cdot (-3) \cdot {\color{red}x} \cdot {\color{red}x^2} \cdot {\color{blue}y} \cdot {\color{blue}y^3} </math> <br/> | ||
<math> = | <math> = 42{\color{red}x^3}{\color{blue}y^4} </math> | ||
|3=Merksatz}} | |3=Merksatz}} | ||
===Aufgaben=== | |||
=== | {{Box | Aufgabe 1: Wer wird Millionär? | | ||
{{LearningApp|width=100%|height=500px|app=pg7bfsfba20}} | |||
{{Lösung versteckt| 1= Wenn du dir bei manchen Antworten unsicher bist, schau noch einmal [[#Einführung|oben]] in den Rechenregeln nach. | 2= Tipp| 3=Tipp einklappen}}| Arbeitsmethode| Farbe={{Farbe|orange}}}} | |||
a) <math> 15 \cdot 2+3 \cdot 5 \cdot 2+4 </math> | {{Box | 1=Aufgabe 2: Rechenaufgaben | 2= Fasse die folgenden Terme zusammen. Nutze dazu deinen Zettel und Stift, um die Rechenwege und Lösungen aufzuschreiben. <br/> | ||
'''a)''' <math> 15 \cdot 2+3 \cdot 5 \cdot 2+4 </math> | |||
{{Lösung versteckt| 1= Denke an die Rechenregel "Punkt vor Strich". | 2= Tipp| 3=Tipp einklappen}} | |||
{{Lösung versteckt| 1= <math> 64 </math> | 2= Lösung | 3=Lösung einklappen}} | {{Lösung versteckt| 1= <math> 64 </math> | 2= Lösung | 3=Lösung einklappen}} | ||
| Zeile 59: | Zeile 62: | ||
b) <math> 3x-4y-2z+4y-2x</math> <br/> | '''b)''' <math> 3x-4y-2z+4y-2x</math> <br/> | ||
{{Lösung versteckt| 1= Sortiere zuerst die Variablen, fasse dann gleiche Variablen zusammen. Farbliche Markierungen wie in den [[#Einführung|Beispielen]] können dir helfen. | 2=Tipp | 3=Tipp einklappen}} | |||
{{Lösung versteckt| 1= <math> x+8y-2z </math> | 2= Lösung | 3=Lösung einklappen}} | {{Lösung versteckt| 1= <math> x+8y-2z </math> | 2= Lösung | 3=Lösung einklappen}} | ||
{{Lösung versteckt| 1= <math> 3x-4y-2z+4y-2x</math> <br/> | {{Lösung versteckt| 1= <math> {\color{red}3x}{\color{blue}-4y}{\color{green}-2z}{\color{blue}+4y}{\color{red}-2x}</math> <br/> | ||
<math> = 3x-2x | <math> = {\color{red}3x-2x}{\color{blue}-4y+4y}{\color{green}-2z} </math> <br/> | ||
<math> = x | <math> = {\color{red}x}{\color{green}-2z} </math> | 2= Lösungsweg | 3=Lösung einklappen}} <br/> <br/> | ||
'''c)''' <math> x \cdot 2 \cdot y+3x-y \cdot x </math> | |||
{{Lösung versteckt| 1= Sortiere zuerst die Variablen, fasse dann gleiche Variablen zusammen. Farbliche Markierungen wie in den Beispielen können dir helfen. | 2=Tipp | 3=Tipp einklappen}} | |||
{{Lösung versteckt| 1= <math> xy+3x </math> | 2= Lösung | 3=Lösung einklappen}} | {{Lösung versteckt| 1= <math> xy+3x </math> | 2= Lösung | 3=Lösung einklappen}} | ||
{{Lösung versteckt| 1= <math> x \cdot 2 \cdot y+ | {{Lösung versteckt| 1= <math> {\color{red}x} \cdot 2 \cdot {\color{blue}y}+3{\color{red}x}-{\color{blue}y} \cdot {\color{red}x} </math> <br/> | ||
<math> = | <math> = 2{\color{red}x}{\color{blue}y}-{\color{red}x}{\color{blue}y}+3{\color{red}x} </math> <br/> | ||
<math> = | <math> = {\color{red}x}{\color{blue}y}+3{\color{red}x} </math> | 2= Lösungsweg | 3=Lösung einklappen}} <br/> <br/> | ||
d) <math> x \cdot y+x\cdot x+2 \cdot y+3 \cdot x \cdot 5 \cdot y </math> <br/> | '''d)''' <math> x \cdot y+x\cdot x+2 \cdot y+3 \cdot x \cdot 5 \cdot y </math> <br/> | ||
{{Lösung versteckt| 1= Sortiere zuerst die Variablen, fasse dann gleiche Variablen zusammen. Denke dabei an die Regel "Punkt vor Strich". Farbliche Markierungen wie in den Beispielen können dir helfen. | 2=Tipp | 3=Tipp einklappen}} | |||
{{Lösung versteckt| 1= <math> 16xy+x^2+2y </math> | 2= Lösung | 3=Lösung einklappen}} | {{Lösung versteckt| 1= <math> 16xy+x^2+2y </math> | 2= Lösung | 3=Lösung einklappen}} | ||
{{Lösung versteckt| 1= <math> x \cdot y+x\cdot x+2 \cdot y+3 \cdot x \cdot 5 \cdot y </math> <br/> | {{Lösung versteckt| 1= <math> {\color{red}x} \cdot {\color{blue}y}+{\color{red}x} \cdot {\color{red}x}+2 \cdot {\color{blue}y}+3 \cdot {\color{red}x} \cdot 5 \cdot {\color{blue}y} </math> <br/> | ||
<math> = | <math> = {\color{red}x}{\color{blue}y}+{\color{red}x^2}+2{\color{blue}y}+15{\color{red}x}{\color{blue}y} </math> <br/> | ||
<math> = | <math> = 16{\color{red}x}{\color{blue}y}+{\color{red}x^2}+2{\color{blue}y} </math> | 2= Lösungsweg | 3=Lösung einklappen}} <br/> <br/> | ||
e) <math> 2x+3xy-y+4x-2yx+ | '''e)''' <math> 2x+3xy-y+4x-2yx+y </math> | ||
{{Lösung versteckt| 1= Sortiere zuerst die Variablen, fasse dann gleiche Variablen zusammen. Farbliche Markierungen wie in den Beispielen können dir helfen. | 2=Tipp | 3=Tipp einklappen}} | |||
{{Lösung versteckt| 1= <math> 6x+xy </math> | 2= Lösung | 3=Lösung einklappen}} | {{Lösung versteckt| 1= <math> 6x+xy </math> | 2= Lösung | 3=Lösung einklappen}} | ||
{{Lösung versteckt| 1= <math> | {{Lösung versteckt| 1= <math> 2{\color{red}x}+3{\color{red}x}{\color{blue}y}-{\color{blue}y}+4{\color{red}x}-2{\color{blue}y}{\color{red}x}+{\color{blue}y} </math> <br/> | ||
<math> = | <math> = 2{\color{red}x}+4{\color{red}x}-{\color{blue}y}+{\color{blue}y}+3{\color{red}x}{\color{blue}y}-2{\color{red}x}{\color{blue}y} </math> <br/> | ||
<math> = | <math> = 6{\color{red}x}+{\color{red}x}{\color{blue}y} </math> | 2= Lösungsweg | 3=Lösung einklappen}} | ||
f) <math> 12x-(12x+3y)+4y-(3x+2y) </math> <br/> | '''f)''' <math> 12x-(12x+3y)+4y-(3x+2y) </math> <br/> | ||
{{Lösung versteckt| 1= Löse zuerst die Klammern auf, sortiere dann die Variablen und fasse gleiche Variablen zusammen. Farbliche Markierungen wie in den Beispielen können dir helfen. | 2=Tipp 1 | 3=Tipp einklappen}} | |||
{{Lösung versteckt| 1= Vergiss nicht: Punkt- vor Strichrechnung. Die Klammer geht immer vor. | 2=Tipp 2 | 3=Tipp einklappen}} | |||
{{Lösung versteckt| 1= <math> -3x-y </math> | 2= Lösung | 3=Lösung einklappen}} | {{Lösung versteckt| 1= <math> -3x-y </math> | 2= Lösung | 3=Lösung einklappen}} | ||
{{Lösung versteckt| 1= <math> 12x-(12x+3y)+4y-(3x+2y) </math> <br/> | {{Lösung versteckt| 1= <math> {\color{red}12x}{\color{green}-}({\color{red}12x}{\color{blue}+3y}){\color{blue}+4y}{\color{green}-}({\color{red}3x}{\color{blue}+2y}) </math> <br/> | ||
<math> = 12x-12x-3y+4y-3x-2y </math> <br/> | <math> = {\color{red}12x-12x}{\color{blue}-3y+4y}{\color{red}-3x}{\color{blue}-2y} </math> <br/> | ||
<math> = 12x-12x-3x-3y+4y-2y </math> <br/> | <math> = {\color{red}12x-12x-3x}{\color{blue}-3y+4y-2y} </math> <br/> | ||
<math> = -3x-y </math> | 2= Lösungsweg | 3=Lösung einklappen}} <br/> <br/> | <math> = {\color{red}-3x}{\color{blue}-y} </math> | 2= Lösungsweg | 3=Lösung einklappen}} <br/> <br/> | ||
g) <math> 4x-({1\over 4}y-(5x+3z)-(x+{5\over 8}y-2z)) </math> | '''g)''' <math> 4x-\left({1\over 4}y-\left(5x+3z\right)-\left(x+{5\over 8}y-2z\right)\right) </math> | ||
{{Lösung versteckt|1= | {{Lösung versteckt|1=Löse zuerst die Klammern auf, ordne dann die Summanden nach ihren Variablen. Mache danach noch die Brüche gleichnamig um alles zusammenfassen zu können.|2=Tipp|3=Tipp ausblenden}} | ||
{{Lösung versteckt|1= <math>10x+{3\over 8}y+z </math>|2=Lösung|3=Lösung ausblenden}} | {{Lösung versteckt|1= <math>10x+{3\over 8}y+z </math>|2=Lösung|3=Lösung ausblenden}} | ||
{{Lösung versteckt|1= <math> 4x-({1\over 4}y-(5x+3z)-(x+{5\over 8}y-2z)) </math> <br/> | {{Lösung versteckt|1= <math> {\color{red}4x}{\color{orange}-}\left({\color{blue}{1\over 4}y}{\color{orange}-}\left({\color{red}5x}{\color{green}+3z}\right){\color{orange}-}\left({\color{red}x}+{\color{blue}{5\over 8}y}{\color{green}-2z}\right)\right) </math> <br/> | ||
<math> = 4x-({1\over 4}y-5x-3z | <math> = {\color{red}4x}{\color{orange}-}\left({\color{blue}{1\over 4}y}{\color{red}-5x}{\color{green}-3z}{\color{red}-x}{\color{blue}-{5\over 8}y}{\color{green}+2z}\right) </math> <br/> | ||
<math> = 4x-{1\over 4}y+5x+3z+x+{5\over 8}y-2z </math> <br/> | <math> = {\color{red}4x}{\color{blue}-{1\over 4}y}{\color{red}+5x}{\color{green}+3z}{\color{red}+x}{\color{blue}+{5\over 8}y}{\color{green}-2z} </math> <br/> | ||
<math> = 4x+5x+x-{1\over 4}y+{5\over 8}y+3z-2z </math> <br/> | <math> = {\color{red}4x+5x+x}{\color{blue}-{1\over 4}y+{5\over 8}y}{\color{green}+3z-2z} </math> <br/> | ||
<math> = 4x+5x+x-{2\over 8}y+{5\over 8}y+3z-2z </math> <br/> | <math> = {\color{red}4x+5x+x}{\color{blue}-{2\over 8}y+{5\over 8}y}{\color{green}+3z-2z} </math> <br/> | ||
<math> = 10x+{3\over 8}y+z </math> |2=Lösungsweg|3=Lösungsweg einklappen}} <br/> <br/> | <math> = {\color{red}10x}{\color{blue}+{3\over 8}y}{\color{green}+z} </math> |2=Lösungsweg|3=Lösungsweg einklappen}} <br/> <br/> | ||
| 3=Arbeitsmethode }} | | 3=Arbeitsmethode }} | ||
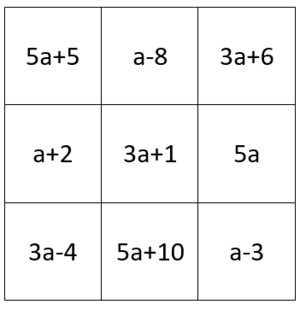
{{Box| 1=Aufgabe 3: | {{Box| 1= Aufgabe 3: Magisches Rechteck| 2= Die Summen jeder Zeile, Spalte und Diagonale des magischen Rechtecks ergeben gleichwertige Terme, das heißt wenn du eine Zeile addierst, kommt das gleiche raus wie bei allen anderen Zeilen, Spalten und Diagonalen. Ergänze die fehlenden Terme. Du kannst sie direkt unten eintragen und deine Antwort überprüfen. <br/> | ||
{{Lösung versteckt|1= Berechne zuerst die Summe der ersten Spalte. Diese Summe muss auch die Summe aller weiteren Zeilen, Spalten und Diagonalen sein | 2=Tipp | {{(!}} class="wikitable" | ||
{{!-}} | |||
{{!}} ''' 5a+5''' | |||
{{!}} <div class="lueckentext-quiz"> '''a-8()''' </div> | |||
{{!}} <div class="lueckentext-quiz"> '''3a+6()''' </div> | |||
{{!-}} | |||
{{!}} '''a+2''' | |||
{{!}} '''3a+1''' | |||
{{!}} <div class="lueckentext-quiz"> '''5a()''' </div> | |||
{{!-}} | |||
{{!}} '''3a-4''' | |||
{{!}} <div class="lueckentext-quiz"> '''5a+10()''' </div> | |||
{{!}} <div class="lueckentext-quiz"> '''a-3()''' </div> | |||
{{!)}} | |||
{{Lösung versteckt|1= Berechne zuerst die Summe der ersten Spalte. Diese Summe muss auch die Summe aller weiteren Zeilen, Spalten und Diagonalen sein. | 2=Tipp 1 | 3=Tipp ausblenden}} | |||
{{Lösung versteckt|1=Wenn du die Summe der ersten Spalte berechnet hast, kannst du als nächstes die Summe der zweiten Zeile berechnen und in das noch auszufüllende Kästchen der zweiten Zeile den Term eintragen, der in der Summe noch fehlt, damit die Summe der ersten Spalt gleich der Summe der zweiten Zeile ist. | 2=Tipp 2 |3= Tipp ausblenden }} | {{Lösung versteckt|1=Wenn du die Summe der ersten Spalte berechnet hast, kannst du als nächstes die Summe der zweiten Zeile berechnen und in das noch auszufüllende Kästchen der zweiten Zeile den Term eintragen, der in der Summe noch fehlt, damit die Summe der ersten Spalt gleich der Summe der zweiten Zeile ist. | 2=Tipp 2 |3= Tipp ausblenden }} | ||
{{Lösung versteckt|1= [[Datei:Magisches Dreieck ausgefüllt.png|mini|center]] | {{Lösung versteckt|1= [[Datei:Magisches Dreieck ausgefüllt.png|mini|center]] | ||
|2=Lösung|3=Lösung ausblenden}}|Farbe={{Farbe|grün}} }} | |2=Lösung|3=Lösung ausblenden}} | ||
| 3= Arbeitsmethode | Farbe={{Farbe|grün|dunkel}} }} | |||
===2) Terme ausmultiplizieren und faktorisieren=== | ===2) Terme ausmultiplizieren und faktorisieren=== | ||
====Terme ausmultiplizieren==== | ====Terme ausmultiplizieren==== | ||
{{Box | 1= | {{Box | 1=Rechenregeln | 2=Das ''Ausmultiplizieren'' hat zum Ziel, eine Klammer aufzulösen. Man multipliziert einen <span style="color: green">'''Faktor'''</span> mit einer Klammer, indem man '''den Faktor mit jedem einzelnen Glied in der Klammer multipliziert.''' So wird z.B. der Faktor <math>{\color{green}2}</math> mit jedem Glied aus der Klammer <math>(5 + 3)</math> multipliziert: <br /> | ||
[[Datei:Ausmultiplizieren 1.png|400px | links | thumb ]] <br /> <br /> <br /> <br /> <br /> <br /> | [[Datei:Ausmultiplizieren 1.png|400px | links | thumb ]] <br /> <br /> <br /> <br /> <br /> <br /> | ||
Es spielt keine Rolle, ob der Faktor links oder rechts von der Klammer steht: | Dies nennt man '''Distributivgesetz'''. Es spielt keine Rolle, ob der Faktor links oder rechts von der Klammer steht: | ||
<math>(5+3){\color{green}2} = 5 \cdot {\color{green}2} + 3\cdot {\color{green}2} = 10 + 6 = 16</math>. | <math>(5+3) \cdot {\color{green}2} = 5 \cdot {\color{green}2} + 3\cdot {\color{green}2} = 10 + 6 = 16</math>. | ||
Achte darauf, ob in der Klammer eine Summe oder Differenz steht, denn: | Achte darauf, ob in der Klammer eine Summe oder Differenz steht, denn: | ||
| Zeile 149: | Zeile 188: | ||
<math>{\color{green}a}(b+c) = {\color{green}a}b + {\color{green}a}c</math>. | <math>{\color{green}a}(b+c) = {\color{green}a}b + {\color{green}a}c</math>. | ||
Das kann man sich auch anhand von Flächen mit den Seitenlängen a, b und c veranschaulichen: | Das '''Distributivgesetz''' kann man sich auch anhand von Flächen mit den Seitenlängen a, b und c veranschaulichen: | ||
[[File:Illustration of distributive property with rectangles.svg| 500px | links | thumb]] <br /> <br /> <br /> <br /> <br /> <br /> <br /> <br /> | [[File:Illustration of distributive property with rectangles.svg| 500px | links | thumb]] <br /> <br /> <br /> <br /> <br /> <br /> <br /> <br /> | ||
Bei Minusklammern, also wenn vor der Klammer ein '''negativer Faktor''' steht, drehen sich die Vorzeichen von jedem Glied in der Klammer um: | |||
<math>{\color{red}-}a(b{\color{red}+}c) = {\color{red}-}ab {\color{red}-} ac</math>. | <math>{\color{red}-}a(b{\color{red}+}c) = {\color{red}-}ab {\color{red}-} ac</math>. | ||
| Zeile 178: | Zeile 217: | ||
<math>{\color{red}-}(a{\color{red}-}b) = {\color{red}-}a {\color{red}+} b</math>. | <math>{\color{red}-}(a{\color{red}-}b) = {\color{red}-}a {\color{red}+} b</math>. | ||
|2=Bei Bedarf findest du hier weitere Beispiele zum Thema | |2=Bei Bedarf findest du hier weitere Beispiele zum Thema Ausmultiplizieren |3=Verbergen}} | ||
| 3=Merksatz}} | | 3=Merksatz}} | ||
| Zeile 184: | Zeile 223: | ||
=====Aufgabe===== | =====Aufgabe===== | ||
{{Box | 1= Aufgabe 1 | 2= In dieser Aufgabe kannst du | {{Box | 1= Aufgabe 1: Zuordnen | 2= In dieser Aufgabe kannst du das ''Ausmultiplizieren'' üben. Ordne jedem Klammerterm die richtige ausmultiplizierte Lösung zu. Nimm dir einen Zettel für Nebenrechnungen zur Hilfe. | ||
{{Lösung versteckt|1=Schaue dir bei Schwierigkeiten nochmal die Beispiele aus dem Kapitel [[#Terme ausmultiplizieren|Terme ausmultiplizieren]] an. | |||
|2=Tipp|3=Verbergen}} | |||
<div class="lueckentext-quiz"> | |||
a) <math> | a) <math> (5b+c+3d)\cdot a = </math> '''<math> 5ab+ac+3ad </math>''' <br /> | ||
b) <math> | b) <math> (5a+4b)\cdot 4 = </math> '''<math> 16b+20a </math>''' <br /> | ||
c) <math> -8(a | c) <math> -8(a-2b) = </math> '''<math> 16b-8a </math>''' <br /> | ||
d) <math> | d) <math> 5a(b+\frac{1}{5}c+3d) = </math> '''<math> 5ab+ac+15ad </math>''' <br /> | ||
e) <math> (5a+10b)(\frac{1}{5}c+2d) = </math> '''<math> ac+10ad+2bc+20bd </math>''' <br /> | e) <math> (5a+10b)(\frac{1}{5}c+2d) = </math> '''<math> ac+10ad+2bc+20bd </math>''' <br /> | ||
f) <math> \frac{1}{2}(\frac{1}{2}a+\frac{1}{ | f) <math> -\frac{1}{2}(\frac{1}{2}a+\frac{1}{2}b) = </math> '''<math> -\frac{1}{4}a-\frac{1}{4}b </math>''' <br /> | ||
g) <math> -\frac{1}{4}(a | g) <math> -\frac{1}{4}(a-b) </math> = '''<math> -\frac{1}{4}a+\frac{1}{4}b </math>''' <br /> | ||
h) <math> (\frac{1}{2}a+\frac{1}{2}b)(2c+ | h) <math> (\frac{1}{2}a+\frac{1}{2}b)(2c+20d) </math> = '''<math> ac+10ad+bc+10bd </math>''' | ||
</div> | </div> | ||
| Zeile 200: | Zeile 244: | ||
====Terme faktorisieren==== | ====Terme faktorisieren==== | ||
{{Box | 1= | {{Box | 1=Rechenregeln | 2=Beim ''Faktorisieren'' (auch genannt: ''Ausklammern'') geht es '''genau umgekehrt wie beim ''Ausmultiplizieren'' darum, etwas in einer Klammer zusammenzufassen.''' Wie das funktioniert, erklärt dir Lehrer Schmidt in folgendem Video: <br \> <br \> <div align="center">{{#ev:youtube|425_2PDl4b4}}</div> | ||
{{Lösung versteckt|1= <math> {\color{green}2}a+{\color{green}2}b = {\color{green}2}(a+b) </math> <br /> | {{Lösung versteckt|1= <math> {\color{green}2}a+{\color{green}2}b = {\color{green}2}(a+b) </math> <br /> | ||
<math> {\color{green}4}x+{\color{green}4}y = {\color{green}4}(x+y) </math> <br /> | <math> {\color{green}4}x+{\color{green}4}y = {\color{green}4}(x+y) </math> <br /> | ||
<math> 16x+4y = {\color{green}4} \cdot 4x + {\color{green}4}y = {\color{green}4}(4x+y) </math> <br /> | <math> 16x+4y = {\color{green}4} \cdot 4x + {\color{green}4}y = {\color{green}4}(4x+y) </math> <br /> | ||
<math> 6a+3b+12c= {\color{green}3} \cdot 2a + {\color{green}3}b + {\color{green}3} \cdot 4c = {\color{green}3}(2a+b+4c) </math> | <math> 6a+3b+12c= {\color{green}3} \cdot 2a + {\color{green}3}b + {\color{green}3} \cdot 4c = {\color{green}3}(2a+b+4c) </math> | ||
|2=Zum Nachschauen findet ihr hier nochmal die Beispiele aus dem Video|3=Verbergen}} | |2=Zum Nachschauen findet ihr hier nochmal die Beispiele aus dem Video|3=Verbergen}} | ||
Um zu überprüfen, ob du richtig faktorisiert hast, kannst du eine Probe durchführen, indem du deinen faktorisierten Term ausmultiplizierst und schaust, ob der Ursprungsterm herauskommt. | Um zu überprüfen, ob du richtig faktorisiert hast, kannst du eine Probe durchführen, indem du deinen faktorisierten Term ausmultiplizierst und schaust, ob der Ursprungsterm herauskommt. <br /> | ||
{{Lösung versteckt|1= Du faktorisierst: <br /> | |||
<math> 9xy - 6x = {\color{green}3x} \cdot 3y - {\color{green}3x} \cdot 2 = {\color{green}3x} (3y - 2) </math> <br /> | |||
Nun prüfst du dein Ergebnis, indem du das Ergebnis ausmultiplizierst: <br /> | |||
<math> 3x \cdot (3y - 2) = 9xy - 6x </math> <br /> | |||
Da der Ursprungsterm herauskommt, stimmt dein Ergebnis. | |||
|2=Beispiel zur Probe|3=Verbergen}} | |||
| 3=Merksatz}} | | 3=Merksatz}} | ||
=====Aufgabe===== | =====Aufgabe===== | ||
{{Box | 1= Aufgabe 2 | 2= | {{Box | 1= Aufgabe 2: Wähle die richtige Antwort | 2= '''a)''' Was lässt sich sinnvollerweise ausklammern? | ||
a) Was lässt sich sinnvollerweise ausklammern? | {{Lösung versteckt|1=Schaue dir (nochmal) die [[#Terme faktorisieren|Beispiele]] aus dem Video von Lehrer Schmidt an. | ||
|2=Tipp|3=Verbergen}} | |||
<br \> | <br \> | ||
<div class="multiplechoice-quiz"> | <div class="multiplechoice-quiz"> | ||
(i) <math> 9x - 15 </math> (!5) (3) (!9) (!x) | '''(i)''' <math> 9x - 15 </math> (!<math> 5 </math>) (<math> 3 </math>) (!<math> 9 </math>) (!<math> x</math>) <math> </math> | ||
(ii) <math> -36 + 12x </math> (!9) (12) (!24) (!x) | '''(ii)''' <math> -36 + 12x </math> (!<math> 9 </math>) (<math> 12 </math>) (!<math> 24 </math>) (!<math> x</math>) | ||
(iii) <math> | '''(iii)''' <math> 5xy + 4xz + 3x </math> (!<math> 5 </math>) (<math> x </math>) (!<math> y </math>) (!<math> 2z </math>) | ||
</div> | </div> | ||
b) Wie sieht der erste Zwischenschritt beim Ausklammern aus? <br \> | '''b)''' Wie sieht der erste Zwischenschritt beim Ausklammern aus? <br \> | ||
{{Lösung versteckt|1=Schaue bei Teilaufgabe a) nach, was du ausklammerst. | |||
|2=Tipp|3=Verbergen}} | |||
<div class="multiplechoice-quiz"> | <div class="multiplechoice-quiz"> | ||
(i) <math> 9x - 15 </math> (<math> 3 \cdot 3x - 3 \cdot 5 </math>) (!<math> 3 \cdot 9x - 3 \cdot 5 </math>) (!<math> 3 \cdot 3x + 3 \cdot 5 </math>) (!<math> | '''(i)''' <math> 9x - 15 </math> (<math> 3 \cdot 3x - 3 \cdot 5 </math>) (!<math> 3 \cdot 9x - 3 \cdot 5 </math>) (!<math> 3 \cdot 3x + 3 \cdot 5 </math>) (!<math> 3 \cdot x -15 </math>) | ||
(ii) <math> -36 + 12x </math> (<math> 12 \cdot [-3] + | '''(ii)''' <math> -36 + 12x </math> (<math> 12 \cdot [-3] + 12 \cdot x </math>) (!<math> 12 \cdot 3 + 12 \cdot x </math>) (!<math> 12 \cdot [-3] - 12 \cdot x </math>) (!<math> 12 \cdot [-3] + x </math>) | ||
</div> | </div> | ||
c) Klammere komplett aus: | '''c)''' Klammere komplett aus: | ||
{{Lösung versteckt|1=Schaue bei Teilaufgabe a) nach, was du ausklammerst und bei b) wie dein erster Zwischenschritt aussieht. Mache zur Überprüfung die Probe wie es im Kapitel zum [[#Terme faktorisieren|''Faktorisieren'']] erklärt ist. <br /> | |||
|2=Tipp|3=Verbergen}} | |||
<div class="multiplechoice-quiz"> | <div class="multiplechoice-quiz"> | ||
(i) <math> 9x - 15 </math> (<math> 3 \cdot [3x - 5] </math>) (!<math> 3 \cdot [9x - 15] </math>) (!<math> 3 \cdot [x - 5] </math>) (!<math> 3 \cdot [3x + 5] </math>) | '''(i)''' <math> 9x - 15 </math> (<math> 3 \cdot [3x - 5] </math>) (!<math> 3 \cdot [9x - 15] </math>) (!<math> 3 \cdot [x - 5] </math>) (!<math> 3 \cdot [3x + 5] </math>) | ||
(ii) <math> -36 + 12x </math> (<math> 12 \cdot [-3 + x] </math>) (!<math> 12 \cdot [3 + x ] </math>) (!<math> 12 \cdot [6 + 3x] </math>) (!<math> 3 \cdot [3 + x] </math>) | '''(ii)''' <math> -36 + 12x </math> (<math> 12 \cdot [-3 + x] </math>) (!<math> 12 \cdot [3 + x ] </math>) (!<math> 12 \cdot [6 + 3x] </math>) (!<math> 3 \cdot [3 + x] </math>) | ||
(iii) <math> | '''(iii)''' <math> 5xy + 4xz + 3x </math> (!<math> x \cdot [54yz + 3]</math>) (<math> x \cdot [5y + 4z + 3] </math>) (!<math> 2x \cdot [5y + 4z + 3]</math>) (!<math> 5 \cdot [xy + 4xz + 3x]</math>) | ||
</div> | </div> | ||
| 3= Arbeitsmethode | Farbe={{Farbe|orange}} }} | | 3= Arbeitsmethode | Farbe={{Farbe|orange}} }} | ||
====Weitere | ====Weitere Aufgaben zum Ausmultiplizieren und Faktorisieren==== | ||
{{Box | 1= Aufgabe 3 | 2= Welche Zahl muss man einsetzen, damit die Umformung stimmt? | {{Box | 1= Aufgabe 3: Fülle die Lücken aus | 2= Welche Zahl muss man einsetzen, damit die Umformung stimmt? | ||
<div class="lueckentext-quiz"> | <div class="lueckentext-quiz"> | ||
a) '''3()''' <math> \cdot ( | a) '''3()''' <math> \cdot (2x + 7) = 6x + 21 </math> | ||
b) '''15()''' <math> \cdot x + 35 = 5 \cdot ( | {{Lösung versteckt|1=Klammere die rechte Seite des Terms aus. | ||
c) <math> 4 \cdot (- | |2=Tipp 1|3=Verbergen}} | ||
d) <math> | {{Lösung versteckt|1=Überlege dir dafür zunächst was du mit <math>2x</math> multiplizieren musst, damit du <math>6x</math> erhältst bzw. was du mit <math>7</math> multiplizieren musst, damit du <math>21</math> erhältst. Mache die Probe, wenn du den Platzhalter ausgefüllt hast. | ||
e) <math> (- | |2=Tipp 2|3=Verbergen}} <br /> | ||
b) '''15()''' <math> \cdot x + 35 = 5 \cdot (3x + 7) </math> <br /> | |||
{{Lösung versteckt|1=Multipliziere die rechte Seite des Terms aus. | |||
|2=Tipp|3=Verbergen}} <br /> | |||
c) <math> 4 \cdot (-3z + 2) = </math> '''-12()''' <math> \cdot z + 8 </math> <br /> | |||
{{Lösung versteckt|1=Multipliziere die linke Seite des Terms aus. | |||
|2=Tipp|3=Verbergen}} <br /> | |||
d) <math> 9y - 15 = 3 \cdot (3y </math> '''-5()''' <math>)</math> <br /> | |||
{{Lösung versteckt|1=Klammere <math>3</math> auf der linken Seite des Terms aus. | |||
|2=Tipp|3=Verbergen}} <br /> | |||
e) <math> (-24a + 42) \cdot \frac{1}{6} = </math> '''-4()''' <math> \cdot a + </math> '''7()''' | |||
{{Lösung versteckt|1=Multipliziere die linke Seite des Terms aus. | |||
|2=Tipp|3=Verbergen}} <br /> | |||
</div> | </div> | ||
| Zeile 253: | Zeile 320: | ||
| 3=Arbeitsmethode}} | | 3=Arbeitsmethode}} | ||
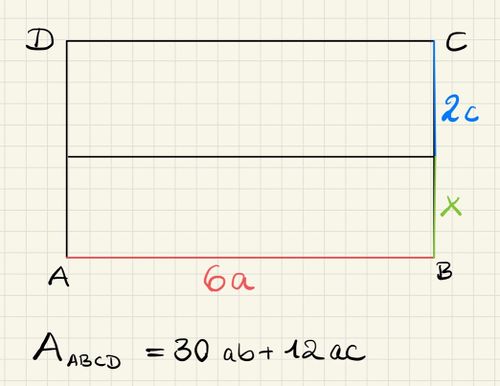
{{Box | 1= Aufgabe 4 | 2= | {{Box | 1= Aufgabe 4: Distributivgesetz veranschaulicht | 2= '''a)''' Wie lang ist die Strecke <math> x </math>?<br /> | ||
{{ | [[Datei:Knobel .jpg|500px|links]] <br /> <br /> <br /> <br /> <br /> <br /> <br /> <br /> <br /> <br /> <br /> <br /> <br /> <br /> <br /> <br /> <br /> <br /> | ||
{{Lösung versteckt|1=Was kannst du aus dem Term <math> 30ab+12ac </math>, der den Flächeninhalt des Rechtecks beschreibt, ausklammern? | |||
{{Lösung versteckt|1=<math> 30ab+12ac={\color{green}6a} \cdot 5b + {\color{green}6a} \cdot 2c = {\color{green}6a}(5b+2c) </math> | |||
|2=Lösung zu Tipp 1|3=Verbergen}} | |||
|2=Tipp 1|3=Verbergen}} | |||
{{Lösung versteckt|1=Stelle eine Beziehung zwischen der Grafik und dem ausgeklammerten Term <math> 6a(5b+2c) </math> her. Welche Stelle des Terms repräsentiert das <math> x </math>? | |||
{{Lösung versteckt|1=An der Grafik können wir ablesen, dass sich der Flächeninhalt für das Rechteck aus dem Term <math> 6a(x+2c) </math> ergibt. Vergleiche diesen mit dem ausgeklammerten Term <math> 6a(5b+2c) </math>. | |||
{{Lösung versteckt|1=Vergleichen wir <math> 6a({\color{green}5b}+2c) </math> mit <math> 6a({\color{green}x}+2c) </math>, so stellen wir fest, dass gilt: <math>x=5b</math>. | |||
|2=Restliche Lösung|3=Verbergen}} | |||
|2=Lösung zu Tipp 2|3=Verbergen}} | |||
|2=Tipp 2|3=Verbergen}} | |||
<div class="lueckentext-quiz"> | |||
<math> x = </math> '''5b()''' | |||
</div> | |||
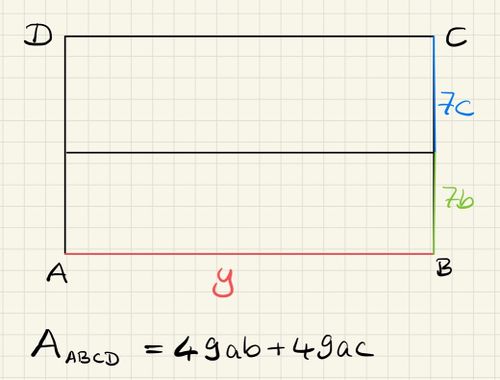
'''b)''' Wie lang ist die Strecke <math> y </math>?<br /> | |||
[[Datei:Knobelaufgabe.jpg|500px|links]] <br /> <br /> <br /> <br /> <br /> <br /> <br /> <br /> <br /> <br /> <br /> <br /> <br /> <br /> <br /> <br /> <br /> <br /> | |||
{{Lösung versteckt|1=Was kannst du aus dem Term <math> 49ab+49ac </math>, der den Flächeninhalt des Rechtecks beschreibt, ausklammern? | |||
{{Lösung versteckt|1=<math> 49ab+49ac={\color{green}7a} \cdot 7b + {\color{green}7a} \cdot 7c = {\color{green}7a}(7b+7c) </math> <br /> | |||
Du könntest zwar auch <math>49a</math> ausklammern, allerdings würde dich das in Bezug auf die Aufgabe nicht weiterbringen. Denn in der Aufgabe sind die Seitenlängen <math>{\color{blue}7b}</math> und <math>{\color{blue}7c}</math> gegeben, die sich im Term <math>7a({\color{blue}7b}+{\color{blue}7c})</math> wiederspiegeln. | |||
|2=Lösung zu Tipp 1|3=Verbergen}} | |||
|2=Tipp 1|3=Verbergen}} | |||
{{Lösung versteckt|1=Stelle eine Beziehung zwischen der Grafik und dem ausgeklammerten Term <math> 7a(7b+7c) </math> her. Welche Stelle des Terms repräsentiert das <math> y </math>? | |||
{{Lösung versteckt|1=An der Grafik können wir ablesen, dass sich der Flächeninhalt für das Rechteck aus dem Term <math> y(7b+7c) </math> ergibt. Vergleiche diesen mit dem ausgeklammerten Term <math> 7a(7b+7c) </math>. | |||
{{Lösung versteckt|1=Vergleichen wir <math> {\color{green}7a}(7b+7c) </math> mit <math> {\color{green}y}(7b+7c) </math>, so stellen wir fest, dass gilt: <math>y=7a</math>. | |||
|2=Restliche Lösung|3=Verbergen}} | |||
|2=Lösung zu Tipp 2|3=Verbergen}} | |||
|2=Tipp 2|3=Verbergen}} | |||
<div class="lueckentext-quiz"> | |||
<math> y = </math> '''7a()''' | |||
</div> | |||
| 3= Arbeitsmethode | Farbe={{Farbe|grün|dunkel}} }} | | 3= Arbeitsmethode | Farbe={{Farbe|grün|dunkel}} }} | ||
===3) Binomische Formeln=== | ===3) Binomische Formeln=== | ||
=====Was sind die binomischen Formeln?===== | =====Was sind die binomischen Formeln?===== | ||
{{Box | 1=Definition | 2=Die folgenden drei Umformungen bilden die sogenannten binomischen Formeln: <br /> <br /> <br /> | {{Box | 1=Definition | 2=Die folgenden drei Umformungen bilden die sogenannten binomischen Formeln: <br /> <br /> <br /> | ||
<div align="center">'''1. binomische Formel:''' <math>({\color{green}a}+{\color{blue}b})^2 = {\color{green}a}^2+2{\color{green}a}{\color{blue}b}+{\color{blue}b}^2 </math> </div> <br /> <br /> | <div align="center">'''1. binomische Formel:''' <math>({\color{green}a}+{\color{blue}b})^2 = {\color{green}a}^2+2{\color{green}a}{\color{blue}b}+{\color{blue}b}^2 </math> </div> <br /> <br /> | ||
<div align="center">'''2. binomische Formel:''' <math>({\color{green}a}-{\color{blue}b})^2 = {\color{green}a}^2-2{\color{green}a}{\color{blue}b}+{\color{blue}b}^2 </math> </div> <br /> <br /> | <div align="center">'''2. binomische Formel:''' <math>({\color{green}a}-{\color{blue}b})^2 = {\color{green}a}^2-2{\color{green}a}{\color{blue}b}+{\color{blue}b}^2 </math> </div> <br /> <br /> | ||
<div align="center">'''3. binomische Formel:''' <math>({\color{green}a}+{\color{blue}b})({\color{green}a}-{\color{blue}b}) = {\color{green}a}^2-{\color{blue}b}^2 </math> </div> <br / | <div align="center">'''3. binomische Formel:''' <math>({\color{green}a}+{\color{blue}b})({\color{green}a}-{\color{blue}b}) = {\color{green}a}^2-{\color{blue}b}^2 </math> </div> <br />| 3=Merksatz}} | ||
=====Herleitung der binomischen Formeln===== | =====Herleitung der binomischen Formeln===== | ||
{{Box | 1= | {{Box | 1=Übungsaufgabe: Binomische Formeln herleiten | 2=Versuche, die erste binomische Formel in deinem Heft rechnerisch herzuleiten. <br />Stelle dazu eine Gleichungskette der Form <math> (a+b)^2 = ... = a^2+2ab+b^2 </math> auf. | ||
{{Lösung versteckt|1=Beginne mit dem Ausgangsterm <math>(a+b)^2</math> und schreibe die Potenz | {{Lösung versteckt|1=Beginne mit dem Ausgangsterm <math>(a+b)^2</math> und schreibe die Potenz wie folgt aus: <math>(a+b)\cdot(a+b)</math>. Dies kannst du nun nach den bekannten Regeln ausmultiplizieren.|2=Tipp|3=Tipp ausblenden}} | ||
{{Lösung versteckt|1= Zunächst beginnt man mit dem Ausgangsterm <div align="center"><math>({\color{green}a}+{\color{blue}b})^2</math></div> <br /> Nun wird die Potenz ausgeschrieben <div align="center"> <math>=({\color{green}a}+{\color{blue}b})\cdot({\color{green}a}+{\color{blue}b})</math> </div> <br /> Als nächstes werden die Klammern ausmultipliziert <div align="center"> <math>={\color{green}a}{\color{green}a}+{\color{green}a}{\color{blue}b}+{\color{blue}b}{\color{green}a}+{\color{blue}b}{\color{blue}b}</math> </div> <br /> Das Kommutativgesetz (Vertauschungsgesetz) liefert das Ergebnis: <div align="center"> <math>={\color{green}a}^2+2{\color{green}a}{\color{blue}b}+{\color{blue}b}^2</math> </div>|2=Lösung|3=Lösung ausblenden}} | {{Lösung versteckt|1= Zunächst beginnt man mit dem Ausgangsterm <div align="center"><math>({\color{green}a}+{\color{blue}b})^2</math></div> <br /> Nun wird die Potenz ausgeschrieben <div align="center"> <math>=({\color{green}a}+{\color{blue}b})\cdot({\color{green}a}+{\color{blue}b})</math> </div> <br /> Als nächstes werden die Klammern ausmultipliziert <div align="center"> <math>={\color{green}a}{\color{green}a}+{\color{green}a}{\color{blue}b}+{\color{blue}b}{\color{green}a}+{\color{blue}b}{\color{blue}b}</math> </div> <br /> Das Kommutativgesetz (Vertauschungsgesetz) liefert das Ergebnis: <div align="center"> <math>={\color{green}a}^2+2{\color{green}a}{\color{blue}b}+{\color{blue}b}^2</math> </div>|2=Lösung|3=Lösung ausblenden}} | ||
{{Lösung versteckt|1=<math>({\color{green}(2x)}+{\color{blue}z})^2 = {\color{green}(2x)}^2+2{\color{green}(2x)}{\color{blue}z}+{\color{blue}z}^2 = 2^2x^2+2 \cdot 2xz+z^2 = 4x^2+4xz+z^2</math> |2=Anwendungsbeispiel der ersten binomischen Formel|3=Beispiel ausblenden}}| 3= | {{Lösung versteckt|1=<math>({\color{green}(2x)}+{\color{blue}z})^2 = {\color{green}(2x)}^2+2{\color{green}(2x)}{\color{blue}z}+{\color{blue}z}^2 = 2^2x^2+2 \cdot 2xz+z^2 = 4x^2+4xz+z^2</math> |2=Anwendungsbeispiel der ersten binomischen Formel|3=Beispiel ausblenden}}| 3=Arbeitsmethode}} | ||
{{Box|1= | |||
{{Box|1=Geometrische Herleitung|2= | |||
Neben der rechnerischen Lösung gibt es noch eine ''anschaulichere Möglichkeit'', die binomischen Formeln herzuleiten. Dies gelingt über das '''Vergleichen von Flächen'''. Ziehe die Punkte an den Balken nach rechts oder links, um die Werte von a und b zu verändern. Beobachte, was das Vergrößern bzw. Verkleinern dieser Werte '''geometrisch''' und '''rechnerisch''' bewirkt. | |||
<ggb_applet id="WEEdZyfV" width="1000" height="550" border="888888" /> | <ggb_applet id="WEEdZyfV" width="1000" height="550" border="888888" /> | ||
Der Flächeninhalt des großen Quadrats ist <math>(a+b)^2 </math> und damit gleich dem Ergebnis der 1. binomischen Formel. An der Zeichnung sieht man, dass sich das Quadrat aus vier Teilflächen zusammensetzt. Diese haben die Flächeninhalte <math>a^2, a \cdot b, b \cdot a, b^2 </math>. Die Fläche des Quadrats ergibt sich als Summe der Teilflächen: <math>a^2+ 2ab+ b^2 </math> Das ist gerade die 1. binomischen Formel. | Der Flächeninhalt des großen Quadrats ist <math>(a+b)^2 </math> und damit gleich dem Ergebnis der 1. binomischen Formel. An der Zeichnung sieht man, dass sich das Quadrat aus '''vier Teilflächen''' zusammensetzt. Diese haben die Flächeninhalte <math>a^2, a \cdot b, b \cdot a, b^2 </math>. Die Fläche des Quadrats ergibt sich als '''Summe der Teilflächen''': <math>a^2+ 2ab+ b^2 </math> Das ist gerade die '''1. binomischen Formel'''. | ||
|3= | |3=Merksatz}} | ||
{{Box|1=Beachte|2=Bisher hast du lediglich die Herleitung der | |||
https://de.serlo.org/mathe/terme-gleichungen/terme-variablen/binomische-formeln|3= | |||
{{Box|1=Beachte|2= | |||
* Bisher hast du lediglich die Herleitung der '''1. binomischen Formel''' kennengelernt. Die Herleitungen der '''2. und 3. binomischen Formel''' erfolgen sehr ähnlich und werden hier nicht thematisiert. Falls du dich trotzdem dafür interessierst, schau doch gerne mal bei Serlo vorbei: https://de.serlo.org/mathe/terme-gleichungen/terme-variablen/binomische-formeln | |||
* Die binomischen Formeln werden dir im Laufe deiner Schulzeit immer wieder begegnen, weshalb du sie unbedingt auswendig können solltest. Falls dir dies schwer fällt, schaue dir folgendes Video dazu an. <br \> <br \> <div align="center">{{#ev:youtube|EYbvhWEG6kE}}</div>|3=Merksatz}} | |||
=====Beispiele===== | =====Beispiele===== | ||
{{Box|1=Anwendungsbeispiele|2=Falls du dich mit den binomischen Formeln noch nicht vertraut genug fühlst, hast du hier die Möglichkeit, anhand von einigen Beispielen dein Verständnis zu fördern. Andernfalls kannst du direkt zum Aufgabenteil übergehen | {{Box|1=Anwendungsbeispiele|2= | ||
Falls du dich mit den binomischen Formeln noch nicht vertraut genug fühlst, hast du hier die Möglichkeit, anhand von einigen Beispielen dein Verständnis zu fördern. Andernfalls kannst du direkt zum Aufgabenteil übergehen. | |||
{{Lösung versteckt|1= | {{Lösung versteckt|1= | ||
Zur Erinnerung: <div align="center">'''1. binomische Formel:''' <math>({\color{green}a}+{\color{blue}b})^2 = {\color{green}a}^2+2{\color{green}a}{\color{blue}b}+{\color{blue}b}^2 </math> </div> <br \> <br \> | Zur Erinnerung: <div align="center">'''1. binomische Formel:''' <math>({\color{green}a}+{\color{blue}b})^2 = {\color{green}a}^2+2{\color{green}a}{\color{blue}b}+{\color{blue}b}^2 </math> </div> <br \> <br \> | ||
Für a und b können verschiedene Zahlen eingesetzt werden: <br \> | Für <math>a</math> und <math>b</math> können verschiedene Zahlen eingesetzt werden: <br \> | ||
a)<math>({\color{green}5}+{\color{blue}3})^2 = {\color{green}5}^2+2 \cdot {\color{green}5} \cdot {\color{blue}3}+{\color{blue}3}^2 = 25+30+9 = 64 (=8^2)</math> <br \> <br \> | a)<math>({\color{green}5}+{\color{blue}3})^2 = {\color{green}5}^2+2 \cdot {\color{green}5} \cdot {\color{blue}3}+{\color{blue}3}^2 = 25+30+9 = 64 (=8^2)</math> <br \> <br \> | ||
Für a und b können auch andere Variablen eingesetzt werden: <br \> | Für <math>a</math> und <math>b</math> können auch andere Variablen eingesetzt werden: <br \> | ||
b)<math>({\color{green}(uv)}+{\color{blue}w})^2 = {\color{green}(uv)}^2+2{\color{green}(uv)}{\color{blue}w}+{\color{blue}w}^2 = u^2v^2+2 \cdot uvw+w^2 </math> <br \> <br \> | b)<math>({\color{green}(uv)}+{\color{blue}w})^2 = {\color{green}(uv)}^2+2{\color{green}(uv)}{\color{blue}w}+{\color{blue}w}^2 = u^2v^2+2 \cdot uvw+w^2 </math> <br \> <br \> | ||
Selbst längere Terme kann man für a und b einsetzen: <br \> | Selbst längere Terme kann man für <math>a</math> und <math>b</math> einsetzen: <br \> | ||
c)<math>({\color{green}(2s+t)}+{\color{blue}u})^2 = {\color{green}(2s+t)}^2+2{\color{green}(2s+t)}{\color{blue}u}+{\color{blue}u}^2 | c)<math>({\color{green}(2s+t)}+{\color{blue}u})^2 = {\color{green}(2s+t)}^2+2{\color{green}(2s+t)}{\color{blue}u}+{\color{blue}u}^2 </math> <br \> | ||
|2=Beispiele zur 1. binomischen Formel|3=Beispiele ausblenden}} | |2=Beispiele zur 1. binomischen Formel|3=Beispiele ausblenden}} | ||
{{Lösung versteckt|1= | {{Lösung versteckt|1= | ||
Zur Erinnerung: <div align="center">'''2. binomische Formel:''' <math>({\color{green}a}-{\color{blue}b})^2 = {\color{green}a}^2-2{\color{green}a}{\color{blue}b}+{\color{blue}b}^2 </math> </div> <br /> <br /> | Zur Erinnerung: <div align="center">'''2. binomische Formel:''' <math>({\color{green}a}-{\color{blue}b})^2 = {\color{green}a}^2-2{\color{green}a}{\color{blue}b}+{\color{blue}b}^2 </math> </div> <br /> <br /> | ||
a)<math>({\color{green}5x}-{\color{blue}3y})^2 = {\color{green}(5x)}^2-2{\color{green}(5x)}{\color{blue}(3y)}+{\color{blue}(3y)}^2 = 25x^2+30xy+9y^2</math> <br \> <br \> | a)<math>({\color{green}5x}-{\color{blue}3y})^2 = {\color{green}(5x)}^2-2{\color{green}(5x)}{\color{blue}(3y)}+{\color{blue}(3y)}^2 = 25x^2+30xy+9y^2</math> <br \> <br \> | ||
Die Reihenfolge der Variablen in der Klammer kann manchmal | Die Reihenfolge der Variablen in der Klammer kann manchmal anders herum sein. In diesem Fall wird zunächst das Kommutativgesetz verwendet, <u>bevor</u> die binomische Formel angewendet wird: <br /> | ||
b)<math>(-{\color{blue}2}+{\color{green}w})^2 = ({\color{green}w}-{\color{blue}2})^2 = {\color{green}w}^2-2{\color{green}w}{\color{blue}(2)}+{\color{blue}2}^2 = w^2-4w+4</math> <br \> <br \> | b)<math>(-{\color{blue}2}+{\color{green}w})^2 = ({\color{green}w}-{\color{blue}2})^2 = {\color{green}w}^2-2{\color{green}w}{\color{blue}2}+{\color{blue}2}^2 = w^2-4w+4</math> <br \> <br \> | ||
Alternativ kann auch direkt die 1. binomische Formel angewendet werden: | |||
<math>(-{\color{blue}2}+{\color{green}w})^2 = (-{\color{blue}2})^2+2{\color{blue}(-2)}{\color{green}w}+{\color{green}w}^2 = 4-4w+w^2 = w^2-4w+4</math> <br \> <br \> | |||
c)<math>({\color{green}ab}-{\color{blue}4t})^2 = {\color{green}(ab)}^2-2{\color{green}(ab)}{\color{blue}(4t)}+{\color{blue}(4t)}^2 = a^2b^2-2(ab)(4t)+4^2t^2 = a^2b^2-8abt+16t^2</math> <br \>|2=Beispiele zur 2. binomischen Formel|3=Beispiele ausblenden}} | c)<math>({\color{green}ab}-{\color{blue}4t})^2 = {\color{green}(ab)}^2-2{\color{green}(ab)}{\color{blue}(4t)}+{\color{blue}(4t)}^2 = a^2b^2-2(ab)(4t)+4^2t^2 = a^2b^2-8abt+16t^2</math> <br \>|2=Beispiele zur 2. binomischen Formel|3=Beispiele ausblenden}} | ||
{{Lösung versteckt|1= | {{Lösung versteckt|1= | ||
Zur Erinnerung: <div align="center">'''3. binomische Formel:''' <math>({\color{green}a}+{\color{blue}b})({\color{green}a}-{\color{blue}b}) = {\color{green}a}^2-{\color{blue}b}^2 </math> </div> <br /> <br /> | Zur Erinnerung: <div align="center">'''3. binomische Formel:''' <math>({\color{green}a}+{\color{blue}b})({\color{green}a}-{\color{blue}b}) = {\color{green}a}^2-{\color{blue}b}^2 </math> </div> <br /> <br /> | ||
a) <math>({\color{green}(2h)}+{\color{blue}i})({\color{green}(2h)}-{\color{blue}i}) = {\color{green}(2h)}^2-{\color{blue}i}^2 </math> <br /> <br /> | a) <math>({\color{green}(2h)}+{\color{blue}i})({\color{green}(2h)}-{\color{blue}i}) = {\color{green}(2h)}^2-{\color{blue}i}^2 </math> <br /> <br /> | ||
Es spielt keine Rolle, | Es spielt keine Rolle, ob zuerst die ''Summe'' oder die ''Differenz'' erscheint (Assoziativgesetz): <br /> | ||
b) <math>({\color{green}(mn)}-{\color{blue}{1\over 3}})({\color{green}(mn)}+{\color{blue}{1\over 3}}) = {\color{green}(mn)}^2-{\color{blue}({1\over 3})}^2 = m^2n^2-{1\over 9}</math> <br /> <br /> | b) <math>({\color{green}(mn)}-{\color{blue}{1\over 3}})({\color{green}(mn)}+{\color{blue}{1\over 3}}) = {\color{green}(mn)}^2-{\color{blue}({1\over 3})}^2 = m^2n^2-{1\over 9}</math> <br /> <br /> | ||
c) <math>({\color{green}(3x)}+{\color{blue}\sqrt{2}})({\color{green}(3x)}-{\color{blue}\sqrt{2}}) = {\color{green}(3x)}^2-{\color{blue}(\sqrt{2})}^2 = 9x^2-2 </math> <br /> <br /> | c) <math>({\color{green}(3x)}+{\color{blue}\sqrt{2}})({\color{green}(3x)}-{\color{blue}\sqrt{2}}) = {\color{green}(3x)}^2-{\color{blue}(\sqrt{2})}^2 = 9x^2-2 </math> <br /> <br /> | ||
|2=Beispiele zur 3. binomischen Formel|3=Beispiele ausblenden}}|3= | |2=Beispiele zur 3. binomischen Formel|3=Beispiele ausblenden}}|3=Hervorhebung1}} | ||
{{Box | 1=Aufgabe 1: Welche binomische Formel?| 2=Ordne | ====Aufgaben==== | ||
{{Box|1=Aufgabe 1: Welche binomische Formel?|2= Ordne zu. | |||
<div class="zuordnungs-quiz"> | <div class="zuordnungs-quiz"> | ||
{{{!}} | |||
{{!}} 1. binomische Formel {{!}}{{!}} <math forcemathmode="png">(x+19)^2</math> {{!}}{{!}} <math forcemathmode="png">({3\over 4}+p)^2</math> {{!}}{{!}} <math forcemathmode="png">(1{,}34+\sqrt{5})^2</math> | |||
{{!}}- | |||
{{!}} 2. binomische Formel {{!}}{{!}} <math forcemathmode="png">(19-x)^2</math> {{!}}{{!}} <math forcemathmode="png">(3-5)^2</math> {{!}}{{!}} <math forcemathmode="png">(25-y)^2</math> | |||
{{!}}- | |||
{{!}} 3. binomische Formel {{!}}{{!}} <math forcemathmode="png">(5+t)(5-t)</math> {{!}}{{!}} <math forcemathmode="png">({3\over 8}-7)(7+{3\over 8})</math> {{!}}{{!}} <math forcemathmode="png">(1{,}37-2)(1{,}37+2)</math> | |||
{{!}}- | |||
{{!}} Das ist keine binomische Formel {{!}}{{!}} <math forcemathmode="png">(4+7)(5-7)</math> {{!}}{{!}} <math forcemathmode="png">(5+7)^{1\over 2}</math> {{!}}{{!}} <math forcemathmode="png">s+3^2</math> | |||
{{!}}} | |||
</div> | |||
|3=Arbeitsmethode|Farbe={{Farbe|grün|dunkel}} | |||
}} | |||
{{Box | 1=Aufgabe 2: Nächste Runde rückwärts (..ärts, ärts..)| 2= | {{Box | 1=Aufgabe 2: Nächste Runde rückwärts (..ärts, ärts..)| 2= | ||
Tom möchte die binomischen Formeln lieber rückwärts verwenden. Leider weiß er nicht wirklich wie. Kannst du ihm helfen? Notiere den Rechnungsweg in dein Heft und trage die korrekten Werte unten ein. | |||
[[Datei:CrangerKirmes05.jpg|mini|center|Alle einsteigen bitte]] | |||
<div class="lueckentext-quiz"> | |||
a) <math> 225+30a+a^2 = (</math>'''15()'''<math>+</math>'''a()'''<math>)^2 </math> <br /> | |||
b) <math> 9a^2-16b^2 = (</math>'''3a()'''<math>+</math>'''4b()'''<math>)\cdot ( </math>'''3a()'''<math>-</math>'''4b()''') <br /> | |||
c) <math> 81u^2-36u+4 = (</math>'''9u()'''<math>-</math>'''2()'''<math>)^2 </math> <br /> | |||
d) <math> 4m^2+28m+49 = (</math>'''2m()'''<math>+</math>'''7()'''<math>)^2 </math> <br /> | |||
e) <math> 64y^2-160yz+100z^2 = (</math>'''8y()'''<math>-</math>'''10z()'''<math>)^2 </math> <br /> | |||
f) <math> 36u^2-121w^2 = (</math>'''6u()'''<math>+</math>'''11w()'''<math>)\cdot ( </math>'''6u()'''<math>-</math>'''11w()''') <br /> | |||
</div> | |||
<div | {{Lösung versteckt|1= | ||
'''Klammere aus'''. Falls du dir unsicher bist, mache die Probe. Du kannst auch [[#Terme faktorisieren|hier]] noch einmal vorbeischauen. | |||
Schaue dir auch noch einmal die [[#Was sind die binomischen Formeln?|binomischen Formeln]] an und entscheide, '''wann''' du '''welche''' Formel anwenden kannst.|2=Tipp 1|3=Tipp ausblenden}} | |||
{{Lösung versteckt|1= | |||
Wir suchen die passende binomische Formel für den Term <math> 9x^2-6xy+y^2 </math>. | |||
Die Anzahl der Summanden bzw. Minuenden geben uns Auskunft darüber, welche binomische Formel wir anwenden können. In diesem Fall haben wir '''zwei Summanden''' und '''einen Minuenden'''. Dies stimmt mit der '''2. binomischen Formel''' überein: <br /> | |||
<div align="center"> <math>(a-b)^2 = a^2-2ab+b^2 </math>. Unsere binomische Formel hat also die Form <math>(a-b)^2 </math>. </div> <br /> | |||
Nun müssen wir noch a und b herausfinden. Wir wissen, dass <math> a^2 = 9x^2 </math> und <math> b^2 = y^2 </math>. | |||
Nun ziehen wir aus diesen Ausdrücken die Wurzel, um a und b zu erhalten: <br /> | |||
<div align="center"> <math> a = \sqrt{a^2} = \sqrt{9x^2} = 3x </math> und <math> b = \sqrt{b^2} = \sqrt{y^2} = y </math>. </div> | |||
Also lautet die binomische Formel <math> (3x-y)^2 </math>. | |||
<br /> <br /> | |||
Probe:<div align="center"> <math> ({\color{green}3x}-{\color{blue}y})^2 = {\color{green}(3x)}^2-2 \cdot {\color{green}3x}{\color{blue}y}+{\color{blue}y}^2 = 9x^2-6xy+y^2 </math>. </div> <br /> | |||
Das Vorgehen für die 1. und 3. binomische Formel erfolgt sehr ähnlich. Falls du trotzdem Probleme beim Lösen der Aufgabe hast, siehe dir Tipp 2 an.|2=Beispiel|3=Beispiel verbergen}} | |||
{{Lösung versteckt|1= | |||
* Bei '''drei Summanden''' wendest du die '''1. binomische Formel''' an. | |||
* Bei '''zwei Summanden und einem Minuenden''' wendest du die '''2. binomische Formel''' an. | |||
* Bei '''einem Summanden und einem Minuenden''' wendest du die '''3. binomische Formel''' an.|2=Tipp 2|3=Tipp ausblenden}}| 3=Arbeitsmethode}} | |||
{{Box | 1=Aufgabe 3: Warum ist das so?| 2= | |||
Gegeben sind nun allgemein zwei aufeinanderfolgende ''natürliche'' Zahlen <math> a </math> und <math> b </math> mit | |||
<math> b = a-1 </math>. <br /> | |||
Begründe unter Verwendung einer binomischen Formel, dass die folgende Rechenregel immer stimmt: <br /> | |||
Die Differenz <math> a^2-b^2 </math> ist gleich der Summe <math> a+b </math>. Notiere deinen Lösungsweg in dein Heft. | |||
{{Lösung versteckt|1= Bilde eine Gleichungskette <math> a^2-b^2 = ... = a+b </math> |2=Tipp 1|3=Tipp verbergen}} | |||
{{Lösung versteckt|1= Verwende bei der Umformung die dritte binomische Formel. |2=Tipp 2|3=Tipp verbergen}} | |||
{{Lösung versteckt|1= Setze für <math> b = a-1 </math> ein. |2=Tipp 3|3=Tipp verbergen}} | |||
{{Lösung versteckt|1= | |||
Zunächst wird die 3. binomische Formel ausgenutzt: <div align="center"><math>a^2-b^2 = (a-b)(a+b)</math></div> <br /> Dann wird für <math> b = a-1 </math> eingesetzt: <div align="center"> <math>= (a-(a-1))(a+(a-1)) </math> </div> <br /> Nun die erste Klammer auflösen: <div align="center"> <math>= 1 \cdot (a+(a-1))</math> </div> <br /> Schließlich für <math> a-1 = b </math> einsetzen: <div align="center"> <math>= 1 \cdot (a+(b)) = a+b </math> </div> | |||
<br /> | |||
Alternativ: | |||
<math> a^2-b^2 = (a+b)(a-b) = (a+b) \cdot 1 = a+b </math> | |||
oder: | |||
<math> a^2-b^2 = a^2-(a-1)^2 = a^2-(a^2-2a+1) = a^2-a^2+2a-1 = 2a-1 = a+(a-1) = a+b </math> | |||
|2= Lösung|3= Lösung verbergen}}|3=Arbeitsmethode|Farbe={{Farbe|grün|dunkel}} }} | |||
Aktuelle Version vom 16. Dezember 2020, 12:05 Uhr
1) Terme zusammenfassen
Einführung
Aufgaben
2) Terme ausmultiplizieren und faktorisieren
Terme ausmultiplizieren
Aufgabe
Terme faktorisieren
Aufgabe
Weitere Aufgaben zum Ausmultiplizieren und Faktorisieren
3) Binomische Formeln
Was sind die binomischen Formeln?
Herleitung der binomischen Formeln
Beispiele
Aufgaben