Benutzer:Carolin Uni MS-14/Testseite: Unterschied zwischen den Versionen
(Die Seite wurde neu angelegt: „Test“) Markierung: 2017-Quelltext-Bearbeitung |
Keine Bearbeitungszusammenfassung Markierung: 2017-Quelltext-Bearbeitung |
||
| (14 dazwischenliegende Versionen desselben Benutzers werden nicht angezeigt) | |||
| Zeile 1: | Zeile 1: | ||
==Spielwiese== | |||
===Schreiben im Wiki=== | |||
Neben normalem Text kann man auch ''kursiven'' oder '''fett gedruckten''' Text schreiben. '''''Ebenso ist eine Kombination aus beidem möglich.''''' <span style="color: green"> Grüner Text ist schon etwas schwieriger</span>, funktioniert aber über die Quelltextbearbeitung. | |||
Einen neuen Absatz beginnt man in der Quelltextbearbeitung durch zwei aufeinanderfolgende Zeilenumbrüche, also einer leeren Zeile zwischen den beiden Absätzen. In der visuellen Bearbeitung reicht hierzu das einmalige Betätigen der Eingabetaste. | |||
<ggb_applet id="xpTk3wBe" width="1000" height="470" /> | |||
===Vorlagen=== | |||
{{Lösung versteckt|Das ist ein Tipp|Tipp anzeigen|Tipp verbergen}}{{Lösung versteckt|Das ist eine Lösung|Lösung anzeigen|Lösung verbergen}}{{Box|Aufgabe 1: Münzwurf|Inhalt|Arbeitsmethode | |||
}}{{Box|Merksatz: Kongruenzsätze|Inhalt|Merksatz | |||
}}{{Box|Beispiel: Polynomdivision|Inhalt|Hervorhebung1 | |||
}} | |||
===Dateien=== | |||
====Über die Bedienelemente==== | |||
[[Datei:Monty Hall. Ilustración de paradoja. Puerta abierta.png|mini|rechts|Ziegenproblem]]Lorem ipsum dolor sit amet, consectetur adipisici elit, sed eiusmod tempor incidunt ut labore et dolore magna aliqua. Ut enim ad minim veniam, quis nostrud exercitation ullamco laboris nisi ut aliquid ex ea commodi consequat. Quis aute iure reprehenderit in voluptate velit esse cillum dolore eu fugiat nulla pariatur. Excepteur sint obcaecat cupiditat non proident, sunt in culpa qui officia deserunt mollit anim id est laborum. Lorem ipsum dolor sit amet, consectetur adipisici elit, sed eiusmod tempor incidunt ut labore et dolore magna aliqua. Ut enim ad minim veniam, quis nostrud exercitation ullamco laboris nisi ut aliquid ex ea commodi consequat. Quis aute iure reprehenderit in voluptate velit esse cillum dolore eu fugiat nulla pariatur. Excepteur sint obcaecat cupiditat non proident, sunt in culpa qui officia deserunt mollit anim id est laborum. Lorem ipsum dolor sit amet, consectetur adipisici elit, sed eiusmod tempor incidunt ut labore et dolore magna aliqua. Ut enim ad minim veniam, quis nostrud exercitation ullamco laboris nisi ut aliquid ex ea commodi consequat. Quis aute iure reprehenderit in voluptate velit esse cillum dolore eu fugiat nulla pariatur. Excepteur sint obcaecat cupiditat non proident, sunt in culpa qui officia deserunt mollit anim id est laborum. | |||
====Mittels Quelltexteingabe (Ohne Umfließen des Textes)==== | |||
Lorem ipsum dolor sit amet, consectetur adipisici elit, sed eiusmod tempor incidunt ut labore et dolore magna aliqua. Ut enim ad minim veniam, quis nostrud exercitation ullamco laboris nisi ut aliquid ex ea commodi consequat. Quis aute iure reprehenderit in voluptate velit esse cillum dolore eu fugiat nulla pariatur. Excepteur sint obcaecat cupiditat non proident, sunt in culpa qui officia deserunt mollit anim id est laborum.[[Datei:GIF Basketball.gif|mini|ohne|Ballwurf]]Lorem ipsum dolor sit amet, consectetur adipisici elit, sed eiusmod tempor incidunt ut labore et dolore magna aliqua. Ut enim ad minim veniam, quis nostrud exercitation ullamco laboris nisi ut aliquid ex ea commodi consequat. Quis aute iure reprehenderit in voluptate velit esse cillum dolore eu fugiat nulla pariatur. Excepteur sint obcaecat cupiditat non proident, sunt in culpa qui officia deserunt mollit anim id est laborum. | |||
====Über Wikipedia (Ohne Rahmen)==== | |||
Lorem ipsum dolor sit amet, consectetur adipisici elit, sed eiusmod tempor incidunt ut labore et dolore magna aliqua. Ut enim ad minim veniam, quis nostrud exercitation ullamco laboris nisi ut aliquid ex ea commodi consequat. Quis aute iure reprehenderit in voluptate velit esse cillum dolore eu fugiat nulla pariatur. Excepteur sint obcaecat cupiditat non proident, sunt in culpa qui officia deserunt mollit anim id est laborum.[[File:KreisMittelpunktRadius.svg|rahmenlos|ohne|500px]]Lorem ipsum dolor sit amet, consectetur adipisici elit, sed eiusmod tempor incidunt ut labore et dolore magna aliqua. Ut enim ad minim veniam, quis nostrud exercitation ullamco laboris nisi ut aliquid ex ea commodi consequat. Quis aute iure reprehenderit in voluptate velit esse cillum dolore eu fugiat nulla pariatur. Excepteur sint obcaecat cupiditat non proident, sunt in culpa qui officia deserunt mollit anim id est laborum. | |||
===Interaktive Applets=== | |||
====LearningApp==== | |||
{{LearningApp|width=100%|height=600px|app=phcsyj21c17}} | |||
====GeoGebra==== | |||
<ggb_applet id="cDyjWjkp" width="100%" height="100%" /> | |||
==Kombinationen== | |||
Die Inhalte basieren auf dem Lernpfad [https://unterrichten.zum.de/wiki/Quadratische_Funktionen_erkunden Quadratische Funktionen erkunden] von [[Benutzer:Elena Jedtke|Elena Jedtke]]. | |||
{{Box|Merksatz: Quadratische Funktionen|Quadratische Funktionen können in der Form <math>f(x)=a(x-{\color{blue}d})^2+{\color{green}e}</math> angegeben werden (wobei <math>a \neq 0</math>). Diese Darstellungsform nennt man '''Scheitelpunktform''', da sich der Scheitelpunkt direkt ablesen lässt. Er hat die Koordinaten <math>S({\color{blue}d}|{\color{green}e})</math>.|Merksatz | |||
}}{{Box|Aufgabe 3: Von Angry Birds bis Basketball|Finde Werte für <math>a</math>, <math>d</math> und <math>e</math>, so dass <math>f(x)</math> die Kurve auf dem Bild möglichst gut beschreibt. Entscheide dich für drei Hintergrundbilder deiner Wahl und notiere den Funktionsterm in deinem Hefter. Wenn du noch weiter arbeiten möchtest, kannst du auch einige der übrigen Hintergundbilder bearbeiten. | |||
<ggb_applet id="cDyjWjkp" width="100%" height="100%"/> | |||
{{Lösung versteckt|1=Da es nicht die eine richtige Lösung gibt, findest du in der Tabelle Lösungsvorschläge sowie Spielräume, in denen die Parameter liegen können, um den Verlauf angemessen zu beschreiben. | |||
{{{!}} class="wikitable" | |||
{{!}}- | |||
! Hintergrundbild!! Lösungsvorschlag !! Parameter <math>a</math> !! Parameter <math>d</math> !! Parameter <math>e</math> | |||
{{!}}- | |||
{{!}} Angry Birds | |||
{{!}}{{!}} <math>f(x)=-0{,}13(x-7)^2+4{,}85</math> | |||
{{!}}style="text-align:center"{{!}} <math>-0{,}15 \leq a \leq -0{,}13</math> | |||
{{!}}style="text-align:center"{{!}} <math>6{,}80 \leq d \leq 7{,}20</math> | |||
{{!}}style="text-align:center"{{!}} <math>4{,}70 \leq e \leq 5{,}00</math> | |||
{{!}}- | |||
{{!}} Golden Gate Bridge | |||
{{!}}{{!}} <math>f(x)=0{,}04(x-5{,}7)^2+1</math> | |||
{{!}}style="text-align:center"{{!}} <math>0{,}03 \leq a \leq 0{,}05</math> | |||
{{!}}style="text-align:center"{{!}} <math>5{,}00 \leq d \leq 6{,}40</math> | |||
{{!}}style="text-align:center"{{!}} <math>0{,}80 \leq e \leq 1{,}10</math> | |||
{{!}}- | |||
{{!}} Springbrunnen | |||
{{!}}{{!}} <math>f(x)=-0{,}33(x-4{,}85)^2+5{,}3</math> | |||
{{!}}style="text-align:center"{{!}} <math>-0{,}40 \leq a \leq -0{,}30</math> | |||
{{!}}style="text-align:center"{{!}} <math>4{,}70 \leq d \leq 5{,}00</math> | |||
{{!}}style="text-align:center"{{!}} <math>5{,}10 \leq e \leq 5{,}50</math> | |||
{{!}}- | |||
{{!}} Elbphilharmonie (Bogen links) | |||
{{!}}{{!}} <math>f(x)=0{,}40(x-2{,}50)^2+4{,}35</math> | |||
{{!}}style="text-align:center"{{!}} <math>0{,}33 \leq a \leq 0{,}47</math> | |||
{{!}}style="text-align:center"{{!}} <math>2{,}40 \leq d \leq 2{,}60</math> | |||
{{!}}style="text-align:center"{{!}} <math>4{,}25 \leq e \leq 4{,}40</math> | |||
{{!}}- | |||
{{!}} Elbphilharmonie (Bogen mitte) | |||
{{!}}{{!}} <math>f(x)=0{,}33(x-5{,}85)^2+3{,}4</math> | |||
{{!}}style="text-align:center"{{!}} <math>0{,}30 \leq a \leq 0{,}36</math> | |||
{{!}}style="text-align:center"{{!}} <math>5{,}70 \leq d \leq 6{,}00</math> | |||
{{!}}style="text-align:center"{{!}} <math>3{,}20 \leq e \leq 3{,}60</math> | |||
{{!}}- | |||
{{!}} Elbphilharmonie (Bogen rechts) | |||
{{!}}{{!}} <math>f(x)=0{,}22(x-9{,}40)^2+3{,}60</math> | |||
{{!}}style="text-align:center"{{!}} <math>0{,}18 \leq a \leq 0{,}27</math> | |||
{{!}}style="text-align:center"{{!}} <math>9{,}30 \leq d \leq 9{,}50</math> | |||
{{!}}style="text-align:center"{{!}} <math>3{,}55 \leq e \leq 3{,}65</math> | |||
{{!}}- | |||
{{!}} Gebirgsformation | |||
{{!}}{{!}} <math>f(x)=-0{,}2(x-5{,}4)^2+2{,}3</math> | |||
{{!}}style="text-align:center"{{!}} <math>-0{,}30 \leq a \leq -0{,}10</math> | |||
{{!}}style="text-align:center"{{!}} <math>5{,}10 \leq d \leq 5{,}70</math> | |||
{{!}}style="text-align:center"{{!}} <math>2{,}10 \leq e \leq 2{,}50</math> | |||
{{!}}- | |||
{{!}} Motorrad-Stunt | |||
{{!}}{{!}} <math>f(x)=-0{,}07(x-7{,}7)^2+5{,}95</math> | |||
{{!}}style="text-align:center"{{!}} <math>-0{,}10 \leq a \leq -0{,}04</math> | |||
{{!}}style="text-align:center"{{!}} <math>7{,}30 \leq d \leq 8{,}10</math> | |||
{{!}}style="text-align:center"{{!}} <math>5{,}70 \leq e \leq 6{,}20</math> | |||
{{!}}- | |||
{{!}} Basketball | |||
{{!}}{{!}} <math>f(x)=-0{,}32(x-6{,}5)^2+6{,}45</math> | |||
{{!}}style="text-align:center"{{!}} <math>-0{,}35 \leq a \leq -0{,}29</math> | |||
{{!}}style="text-align:center"{{!}} <math>6{,}20 \leq d \leq 6{,}80</math> | |||
{{!}}style="text-align:center"{{!}} <math>6{,}20 \leq e \leq 6{,}70</math> | |||
{{!}}} | |||
|2=Lösungsvorschläge anzeigen|3=Lösungsvorschläge verbergen}}|Arbeitsmethode | |||
}} | |||
Auf dieser Seite sind verschiedene Vorlagen für die Lernpfadkapitel des Seminars [[Digitale Werkzeuge in der Schule|''Digitale Werkzeuge in der Schule'']] dargestellt. Dabei findet sich jeweils links im grau unterlegten Feld der benötigte Code um die Ausgabe rechts daneben zu erhalten. | |||
==Allgemeines== | |||
===Kapitel-Informationskästchen=== | |||
<span style="color: red">Bei Lernpfaden für die Sekundarstufe I oder Einführungsphase entfällt der Stichpunkt zu LK-Aufgaben!</span> | |||
<div class="grid"> | |||
<div class="width-1-2"> | |||
<pre> | |||
{{Box | |||
|1=Info | |||
|2=In diesem Lernpfadkapitel <Kurzbeschreibung des Kapitelziels> | |||
Für die Bearbeitung dieses Kapitels benötigst du den Hefter [https://projekte.zum.de/wiki/Datei:Hefter_zum_Lernpfad_Pyramiden_entdecken.pdf "Pyramiden entdecken"], einen Taschenrechner, einen Stift, einen Bleistift, vier verschiedenfarbige Stifte, einen Zirkel, ein Geodreieck, ein Lineal (mind. 7 cm lang), eine Schere, etwas Klebeband, zwei lose DIN A3-Blätter und zwei lose DIN A4-Blätter. | |||
Bei den Aufgaben unterscheiden wir folgende Typen: | |||
* In Aufgaben, die '''<span style="color: #F19E4F">orange</span>''' gefärbt sind, kannst du '''grundlegende Kompetenzen''' wiederholen und vertiefen. | |||
* Aufgaben in '''<span style="color: #CD2990">pinker</span>''' Farbe sind '''Aufgaben mittlerer Schwierigkeit'''. | |||
* Und Aufgaben mit '''<span style="color: #5E43A5">lilanem</span>''' Streifen sind '''Knobelaufgaben'''. | |||
* Aufgaben, die mit einem &#x2B50; gekennzeichnet sind, sind nur für den LK gedacht. | |||
Viel Erfolg! | |||
|3=Kurzinfo}} | |||
</pre> | |||
</div> | |||
<div class="width-1-2"> | |||
{{Box | |||
|1=Info | |||
|2=In diesem Lernpfadkapitel <Kurzbeschreibung des Kapitelziels> | |||
Für die Bearbeitung dieses Kapitels benötigst du den Hefter [https://projekte.zum.de/wiki/Datei:Hefter_zum_Lernpfad_Pyramiden_entdecken.pdf "Pyramiden entdecken"], einen Taschenrechner, einen Stift, einen Bleistift, vier verschiedenfarbige Stifte, einen Zirkel, ein Geodreieck, ein Lineal (mind. 7 cm lang), eine Schere, etwas Klebeband, zwei lose DIN A3-Blätter und zwei lose DIN A4-Blätter. | |||
Bei den Aufgaben unterscheiden wir folgende Typen: | |||
* In Aufgaben, die '''<span style="color: #F19E4F">orange</span>''' gefärbt sind, kannst du '''grundlegende Kompetenzen''' wiederholen und vertiefen. | |||
* Aufgaben in '''<span style="color: #CD2990">pinker</span>''' Farbe sind '''Aufgaben mittlerer Schwierigkeit'''. | |||
* Und Aufgaben mit '''<span style="color: #5E43A5">lilanem</span>''' Streifen sind '''Knobelaufgaben'''. | |||
* Aufgaben, die mit einem ⭐ gekennzeichnet sind, sind nur für den LK gedacht. | |||
Viel Erfolg! | |||
|3=Kurzinfo}} | |||
</div> | |||
</div> | |||
===Navigation=== | |||
<span style="color: red">Parameter ''vorherlink'' an aktuellen Lernpfad anpassen.</span> | |||
<div class="grid"> | |||
<div class="width-1-2"> | |||
<pre> | |||
{{Fortsetzung|vorher=zurück zur Kapitelauswahl|vorherlink=Digitale_Werkzeuge_in_der_Schule/LERNPFAD#Kapitelauswahl}} | |||
</pre> | |||
</div> | |||
<div class="width-1-2"> | |||
{{Fortsetzung|vorher=zurück zur Kapitelauswahl|vorherlink=Digitale_Werkzeuge_in_der_Schule/LERNPFAD#Kapitelauswahl}} | |||
</div> | |||
</div> | |||
<span style="color: red">Parameter ''weiterlink'' an aktuellen Lernpfad anpassen.</span> | |||
<div class="grid"> | |||
<div class="width-1-2"> | |||
<pre> | |||
{{Fortsetzung|weiter=weiter zum nächsten Kapitel|weiterlink=Digitale_Werkzeuge_in_der_Schule/LERNPFAD#Kapitel}} | |||
</pre> | |||
</div> | |||
<div class="width-1-2"> | |||
{{Fortsetzung|weiter=weiter zum nächsten Kapitel|weiterlink=Digitale_Werkzeuge_in_der_Schule/LERNPFAD#Kapitel}} | |||
</div> | |||
</div> | |||
<div class="grid"> | |||
<div class="width-1-2"> | |||
<pre> | |||
{{Lösung versteckt|1=Inhalt|2=Lösung|3=Lösung verbergen}} | |||
</pre> | |||
</div> | |||
<div class="width-1-2"> | |||
{{Lösung versteckt|1=Inhalt|2=Lösung|3=Lösung verbergen}} | |||
</div> | |||
</div> | |||
===Kategoriezuordnung=== | |||
<div class="grid"> | |||
<div class="width-1-2"> | |||
<pre> | |||
{{SORTIERUNG:{{SUBPAGENAME}}}} | |||
[[Kategorie:Digitale Werkzeuge in der Schule]] | |||
</pre> | |||
</div> | |||
<div class="width-1-2"> | |||
Siehe Kategorie am Ende dieser Seite. | |||
</div> | |||
</div> | |||
===Aufgaben-Boxen=== | |||
====Schwierigkeitsstufe I==== | |||
<div class="grid"> | |||
<div class="width-1-2"> | |||
<pre> | |||
{{Box | Aufgabe <Nummer>: <Name> | Inhalt | Arbeitsmethode | Farbe={{Farbe|orange}} }} | |||
</pre> | |||
</div> | |||
<div class="width-1-2"> | |||
{{Box | Aufgabe <Nummer>: <Name> | Inhalt | Arbeitsmethode | Farbe={{Farbe|orange}} }} | |||
</div> | |||
</div> | |||
====Schwierigkeitsstufe II==== | |||
<div class="grid"> | |||
<div class="width-1-2"> | |||
<pre> | |||
{{Box | Aufgabe <Nummer>: <Name> | Inhalt | Arbeitsmethode | Farbe=#CD2990 }} | |||
</pre> | |||
</div> | |||
<div class="width-1-2"> | |||
{{Box | Aufgabe <Nummer>: <Name> | Inhalt | Arbeitsmethode | Farbe=#CD2990 }} | |||
</div> | |||
</div> | |||
====Schwierigkeitsstufe III==== | |||
<div class="grid"> | |||
<div class="width-1-2"> | |||
<pre> | |||
{{Box | Aufgabe <Nummer>: <Name> | Inhalt | Arbeitsmethode}} | |||
</pre> | |||
</div> | |||
<div class="width-1-2"> | |||
{{Box | Aufgabe <Nummer>: <Name> | Inhalt | Arbeitsmethode}} | |||
</div> | |||
</div> | |||
====Mit Verweis zum Arbeitsblatt==== | |||
<div class="grid"> | |||
<div class="width-1-2"> | |||
<pre> | |||
{{Box | Aufgabe <Nummer>: <Name> | | |||
[[Datei:Grundlagen-bearbeiten.png|30px|middle]] '''zurück zum Arbeitsblatt''' | |||
| Arbeitsmethode | Farbe={{Farbe|orange}} }} | |||
</pre> | |||
</div> | |||
<div class="width-1-2"> | |||
{{Box | Aufgabe <Nummer>: <Name> | [[Datei:Grundlagen-bearbeiten.png|30px|middle]] '''zurück zum Arbeitsblatt''' | Arbeitsmethode | Farbe={{Farbe|orange}}}} | |||
</div> | |||
</div> | |||
====LK-Kennzeichnung==== | |||
=====Gesamte Aufgabe===== | |||
<div class="grid"> | |||
<div class="width-1-2"> | |||
<pre> | |||
{{Box | Aufgabe <Nummer>&#x2B50;: <Name> | Inhalt | Arbeitsmethode}} | |||
</pre> | |||
</div> | |||
<div class="width-1-2"> | |||
{{Box | Aufgabe <Nummer>⭐: <Name> | Inhalt | Arbeitsmethode}} | |||
</div> | |||
</div> | |||
=====Teilaufgabe===== | |||
<div class="grid"> | |||
<div class="width-1-2"> | |||
<pre> | |||
{{Box | Aufgabe <Nummer>: <Name> | | |||
'''a)''' Inhalt | |||
'''b)''' Inhalt | |||
'''c)&#x2B50;''' Inhalt | |||
| Arbeitsmethode}} | |||
</pre> | |||
</div> | |||
<div class="width-1-2"> | |||
{{Box | Aufgabe <Nummer>: <Name> | | |||
'''a)''' Inhalt | |||
'''b)''' Inhalt | |||
'''c)⭐''' Inhalt | |||
| Arbeitsmethode}} | |||
</div> | |||
</div> | |||
===Andere Boxen=== | |||
====Merksatz==== | |||
<div class="grid"> | |||
<div class="width-1-2"> | |||
<pre> | |||
{{Box | Merksatz: <Name> | Inhalt | Merksatz | Farbe={{Farbe|grün|dunkel}}}} | |||
</pre> | |||
</div> | |||
<div class="width-1-2"> | |||
{{Box | Merksatz: <Name> | Inhalt | Merksatz|| Farbe={{Farbe|grün|dunkel}}}} | |||
</div> | |||
</div> | |||
====Beispiel==== | |||
<div class="grid"> | |||
<div class="width-1-2"> | |||
<pre> | |||
{{Box | Beispiel: <Name> | Inhalt | Hervorhebung1}} | |||
</pre> | |||
</div> | |||
<div class="width-1-2"> | |||
{{Box | Beispiel: <Name> | Inhalt | Hervorhebung1}} | |||
</div> | |||
</div> | |||
===Eingebettete Aufgabenstellung=== | |||
<div class="grid"> | |||
<div class="width-1-2"> | |||
<pre> | |||
<div style="background:#FFFACD; border:ridge #FFEC8B; padding:10px"> | |||
Hier steht eine eingebettete Aufgabenstellung. | |||
</div> | |||
</pre> | |||
</div> | |||
<div class="width-1-2"> | |||
<div style="background:#FFFACD; border:ridge #FFEC8B; padding:10px"> | |||
Hier steht eine eingebettete Aufgabenstellung. | |||
</div> | |||
</div> | |||
</div> | |||
==Applets== | |||
===Technischer Benutzungshinweis=== | |||
<div class="grid"> | |||
<div class="width-1-2"> | |||
<pre> | |||
[[Datei:About icon (The Noun Project).svg|15px|middle]] Technischer Benutzungshinweis | |||
</pre> | |||
</div> | |||
<div class="width-1-2"> | |||
[[Datei:About icon (The Noun Project).svg|15px|middle]] Technischer Benutzungshinweis | |||
</div> | |||
</div> | |||
==Mathematik-Modus== | |||
===Äquivalenzumformung=== | |||
<div class="grid"> | |||
<div class="width-1-2"> | |||
<pre> | |||
<math>\begin{align} | |||
& & x^2 + 24 &= 42 - 0 & &\mid \text{Termumformung}\\ | |||
\Leftrightarrow & & x^2 + 24 &= 42 & &\mid -24\\ | |||
\Leftrightarrow & & x^2 &= 18 & &\mid \pm \surd\\ | |||
\Leftrightarrow & & x &= \pm \sqrt{18} | |||
\end{align}</math> | |||
</pre> | |||
</div> | |||
<div class="width-1-2"> | |||
<math>\begin{align} | |||
& & x^2 + 24 &= 42 - 0 & &\mid \text{Termumformung}\\ | |||
\Leftrightarrow & & x^2 + 24 &= 42 & &\mid -24\\ | |||
\Leftrightarrow & & x^2 &= 18 & &\mid \pm \surd\\ | |||
\Leftrightarrow & & x &= \pm \sqrt{18} | |||
\end{align}</math> | |||
</div> | |||
</div> | |||
===Gleichungskette=== | |||
<div class="grid"> | |||
<div class="width-1-2"> | |||
<pre> | |||
<math>\begin{align} | |||
A_{\text{Kreis}} &= \pi \cdot r^2\\ | |||
&= \pi \cdot 42^2\\ | |||
&= \pi \cdot 1.764^2\\ | |||
&\approx 5.541{,}7 | |||
\end{align}</math> | |||
</pre> | |||
</div> | |||
<div class="width-1-2"> | |||
<math>\begin{align} | |||
A_{\text{Kreis}} &= \pi \cdot r^2\\ | |||
&= \pi \cdot 42^2\\ | |||
&= \pi \cdot 1.764^2\\ | |||
&\approx 5.541{,}7 | |||
\end{align}</math> | |||
</div> | |||
</div> | |||
===LGS=== | |||
<div class="grid"> | |||
<div class="width-1-2"> | |||
<pre> | |||
<math>\begin{array}{crcrcr}\\ | |||
\text{I}\quad & 7x & - & 2y & = & 48\\ | |||
\text{II}\quad & 3x & + & 11y & = & 11 | |||
\end{array}</math> | |||
</pre> | |||
</div> | |||
<div class="width-1-2"> | |||
<math>\begin{array}{crcrcr}\\ | |||
\text{I}\quad & 7x & - & 2y & = & 48\\ | |||
\text{II}\quad & 3x & + & 11y & = & 11 | |||
\end{array}</math> | |||
</div> | |||
</div> | |||
<div class="grid"> | |||
<div class="width-1-2"> | |||
<pre> | |||
<math>\left\vert\begin{alignat}{7} | |||
x &&\; + \;&& 42y &&\; - \;&& z &&\; = \;&& 1 \\ | |||
4242x &&\; - \;&& 24y &&\; + \;&& 4z &&\; = \;&& -42\\ | |||
-x &&\; + \;&& \tfrac{1}{3} y &&\; \;&& &&\; = \;&& 0 | |||
\end{alignat}\right\vert</math> | |||
</pre> | |||
</div> | |||
<div class="width-1-2"> | |||
<math>\left\vert\begin{alignat}{7} | |||
x &&\; + \;&& 42y &&\; - \;&& z &&\; = \;&& 1 \\ | |||
4242x &&\; - \;&& 24y &&\; + \;&& 4z &&\; = \;&& -42\\ | |||
-x &&\; + \;&& \tfrac{1}{3} y &&\; \;&& &&\; = \;&& 0 | |||
\end{alignat}\right\vert</math> | |||
</div> | |||
</div> | |||
===Vektoren und Matrizen=== | |||
<div class="grid"> | |||
<div class="width-1-2"> | |||
<pre> | |||
<math>\begin{pmatrix} | |||
1\\ | |||
2\\ | |||
3 | |||
\end{pmatrix}</math> | |||
</pre> | |||
</div> | |||
<div class="width-1-2"> | |||
<math>\begin{pmatrix} | |||
1\\ | |||
2\\ | |||
3 | |||
\end{pmatrix}</math> | |||
</div> | |||
</div> | |||
<div class="grid"> | |||
<div class="width-1-2"> | |||
<pre> | |||
<math>\begin{pmatrix} | |||
1 & 2 & 3\\ | |||
4 & 5 & 6\\ | |||
7 & 8 & 9 | |||
\end{pmatrix}</math> | |||
</pre> | |||
</div> | |||
<div class="width-1-2"> | |||
<math>\begin{pmatrix} | |||
1 & 2 & 3\\ | |||
4 & 5 & 6\\ | |||
7 & 8 & 9 | |||
\end{pmatrix}</math> | |||
</div> | |||
</div> | |||
<div class="grid"> | |||
<div class="width-1-2"> | |||
<pre> | |||
Mit Text in einer Zeile geht <math>\left( \begin{smallmatrix} | |||
1\\ | |||
2\\ | |||
3 | |||
\end{smallmatrix} \right)</math> so und <math>\left( \begin{smallmatrix} | |||
1 & 2 & 3\\ | |||
4 & 5 & 6\\ | |||
7 & 8 & 9 | |||
\end{smallmatrix} \right)</math> so. | |||
</pre> | |||
</div> | |||
<div class="width-1-2"> | |||
Mit Text in einer Zeile geht <math>\left( \begin{smallmatrix} | |||
1\\ | |||
2\\ | |||
3 | |||
\end{smallmatrix} \right)</math> so und <math>\left( \begin{smallmatrix} | |||
1 & 2 & 3\\ | |||
4 & 5 & 6\\ | |||
7 & 8 & 9 | |||
\end{smallmatrix} \right)</math> so. | |||
</div> | |||
</div> | |||
{{SORTIERUNG:{{SUBPAGENAME}}}} | |||
[[Kategorie:Digitale Werkzeuge in der Schule]] | |||
{{Box|Übung 1: Wahr oder falsch? |Entscheide, ob die Aussagen zu den speziellen Winkeln wahr oder falsch sind.|Üben | |||
}} | |||
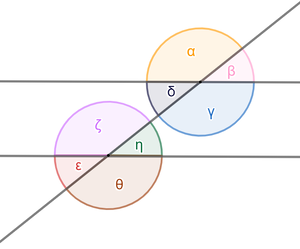
[[Datei:Winkelarten Diagnoseaufgaben.png|mini|ohne|Winkelarten]] | |||
<div class="zuordnungs-quiz"> | |||
{| | |||
|wahr | |||
|Die Winkel α und β sind Nebenwinkel. | |||
|Die Winkel α und γ sind Scheitelwinkel. | |||
|Die Winkel ε und δ sind Stufenwinkel. | |||
|Die Winkel α und θ sind Wechselwinkel. | |||
|Scheitelwinkel sind immer gleich groß. | |||
|- | |||
|falsch | |||
|Wechselwinkel sind zusammen immer 90 Grad groß. | |||
|Die Winkel ζ und δ sind Wechselwinkel. | |||
|Die Winkel α und β sind Scheitelwinkel. | |||
|Die Winkel α und γ sind zusammen 180 Grad groß. | |||
|} | |||
</div> | |||
[[Datei:Winkelarten Diagnoseaufgaben.png|mini|ohne|Winkelarten]] | |||
<quiz display="simple"> | |||
Die Winkel α und γ sind ______________.} | |||
- Wechselwinkel | |||
- Stufenwinkel | |||
- Nebenwinkel | |||
+ Scheitelwinkel | |||
{ Die Winkel ε und δ sind ______________. } | |||
- Wechselwinkel | |||
+ Stufenwinkel | |||
- Nebenwinkel | |||
- Scheitelwinkel | |||
{ Die Winkel α und θ sind ______________. } | |||
+ Wechselwinkel | |||
- Stufenwinkel | |||
- Nebenwinkel | |||
- Scheitelwinkel | |||
{ Die Winkel α und β sind ______________. } | |||
+ zusammen 180 Grad groß. | |||
- Zusammen 90 Grad groß. | |||
</quiz> | |||
Alternativ: welche Aussagen stimmen? | |||
[[Datei:Winkelarten Diagnoseaufgaben.png|mini|ohne|Winkelarten]] | |||
<quiz display="simple"> | |||
Welche der folgenden Aussagen sind wahr?} | |||
+ Die Winkel α und β sind Nebenwinkel. | |||
- Die Winkel α und β sind Scheitelwinkel. | |||
+ Die Winkel ε und δ sind Stufenwinkel. | |||
+ Scheitelwinkel sind immer gleich groß. | |||
- Wechselwinkel sind zusammen immer 90 Grad groß. | |||
- Die Winkel ζ und δ sind Wechselwinkel. | |||
+ Die Winkel α und γ sind Scheitelwinkel. | |||
+ Die Winkel α und θ sind Wechselwinkel. | |||
- Die Winkel α und γ sind zusammen 180 Grad groß. | |||
</quiz> | |||
Aktuelle Version vom 8. November 2024, 11:30 Uhr
Spielwiese
Schreiben im Wiki
Neben normalem Text kann man auch kursiven oder fett gedruckten Text schreiben. Ebenso ist eine Kombination aus beidem möglich. Grüner Text ist schon etwas schwieriger, funktioniert aber über die Quelltextbearbeitung.
Einen neuen Absatz beginnt man in der Quelltextbearbeitung durch zwei aufeinanderfolgende Zeilenumbrüche, also einer leeren Zeile zwischen den beiden Absätzen. In der visuellen Bearbeitung reicht hierzu das einmalige Betätigen der Eingabetaste.

Vorlagen
Dateien
Über die Bedienelemente
Lorem ipsum dolor sit amet, consectetur adipisici elit, sed eiusmod tempor incidunt ut labore et dolore magna aliqua. Ut enim ad minim veniam, quis nostrud exercitation ullamco laboris nisi ut aliquid ex ea commodi consequat. Quis aute iure reprehenderit in voluptate velit esse cillum dolore eu fugiat nulla pariatur. Excepteur sint obcaecat cupiditat non proident, sunt in culpa qui officia deserunt mollit anim id est laborum. Lorem ipsum dolor sit amet, consectetur adipisici elit, sed eiusmod tempor incidunt ut labore et dolore magna aliqua. Ut enim ad minim veniam, quis nostrud exercitation ullamco laboris nisi ut aliquid ex ea commodi consequat. Quis aute iure reprehenderit in voluptate velit esse cillum dolore eu fugiat nulla pariatur. Excepteur sint obcaecat cupiditat non proident, sunt in culpa qui officia deserunt mollit anim id est laborum. Lorem ipsum dolor sit amet, consectetur adipisici elit, sed eiusmod tempor incidunt ut labore et dolore magna aliqua. Ut enim ad minim veniam, quis nostrud exercitation ullamco laboris nisi ut aliquid ex ea commodi consequat. Quis aute iure reprehenderit in voluptate velit esse cillum dolore eu fugiat nulla pariatur. Excepteur sint obcaecat cupiditat non proident, sunt in culpa qui officia deserunt mollit anim id est laborum.
Mittels Quelltexteingabe (Ohne Umfließen des Textes)
Lorem ipsum dolor sit amet, consectetur adipisici elit, sed eiusmod tempor incidunt ut labore et dolore magna aliqua. Ut enim ad minim veniam, quis nostrud exercitation ullamco laboris nisi ut aliquid ex ea commodi consequat. Quis aute iure reprehenderit in voluptate velit esse cillum dolore eu fugiat nulla pariatur. Excepteur sint obcaecat cupiditat non proident, sunt in culpa qui officia deserunt mollit anim id est laborum.
Lorem ipsum dolor sit amet, consectetur adipisici elit, sed eiusmod tempor incidunt ut labore et dolore magna aliqua. Ut enim ad minim veniam, quis nostrud exercitation ullamco laboris nisi ut aliquid ex ea commodi consequat. Quis aute iure reprehenderit in voluptate velit esse cillum dolore eu fugiat nulla pariatur. Excepteur sint obcaecat cupiditat non proident, sunt in culpa qui officia deserunt mollit anim id est laborum.
Über Wikipedia (Ohne Rahmen)
Lorem ipsum dolor sit amet, consectetur adipisici elit, sed eiusmod tempor incidunt ut labore et dolore magna aliqua. Ut enim ad minim veniam, quis nostrud exercitation ullamco laboris nisi ut aliquid ex ea commodi consequat. Quis aute iure reprehenderit in voluptate velit esse cillum dolore eu fugiat nulla pariatur. Excepteur sint obcaecat cupiditat non proident, sunt in culpa qui officia deserunt mollit anim id est laborum.
Lorem ipsum dolor sit amet, consectetur adipisici elit, sed eiusmod tempor incidunt ut labore et dolore magna aliqua. Ut enim ad minim veniam, quis nostrud exercitation ullamco laboris nisi ut aliquid ex ea commodi consequat. Quis aute iure reprehenderit in voluptate velit esse cillum dolore eu fugiat nulla pariatur. Excepteur sint obcaecat cupiditat non proident, sunt in culpa qui officia deserunt mollit anim id est laborum.
Interaktive Applets
LearningApp
GeoGebra

Kombinationen
Die Inhalte basieren auf dem Lernpfad Quadratische Funktionen erkunden von Elena Jedtke.
Auf dieser Seite sind verschiedene Vorlagen für die Lernpfadkapitel des Seminars Digitale Werkzeuge in der Schule dargestellt. Dabei findet sich jeweils links im grau unterlegten Feld der benötigte Code um die Ausgabe rechts daneben zu erhalten.
Allgemeines
Kapitel-Informationskästchen
Bei Lernpfaden für die Sekundarstufe I oder Einführungsphase entfällt der Stichpunkt zu LK-Aufgaben!
{{Box
|1=Info
|2=In diesem Lernpfadkapitel <Kurzbeschreibung des Kapitelziels>
Für die Bearbeitung dieses Kapitels benötigst du den Hefter [https://projekte.zum.de/wiki/Datei:Hefter_zum_Lernpfad_Pyramiden_entdecken.pdf "Pyramiden entdecken"], einen Taschenrechner, einen Stift, einen Bleistift, vier verschiedenfarbige Stifte, einen Zirkel, ein Geodreieck, ein Lineal (mind. 7 cm lang), eine Schere, etwas Klebeband, zwei lose DIN A3-Blätter und zwei lose DIN A4-Blätter.
Bei den Aufgaben unterscheiden wir folgende Typen:
* In Aufgaben, die '''<span style="color: #F19E4F">orange</span>''' gefärbt sind, kannst du '''grundlegende Kompetenzen''' wiederholen und vertiefen.
* Aufgaben in '''<span style="color: #CD2990">pinker</span>''' Farbe sind '''Aufgaben mittlerer Schwierigkeit'''.
* Und Aufgaben mit '''<span style="color: #5E43A5">lilanem</span>''' Streifen sind '''Knobelaufgaben'''.
* Aufgaben, die mit einem ⭐ gekennzeichnet sind, sind nur für den LK gedacht.
Viel Erfolg!
|3=Kurzinfo}}
Parameter vorherlink an aktuellen Lernpfad anpassen.
{{Fortsetzung|vorher=zurück zur Kapitelauswahl|vorherlink=Digitale_Werkzeuge_in_der_Schule/LERNPFAD#Kapitelauswahl}}
Parameter weiterlink an aktuellen Lernpfad anpassen.
{{Fortsetzung|weiter=weiter zum nächsten Kapitel|weiterlink=Digitale_Werkzeuge_in_der_Schule/LERNPFAD#Kapitel}}
{{Lösung versteckt|1=Inhalt|2=Lösung|3=Lösung verbergen}}
Kategoriezuordnung
{{SORTIERUNG:{{SUBPAGENAME}}}}
[[Kategorie:Digitale Werkzeuge in der Schule]]
Siehe Kategorie am Ende dieser Seite.
Aufgaben-Boxen
Schwierigkeitsstufe I
{{Box | Aufgabe <Nummer>: <Name> | Inhalt | Arbeitsmethode | Farbe={{Farbe|orange}} }}
Schwierigkeitsstufe II
{{Box | Aufgabe <Nummer>: <Name> | Inhalt | Arbeitsmethode | Farbe=#CD2990 }}
Schwierigkeitsstufe III
{{Box | Aufgabe <Nummer>: <Name> | Inhalt | Arbeitsmethode}}
Mit Verweis zum Arbeitsblatt
{{Box | Aufgabe <Nummer>: <Name> |
[[Datei:Grundlagen-bearbeiten.png|30px|middle]] '''zurück zum Arbeitsblatt'''
| Arbeitsmethode | Farbe={{Farbe|orange}} }}
LK-Kennzeichnung
Gesamte Aufgabe
{{Box | Aufgabe <Nummer>⭐: <Name> | Inhalt | Arbeitsmethode}}
Teilaufgabe
{{Box | Aufgabe <Nummer>: <Name> |
'''a)''' Inhalt
'''b)''' Inhalt
'''c)⭐''' Inhalt
| Arbeitsmethode}}
Andere Boxen
Merksatz
{{Box | Merksatz: <Name> | Inhalt | Merksatz | Farbe={{Farbe|grün|dunkel}}}}
Beispiel
{{Box | Beispiel: <Name> | Inhalt | Hervorhebung1}}
Eingebettete Aufgabenstellung
<div style="background:#FFFACD; border:ridge #FFEC8B; padding:10px"> Hier steht eine eingebettete Aufgabenstellung. </div>
Hier steht eine eingebettete Aufgabenstellung.
Applets
Technischer Benutzungshinweis
[[Datei:About icon (The Noun Project).svg|15px|middle]] Technischer Benutzungshinweis
Mathematik-Modus
Äquivalenzumformung
<math>\begin{align}
& & x^2 + 24 &= 42 - 0 & &\mid \text{Termumformung}\\
\Leftrightarrow & & x^2 + 24 &= 42 & &\mid -24\\
\Leftrightarrow & & x^2 &= 18 & &\mid \pm \surd\\
\Leftrightarrow & & x &= \pm \sqrt{18}
\end{align}</math>
Gleichungskette
<math>\begin{align}
A_{\text{Kreis}} &= \pi \cdot r^2\\
&= \pi \cdot 42^2\\
&= \pi \cdot 1.764^2\\
&\approx 5.541{,}7
\end{align}</math>
LGS
<math>\begin{array}{crcrcr}\\
\text{I}\quad & 7x & - & 2y & = & 48\\
\text{II}\quad & 3x & + & 11y & = & 11
\end{array}</math>
<math>\left\vert\begin{alignat}{7}
x &&\; + \;&& 42y &&\; - \;&& z &&\; = \;&& 1 \\
4242x &&\; - \;&& 24y &&\; + \;&& 4z &&\; = \;&& -42\\
-x &&\; + \;&& \tfrac{1}{3} y &&\; \;&& &&\; = \;&& 0
\end{alignat}\right\vert</math>
Vektoren und Matrizen
<math>\begin{pmatrix}
1\\
2\\
3
\end{pmatrix}</math>
<math>\begin{pmatrix}
1 & 2 & 3\\
4 & 5 & 6\\
7 & 8 & 9
\end{pmatrix}</math>
Mit Text in einer Zeile geht <math>\left( \begin{smallmatrix}
1\\
2\\
3
\end{smallmatrix} \right)</math> so und <math>\left( \begin{smallmatrix}
1 & 2 & 3\\
4 & 5 & 6\\
7 & 8 & 9
\end{smallmatrix} \right)</math> so.
Mit Text in einer Zeile geht so und so.
| wahr | Die Winkel α und β sind Nebenwinkel. | Die Winkel α und γ sind Scheitelwinkel. | Die Winkel ε und δ sind Stufenwinkel. | Die Winkel α und θ sind Wechselwinkel. | Scheitelwinkel sind immer gleich groß. |
| falsch | Wechselwinkel sind zusammen immer 90 Grad groß. | Die Winkel ζ und δ sind Wechselwinkel. | Die Winkel α und β sind Scheitelwinkel. | Die Winkel α und γ sind zusammen 180 Grad groß. |
Alternativ: welche Aussagen stimmen?