Benutzer:Vivien WWU-6/TestseiteAufgaben: Unterschied zwischen den Versionen
Aus ZUM Projektwiki
Keine Bearbeitungszusammenfassung Markierung: 2017-Quelltext-Bearbeitung |
Keine Bearbeitungszusammenfassung Markierung: 2017-Quelltext-Bearbeitung |
||
| (124 dazwischenliegende Versionen desselben Benutzers werden nicht angezeigt) | |||
| Zeile 1: | Zeile 1: | ||
=== Monotonie === | ===Monotonie=== | ||
{{Box | Merksatz | | {{Box | Merksatz | | ||
Das '''Monotonieverhalten''' einer Funktion | Das '''Monotonieverhalten''' einer Funktion | ||
…beschreibt den Verlauf des Graphen einer Funktion. | …beschreibt den Verlauf des Graphen einer Funktion. Die Montonie gibt an, ob eine Funktion fällt, steigt oder konstant ist. | ||
| Zeile 20: | Zeile 20: | ||
- Falls auf einem Intervall <math>f(x_1) \geq \ f(x_2)</math> gilt, so ist die Funktion '''monoton fallend''' | - Falls auf einem Intervall <math>f(x_1) \geq \ f(x_2)</math> gilt, so ist die Funktion '''monoton fallend''' | ||
[[Datei:MonotonieAbbildung.png|links|1200x1200px]] | |||
| Merke}} | | Merke}} | ||
{{Box | Aufgabe 1 | | {{Box | Aufgabe 1 | | ||
{{LearningApp|width:100%|height:500px|app=p7pny09y220}} | Arbeitsmethode}} | |||
{{Box| So berechnest du das Monotonieverhalten einer Funktion| | |||
1. Erste Ableitung berechnen | |||
2. Nullstellen der ersten Ableitung berechnen | |||
3. Intervalle benennen | |||
4. Monotonietabelle aufstellen | |||
5. Vorzeichen der Intervalle berechnen | |||
6. Ergebnis interpretieren | |||
| Beispiel}} | |||
{{Box| Beispiel: Monotonieverhalten für <math>g(x)=x^2</math> bestimmen | | |||
Zuerst berechnen wir die Ableitung <math>g'(x)=2x</math>. Anschließend berechnen wir die Nullstellen der Ableitung (<math>g'(x)=0</math>) und erhalten durch Umformungen als Nullstelle <math>x=0</math>. | |||
Damit sind die zu betrachtenden Intervalle für das Monotonieverhalten <math>(-\infty,0)</math> und <math>(0,+\infty)</math>. Darauffolgend stellen wir eine Monotonietabelle auf und berechnen die Vorzeichen für die Intervalle: | Beispiel}} | |||
{| class="wikitable center" | |||
|- | |||
! | |||
!<math> -\infty < x < 0 </math> | |||
!<math> f'(0) </math> | |||
!<math> 0 < x < \infty </math> | |||
|- | |||
|<math> f'(x) </math> | |||
|<math> < 0 </math> | |||
|<math> = 0 </math> | |||
|<math> > 0</math> | |||
|- | |||
|Graph von f | |||
|<math> \searrow </math> | |||
|'''Tiefpunkt''' | |||
|<math> \nearrow </math> | |||
|} | |||
Aus dem Ergebnis können wir schließen, dass die Funktion für <math>(-\infty,0)</math> streng monoton fallend und für <math>(0,+\infty)</math> streng monoton steigend ist. | |||
{{Box | Aufgabe 2 | | |||
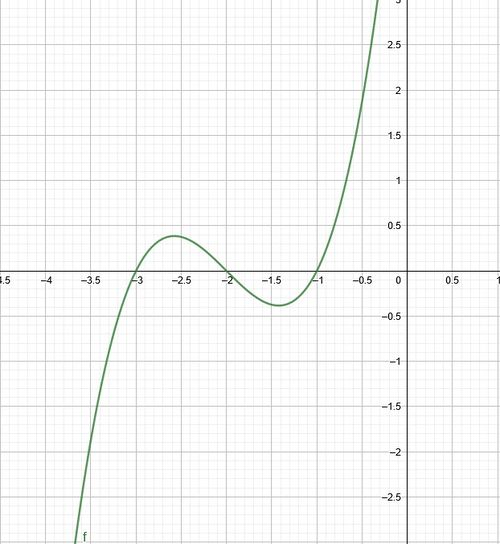
a) Auf dem Bild siehst du den Graphen einer Ableitungsfunktion <math>f'(x)</math>. Welche Aussagen kannst du über das Monotonieverhalten von <math>f(x)</math> machen? | |||
[[Datei:Graph der Funktion f'(x).jpg|links|alternativtext=|544x544px]] | |||
{{Lösung versteckt|1=Erinnere dich daran, wie du bei der Berechnung des Monotonieverhaltens vorgehst. Welche Aussagen zum Monotonieverhalten liefert dir <math>f'(x)=0</math>? |2=Tipp 1|3=Schließen}} | |||
{{Lösung versteckt|1=Die Nullstellen von <math>f'(x)</math> definieren die verschiedenen Intervalle, in denen das Monotonieverhalten von <math>f</math> verschieden ist. Nun kannst du betrachten, auf welchen Intervallen <math>f'(x)</math> <math><0</math> bzw. <math>>0</math> ist. Welche Aussagen kannst du damit über das Monotonieverhalten von <math>f(x)</math> machen? |2=Tipp 2|3=Schließen}} | |||
{{Lösung versteckt|1= Die Nullstellen von <math>f'(x)</math> sind <math>x_1=-3, x_2=-2</math> und <math>x_3=-1</math>. | |||
Damit sind die zu betrachtenden Intervalle <math>(-\infty, -3)</math>, <math>(-3, -2)</math>, <math>(-2, -1)</math> und <math>(-1, +\infty)</math>. Nun kannst du auf den verschiedenen Intervallen anhand des Graphen ablesen, ob <math>f'(x)</math> an diesen <math><0</math> oder <math>>0</math> ist. | |||
Für <math>(-\infty, -3)</math> ist <math>f'(x)<0</math>, somit ist <math>f(x)</math> auf diesem Intervall streng monoton fallend. | |||
Für <math>(-3, -2)</math> ist <math>f'(x)>0</math>, somit ist <math>f(x)</math> auf diesem Intervall streng monoton steigend. | |||
Für <math>(-2, -1)</math> ist <math>f'(x)<0</math>, somit ist <math>f(x)</math> auf diesem Intervall streng monoton fallend. | |||
Für <math>(-1, +\infty)</math> ist <math>f'(x)>0</math>, somit ist <math>f(x)</math> auf diesem Intervall streng monoton steigend. |2=Lösung|3=Schließen}} | |||
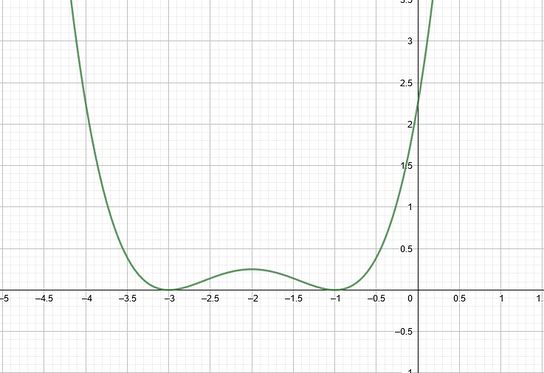
b) Zeichne nun mithilfe deiner Ergebnisse aus a) den Funktionsgraphen <math>f(x)</math> mithilfe deiner Kenntnisse über sein Monotonieverhalten in dein Heft. | |||
{{Lösung versteckt|1=Dein Graph könnte in etwa so aussehen: | |||
[[Datei:Graph f(x).jpg|links|544x544px]] | |||
Möglich, weitere Lösungen für die Zeichnung des Graphen sind unter anderem Verschiebungen in Richtung der Ordinate, also nach unten und oben oder auch Streckungen bzw. Stauchungen. |2=Lösung|3=Schließen}} | Arbeitsmethode}} | |||
{{Box | Aufgabe 3 | | |||
a) Nach einem starken Regenschauer in Münster steigt der Wasserspiegel im Aasee an. Die Funktion <math>f(x)=\frac{1}{4}x^{3} -\frac{25}{2}x^{2} +144x</math> beschreibt die Zuflussgeschwindkeit in den ersten 72 Stunden. Wann fließt innerhalb dieser Zeit Wasser zu und wann Wasser ab? | |||
b) | |||
| Arbeitsmethode}} | |||
Für Aufgabe 2a) Monotonietabelle | |||
{| class="wikitable center" | |||
|- | |||
! | |||
!<math> -\infty < x < 7,4 </math> | |||
!<math> x = 7,4 </math> | |||
!<math> 7,4 < x < 25,92 </math> | |||
!<math> x = 25,92 </math> | |||
!<math> 25,92 < x < \infty </math> | |||
|- | |||
|<math> g'(x) </math> | |||
|<math> > 0 </math> | |||
|<math> = 0 </math> | |||
|<math> < 0</math> | |||
|<math> = 0</math> | |||
|<math> > 0</math> | |||
|- | |||
|Graph von <math>g</math> | |||
|<math> \nearrow </math> | |||
|'''Hochpunkt''' | |||
|<math> \searrow </math> | |||
|'''Tiefpunkt''' | |||
|<math> \nearrow </math> | |||
|} | |||
Für 2b) | |||
{| class="wikitable center" | |||
|- | |||
! | |||
!<math> -\infty < x < -\frac{\sqrt{15}}{5}a </math> | |||
!<math> f'\Big(-\frac{\sqrt{15}}{5}a \Big) </math> | |||
!<math> -\frac{\sqrt{15}}{5}a < x < 0 </math> | |||
!<math> f'\Big(0\Big) </math> | |||
!<math> 0 < x < \frac{\sqrt{15}}{5}a </math> | |||
!<math> f'\Big(\frac{\sqrt{15}}{5}a \Big) </math> | |||
!<math> \frac{\sqrt{15}}{5}a < x < \infty</math> | |||
|- | |||
|<math> f'(x) </math> | |||
|<math> < 0 </math> | |||
|<math> = 0 </math> | |||
|<math> > 0</math> | |||
|<math> = 0</math> | |||
|<math> < 0</math> | |||
|<math> = 0 </math> | |||
|<math> > 0</math> | |||
|- | |||
|<math> Graph von f </math> | |||
|<math> \searrow </math> | |||
|'''TP''' | |||
|<math> \nearrow </math> | |||
|'''HP''' | |||
|<math> \searrow </math> | |||
|'''TP''' | |||
|<math> \nearrow </math> | |||
|} | |||
{| class="wikitable center" | |||
|- | |||
! | |||
!<math> x=0 </math> | |||
!<math> 0 < x < \frac{\sqrt{15}}{5}a </math> | |||
!<math> x=\frac{\sqrt{15}}{5}a </math> | |||
!<math> \frac{\sqrt{15}}{5}a < x \leq \ 4</math> | |||
|- | |||
|<math> f_a'(x) </math> | |||
|<math> = 0</math> | |||
|<math> < 0</math> | |||
|<math> = 0 </math> | |||
|<math> > 0</math> | |||
|- | |||
| Graph von <math>f_a</math> | |||
|'''HP''' | |||
|<math> \searrow </math> | |||
|'''TP''' | |||
|<math> \nearrow </math> | |||
|} | |||
{| class="wikitable center" | |||
|- | |||
! | |||
!<math> -\infty < x < -\frac{1}{3} </math> | |||
!<math> f'\Big(-\frac{1}{3}\Big) </math> | |||
!<math> -\frac{1}{3} < x < 1 </math> | |||
!<math> f'(1) </math> | |||
!<math> 1 < x < \infty </math> | |||
|- | |||
|<math> f'(x) </math> | |||
|<math> > 0 </math> | |||
|<math> = 0 </math> | |||
|<math> < 0</math> | |||
|<math> = 0</math> | |||
|<math> > 0</math> | |||
|- | |||
|<math> G_{f} </math> | |||
|<math> \nearrow </math> | |||
|'''Hochpunkt''' | |||
|<math> \searrow </math> | |||
|'''Tiefpunkt''' | |||
|<math> \nearrow </math> | |||
|} | |||
{| class="wikitable center" | |||
|- | |||
! | |||
!<math> -\infty < x < 0 </math> | |||
!<math> x=0 </math> | |||
!<math> 0 < x < \infty </math> | |||
|- | |||
|<math> f'(x) </math> | |||
|<math> < 0 </math> | |||
|<math> = 0 </math> | |||
|<math> > 0</math> | |||
|- | |||
|Graph von <math> f </math> | |||
|<math> \searrow </math> | |||
|'''Tiefpunkt''' | |||
|<math> \nearrow </math> | |||
|} | |||
Aktuelle Version vom 18. Mai 2020, 10:09 Uhr
Monotonie
| Graph von f | Tiefpunkt |
Aus dem Ergebnis können wir schließen, dass die Funktion für streng monoton fallend und für streng monoton steigend ist.
Für Aufgabe 2a) Monotonietabelle
| Graph von | Hochpunkt | Tiefpunkt |
Für 2b)
| TP | HP | TP |
| Graph von | HP | TP |
| Hochpunkt | Tiefpunkt |
| Graph von | Tiefpunkt |