Herta-Lebenstein-Realschule/Die Scheitelpunktform quadratischer Funktionen sportlich erarbeiten: Unterschied zwischen den Versionen

Aus ZUM Projektwiki
Keine Bearbeitungszusammenfassung
Markierung: 2017-Quelltext-Bearbeitung
Keine Bearbeitungszusammenfassung
Markierung: 2017-Quelltext-Bearbeitung
Zeile 44: Zeile 44:


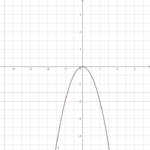
| style="padding:5px" |[[Datei:F(x) = -2x².png|150px]]
| style="padding:5px" |[[Datei:F(x) = -2x².png|150px]]
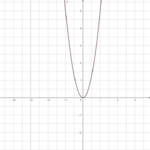
| style="padding:5px" |[[Datei:F(x) = 5x².png|150px]]
| style="padding:5px" |[[Datei:F(x) = 0.2x².png|150px]]


|-  
|-  


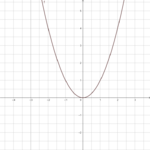
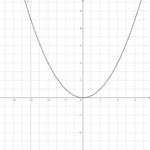
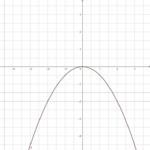
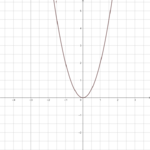
|<strong>f(x) = x<sup>2</sup> </strong> ||<strong>f(x) = - x<sup>2</sup> </strong>||<strong>f(x) = 0,5x<sup>2</sup></strong>||<strong>f(x) = -0,5x<sup>2</sup></strong>||<strong>f(x) = 2x<sup>2</sup> </strong>||<strong>f(x) = -2x<sup>2</sup></strong>
|<strong>f(x) = x<sup>2</sup> </strong> ||<strong>f(x) = - x<sup>2</sup> </strong>||<strong>f(x) = 0,5x<sup>2</sup></strong>||<strong>f(x) = -0,5x<sup>2</sup></strong>||<strong>f(x) = 2x<sup>2</sup> </strong>||<strong>f(x) = -2x<sup>2</sup></strong>
||<strong>f(x) = 5x<sup>2</sup></strong>||<strong>f(x) = <math>{1 \over 5}</math>x<sup>2</sup></strong>


|}
|}

Version vom 10. April 2020, 10:14 Uhr

Lernpfad Scheitelpunktform quadratische Funktionen sportlich erarbeiten
[[Bild:
Bild von Hebi B. auf Pixabay
|250px]]
 Die Parameter a, d und e der Scheitelpunktform quadratische Funktionen f(x) = a (x + d)² + e werden mithilfe dreier "Sportler" erarbeiten

  1. Anton: f(x) = ax²

Anton ist sehr sportlich, er spielt Basketball:

  • normal

    normal

  • gestreckt

    gestreckt

  • gestaucht

    gestaucht


Bedeutung des Parameters a
Welche Rolle spielt anton für den Graphen der Parabel?


Öffne die Seite und verändere a.

GeoGebra


Welche Auswirkungen hat der anton auf das Schaubild der Normalparabel?



Bedeutung des Parameters a
Schreibe den Lückentext in dein Heft ab.


Wende dein Wissen an.
Ordne den Funktionsgraphen die passenden Funktionsgleichungen zu.
F(x) = x².png F(x) = -x².png F(x) = 0.5x².png F(x) = -0.5x².png F(x) = 2x².png F(x) = -2x².png F(x) = 5x².png F(x) = 0.2x².png
f(x) = x2   f(x) = - x2 f(x) = 0,5x2 f(x) = -0,5x2 f(x) = 2x2 f(x) = -2x2 f(x) = 5x2 f(x) = x2




2. Detlef: f(x) = (x + d)²

Detlef ist ebenfalls sportlich, allerdings auch ein wenig dusselig. Er läuft beim Sprint immer in die entgegengesetzte Richtung.


  • Bild von OpenClipart-Vectors auf Pixabay

    Bild von OpenClipart-Vectors auf Pixabay

  • Jumping-151842 1280.png
  • Jumping-151842 1280.png
  • Detlef

    Detlef


Bedeutung des Parameters d
Welche Rolle spielt detlef ?

Öffne die Seite und verändere d.

GeoGebra

Welche Auswirkungen hat detlf auf das Schaubild der Normalparabel?



Bedeutung des Parameters d
Schreibe den ausgefüllten Lückentext zur Bedeutung des Parameters d für in dein Heft ab.


Wende dein Wissen an
Ordne den Funktionsgraphen die passenden Funktionsgleichungen zu.

3. Emil: f(x) = x² + e

emil ist ebenfalls sehr sportlich:

Er kann sehr hoch springen, ebenso gut kann er tauchen.

Bedeutung des Parameters e
Welche Rolle spielt emil ?
Öffne die Seite und verändere e.
GeoGebra



Welche Auswirkungen hat emil auf das Schaubild der Normalparabel?


Bedeutung des Parameters e
Schreibe den ausgefüllten Lückentext zur Bedeutung des Parameters e für in dein Heft ab.


Wende dein Wissen an
Ordne den Funktionsgraphen die passenden Funktionsgleichungen zu.




Abgerufen von „https://projekte.zum.de/index.php?title=Herta-Lebenstein-Realschule/Die_Scheitelpunktform_quadratischer_Funktionen_sportlich_erarbeiten&oldid=17588“